Question

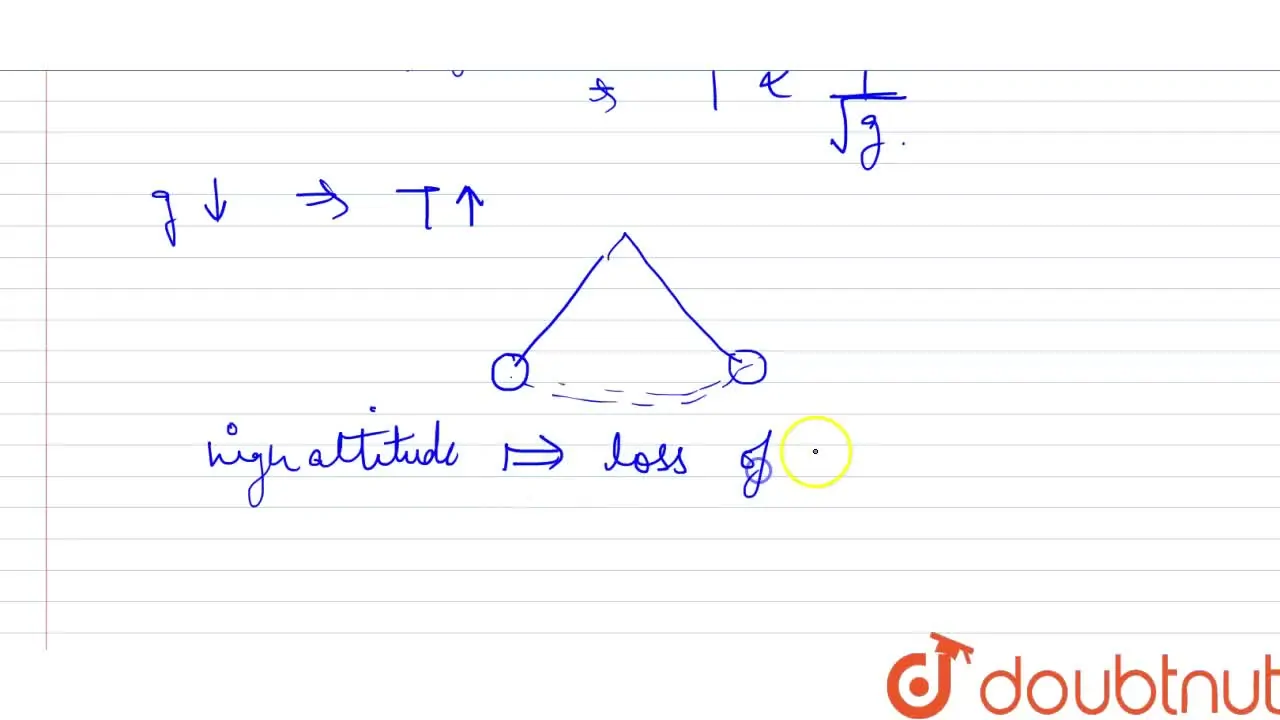
A pendulum clock is taken to a high altitude. Will it gain or lose time?

Explanation

To determine whether a pendulum clock will gain or lose time when taken to a high altitude, we can analyze the effect of altitude on the gravitational acceleration (g) and the time period of the pendulum.

1. Understanding the Effect of Altitude on Gravitational Acceleration (g):
- As we ascend to higher altitudes, the gravitational acceleration (g) decreases. This is due to the fact that gravitational force diminishes with distance from the Earth's center.

2. Time Period of a Pendulum:
- The time period (T) of a simple pendulum is given by the formula:
\( T = 2\pi \sqrt{\frac{L}{g}} \)
where \(L\) is the length of the pendulum and \(g\) is the acceleration due to gravity.

3. Analyzing the Relationship:
- From the formula, we can see that the time period \(T\) is inversely proportional to the square root of \(g\). This means that as \(g\) decreases, the time period \(T\) increases.

4. Conclusion on Time Measurement:
- Since the time period of the pendulum increases at higher altitudes (due to the decrease in g), the pendulum will take longer to complete one oscillation. Consequently, the clock will take longer to measure the same amount of time compared to when it is at sea level.

5. Final Result:
- Therefore, when the pendulum clock is taken to a high altitude, it will lose time because it takes longer to complete each oscillation.

Summary:
At high altitudes, the pendulum clock will lose time due to the decrease in gravitational acceleration, which increases the time period of the pendulum's oscillation.