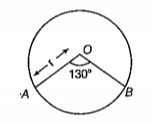
In the adjoining circle C(O,r)the degree measure of minor arc $A B = 130^{\circ}$ Find the degree measure of major arc.

A

$230^{\circ}$

B

$260^{\circ}$

C

$310^{\circ}$

D

none of the above

Video Solution

Text Solution

Verified by Experts

The correct Answer is:A

|

Answer

Step by step video, text & image solution for In the adjoining circle C(O,r)the degree measure of minor arc AB=130^@ Find the degree measure of major arc. by Maths experts to help you in doubts & scoring excellent marks in Class 14 exams.

Updated on:21/07/2023

Class 14 MATHS GEOMETRY

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
In the adjoining circle C(O,r)the degree measure of minor arc $A B = 130^{\circ}$ Find the degree measure of major arc.

A $230^{\circ}$
B $260^{\circ}$
C $310^{\circ}$
Dnone of the above
Question 2 - Select One
Area of a sector of a circle is $\frac{1}{6}$ of the circle. Find the degree measure of it minor arc.
A $90^{\circ}$
B $60^{\circ}$
C $45^{\circ}$
D $30^{\circ}$
Question 3 - Select One
If the measure of an arc of a circle is $60^{\circ}$ , then what is the measure of its corresponding arc ?
A $60^{\circ}$
B $120^{\circ}$
C $30^{\circ}$
D $300^{\circ}$

ARIHANT SSC-GEOMETRY-INTRODUCTORY EXERCISE - 12.5

Home
Ask Doubt
Profile