Write the conditions for total internal reflection.
To determine the conditions for total internal reflection, we need to consider the behavior of light as it travels between two different media. Here’s a step-by-step solution outlining the conditions:
Step 1: Identify the Media
- Total internal reflection occurs at the interface between two media. The first medium (Medium 1) must be denser than the second medium (Medium 2).
Step 2: Direction of Light
- Light must be traveling from the denser medium (Medium 1) to the rarer medium (Medium 2). This means that the refractive index of Medium 1 should be greater than that of Medium 2.
Step 3: Angle of Incidence
- The angle of incidence (the angle at which the light hits the boundary between the two media) must be greater than the critical angle. The critical angle is defined as the angle of incidence that results in an angle of refraction of 90 degrees, meaning the light travels along the boundary.
Step 4: Summary of Conditions
- Therefore, the two conditions for total internal reflection are:
1. Light must travel from a denser medium to a rarer medium.
2. The angle of incidence must be greater than the critical angle.
- Total internal reflection occurs at the interface between two media. The first medium (Medium 1) must be denser than the second medium (Medium 2).
- Light must be traveling from the denser medium (Medium 1) to the rarer medium (Medium 2). This means that the refractive index of Medium 1 should be greater than that of Medium 2.
- The angle of incidence (the angle at which the light hits the boundary between the two media) must be greater than the critical angle. The critical angle is defined as the angle of incidence that results in an angle of refraction of 90 degrees, meaning the light travels along the boundary.
- Therefore, the two conditions for total internal reflection are:
1. Light must travel from a denser medium to a rarer medium.
2. The angle of incidence must be greater than the critical angle.
Topper's Solved these Questions
- OPTICSBOOK - MBD -HARYANA BOARDCHAPTER - OPTICSEXERCISE - SHORT ANSWER TYPE QUESTIONS
46 Videos
- OPTICSBOOK - MBD -HARYANA BOARDCHAPTER - OPTICSEXERCISE - LONG ANSWER TYPE QUESTIONS
18 Videos
- MODEL TEST PAPER - 2BOOK - MBD -HARYANA BOARDCHAPTER - MODEL TEST PAPER - 2EXERCISE - SET - D ( LONG ANSWER TYPE QUESTIONS)
4 Videos
- PRINCIPLES OF COMMUNICATIONBOOK - MBD -HARYANA BOARDCHAPTER - PRINCIPLES OF COMMUNICATIONEXERCISE - Objective Type Questions
19 Videos
Similar Questions
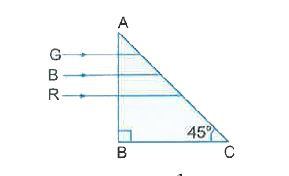
State the conditions of total internal reflection. Refractive indices of the given prism material for Red, Blue and Green colors are respectively 1.39, 1.48 and 1.42 respectively. Trace the path of rays through the prism.
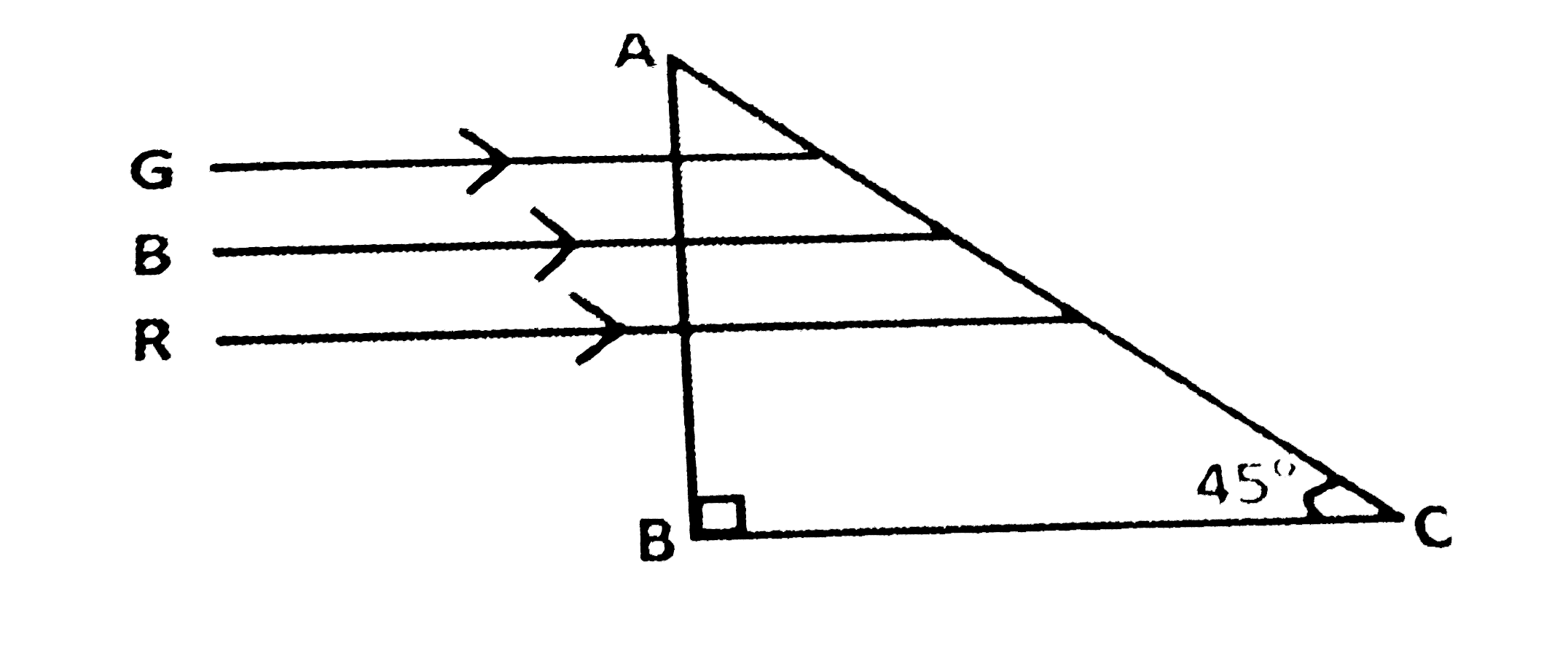
View SolutionState the conditions of total internal reflection. Refractive indices of the given prism material for Red, Blue and Green colours are respectively 1.39, 1.48 and 1.42 respectively. Trace the path of rays through the prism.
View Solution
Knowledge Check
For a given incident ray as shown in Fig., the condition of total internal reflection of ray will be satisfied if the refractive index of the block will be.
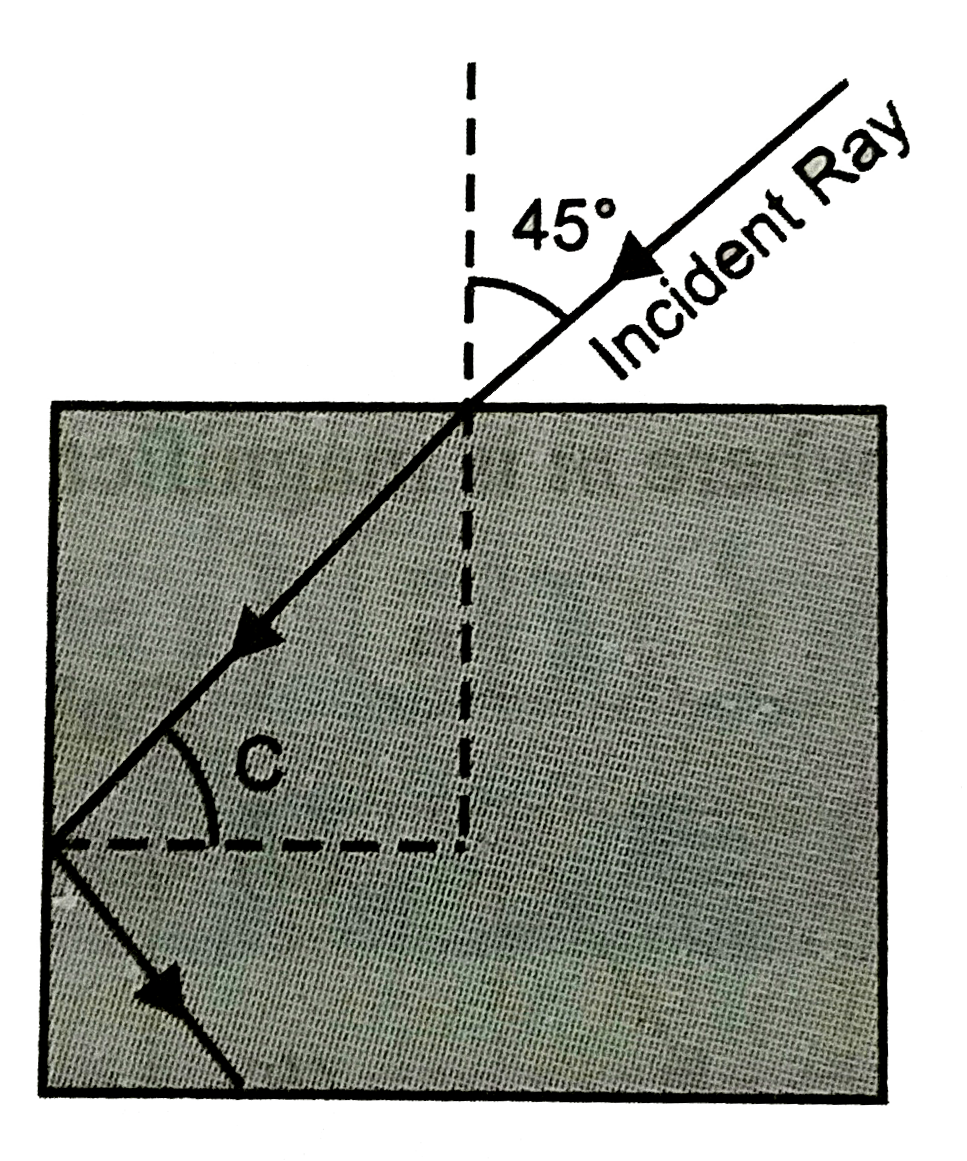 .A√3+12B√2+12C√32D√76
.A√3+12B√2+12C√32D√76If the critical angle for total internal reflection from a medium to vacuum is 30∘, the velocity of light in the medium is
A3×108 m/sB1.5×108 m/sC6×108 m/sD√3×108 m/sIf the critical angle for total internal reflection, from a medium to vacuum is 30∘, then velocity of light in the medium is
A6×108m/secB3×108m/secC2×108m/secD1.5×108m/sec
What is total internal reflection of light ? What are conditions for total internal reflection ?
View SolutionConditions Of Total Internal Reflection
View SolutionWhat is total internalreflection ? What is the meaning of critical angle ? What are the necessary conditions for total internal reflection ?
Write the relation between critical angle and refractive index of the medium.
View SolutionDescribe briefly any three applications of total internal reflection.
View SolutionWhat is critical angle? Give one application of total internal reflection.
View Solution
