A parallelogram has sides 30 cm and 20 cm and one of its diagonal is 40 cm long. Then its area is :
75√5cm2
245cm2
150√15cm2
300cm2
The correct Answer is:C
To find the area of the parallelogram given its sides and one diagonal, we can use Heron's formula. Here’s a step-by-step solution:
Step 1: Identify the given values
We have a parallelogram with:
- Side a=30 cm
- Side b=20 cm
- Diagonal d=40 cm
Step 2: Split the parallelogram into two triangles
The diagonal divides the parallelogram into two triangles, △ABC and △ADC. Both triangles share the diagonal AC.
Step 3: Calculate the semi-perimeter of triangle ABC
The semi-perimeter s of triangle ABC is calculated as follows:
s=a+b+d2=30+20+402=902=45 cm
Step 4: Use Heron's formula to find the area of triangle ABC
Heron's formula states that the area A of a triangle can be calculated using:
A=√s(s−a)(s−b)(s−d)
Substituting the values we have:
A=√45(45−30)(45−20)(45−40)
Calculating each term:
- s−a=45−30=15
- s−b=45−20=25
- s−d=45−40=5
Now substituting these values into the formula:
A=√45×15×25×5
Step 5: Simplify the expression
Calculating the product inside the square root:
45=32×5,15=3×5,25=52,5=5
Thus,
A=√(32×5)×(3×5)×(52)×(5)=√33×55
Step 6: Calculate the area of triangle ABC
Taking the square root:
A=33/2×55/2=3√3×25√5=75√15 cm2
Step 7: Calculate the area of the parallelogram
Since the area of the parallelogram is twice the area of one triangle:
Area of parallelogram=2×A=2×75√15=150√15 cm2
Final Answer
The area of the parallelogram is 150√15 cm2.
---
We have a parallelogram with:
- Side a=30 cm
- Side b=20 cm
- Diagonal d=40 cm
The diagonal divides the parallelogram into two triangles, △ABC and △ADC. Both triangles share the diagonal AC.
The semi-perimeter s of triangle ABC is calculated as follows:
s=a+b+d2=30+20+402=902=45 cm
Heron's formula states that the area A of a triangle can be calculated using:
A=√s(s−a)(s−b)(s−d)
Substituting the values we have:
A=√45(45−30)(45−20)(45−40)
Calculating each term:
- s−a=45−30=15
- s−b=45−20=25
- s−d=45−40=5
A=√45×15×25×5
Calculating the product inside the square root:
45=32×5,15=3×5,25=52,5=5
Thus,
A=√(32×5)×(3×5)×(52)×(5)=√33×55
Taking the square root:
A=33/2×55/2=3√3×25√5=75√15 cm2
Since the area of the parallelogram is twice the area of one triangle:
Area of parallelogram=2×A=2×75√15=150√15 cm2
The area of the parallelogram is 150√15 cm2.
Topper's Solved these Questions
- MENSURATIONBOOK - ARIHANT SSCCHAPTER - MENSURATIONEXERCISE - INTRODUCTORY EXERCISE- 10.4
25 Videos
- MENSURATIONBOOK - ARIHANT SSCCHAPTER - MENSURATIONEXERCISE - INTRODUCTORY EXERCISE- 10.5
32 Videos
- MENSURATIONBOOK - ARIHANT SSCCHAPTER - MENSURATIONEXERCISE - INTRODUCTORY EXERCISE- 10.2
10 Videos
- LOGARITHM BOOK - ARIHANT SSCCHAPTER - LOGARITHM EXERCISE - EXERCISE LEVEL 2
19 Videos
- MIXED GRAPHBOOK - ARIHANT SSCCHAPTER - MIXED GRAPHEXERCISE - Higher Skill Level Questions
15 Videos
Similar Questions
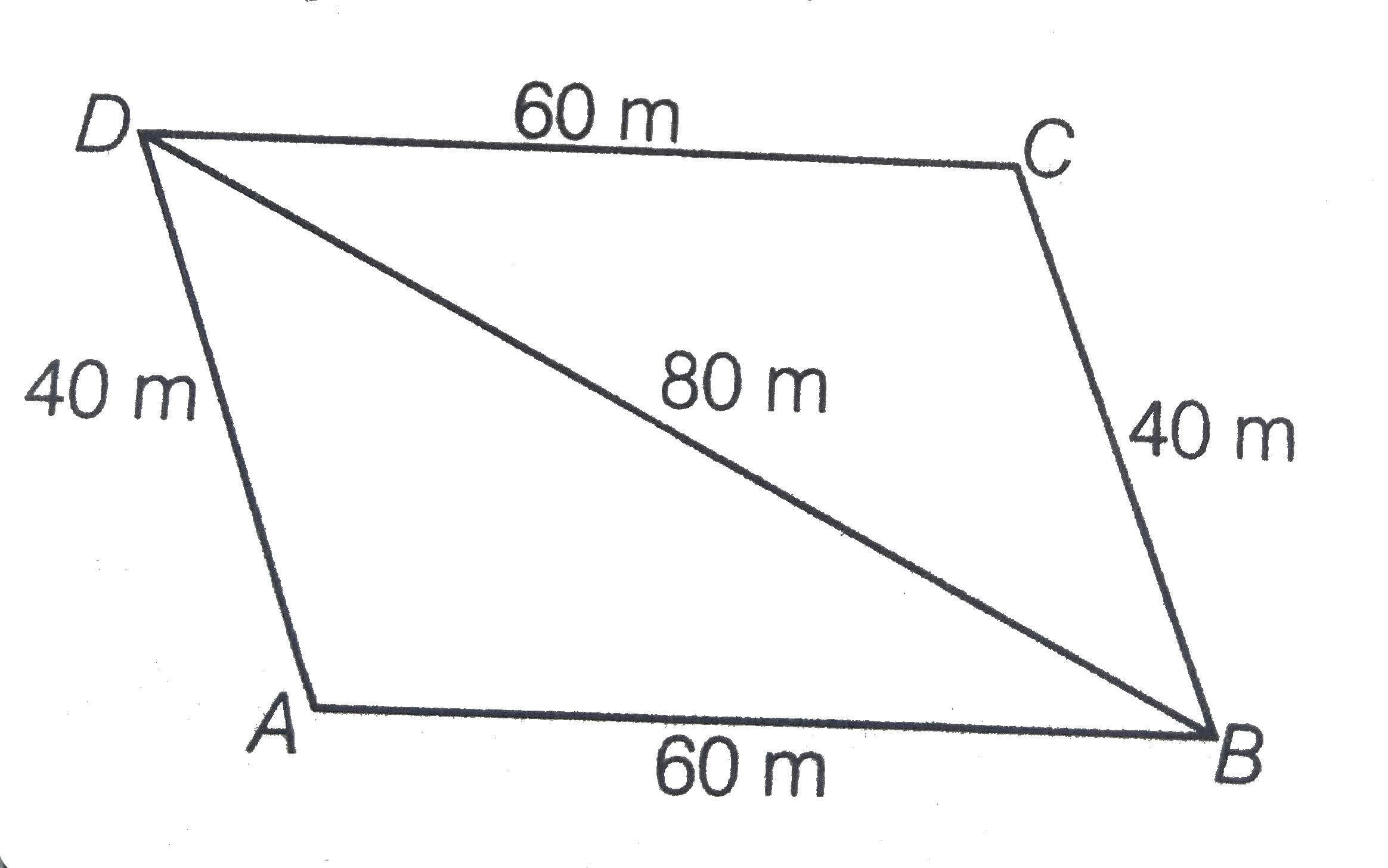
A field in the form of a parallelogram has sides 60 m and 40 m and one of its diagonals is 80 m long. Find the area of the parallelogram.
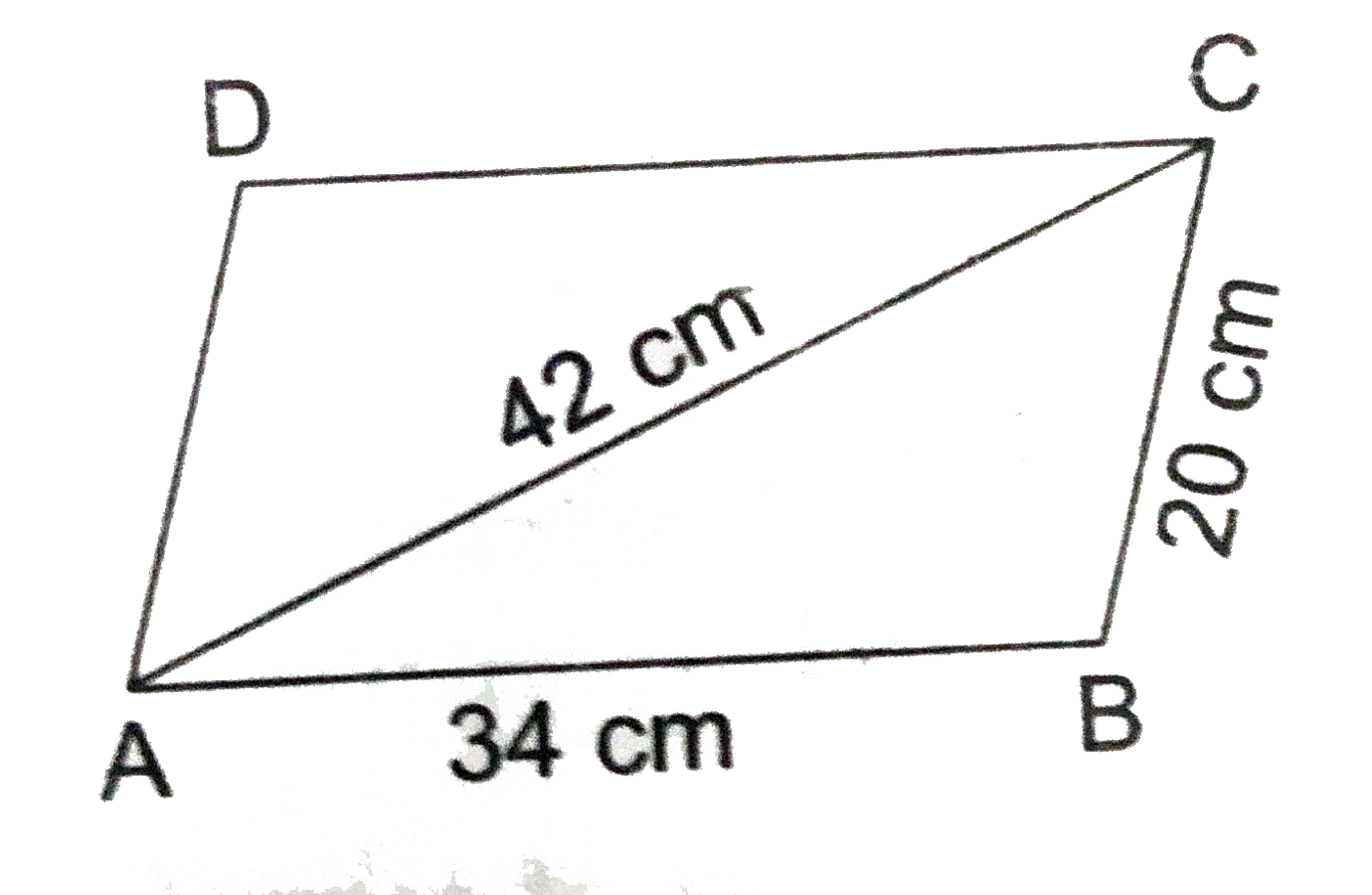
View SolutionThe adjacent sides of a parallelogram ABCD measure 34 cm and 20 cm , and the diagonal ac measures 42 cm . Find the area of the parallelogram
View Solution
Knowledge Check
A parallelogram has sides 30 cm and 20 cm and one of its diagonal is 40 cm long. Then its area is :
A75√5cm2B245cm2C150√15cm2D300cm2A field in the form of a parallelogram has sides 60 m and 40 m and one of its diagonals is 80 m long. Find the area of the parallelogram.
 A480 sq. mB320 sq. mC600√15sq.mD450√15 sq . M
A480 sq. mB320 sq. mC600√15sq.mD450√15 sq . MA parallelogram has sides 30 m, 70 m and one of its diagonals is 80 m long. Its area will be
A600 m2B1200√3m2C1200 m2D600√3m2
A parallelogram has sides 15 cm and 7 cm long. The length of one of the diagonals is 20 cm. The area of the parallelogram is
View SolutionThe sides of a parallelogram are 12 cm and 8 cm long and one of the diagonals is 10 cm long. If d is the length of other diagonal, then which one of the following is correct?
View SolutionTwo adjacent sides of a parallelogram are 10 cm and 12 cm. If its one diagonal is 14 cm long, find the area of the parallelogram.
View SolutionThe side of a rhombus are 10 cm and one of its diagonals is 16 cm . The area of the rhombus is :
View SolutionThe side of a rhombus are 10 cm and one of its diagonals is 16 cm . The area of the rhombus is :
View Solution
