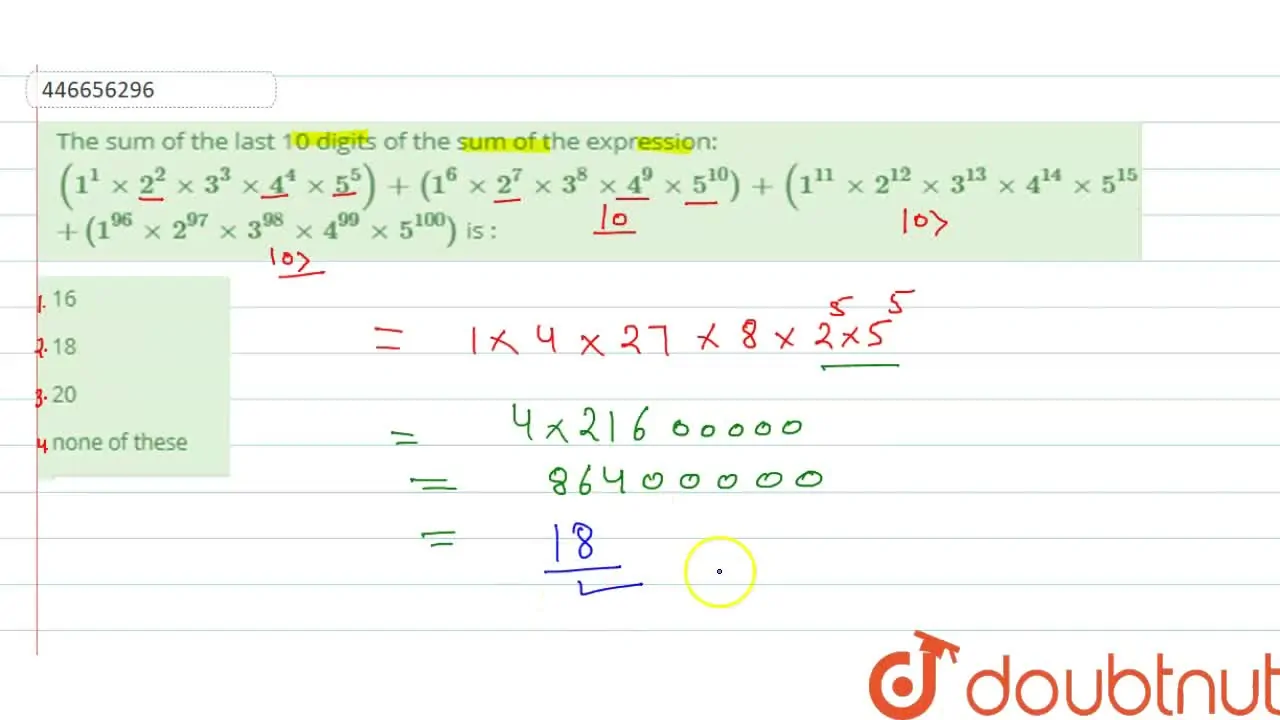
The sum of the last 10 digits of the sum of the expression:
(11×22×33×44×55)+(16×27×38×49×510)+(111×212×313×414×515)+……
+(196×297×398×499×5100) is :
16
18
20
none of these
The correct Answer is:B
Step by step video, text & image solution for The sum of the last 10 digits of the sum of the expression: (1^1 xx 2^2 xx 3^3 xx 4^4 xx 5^5) + (1^6 xx 2^7 xx 3^8 xx 4^9 xx 5^10) + (1^11 xx 2^12 xx 3^13 xx 4^14 xx 5^15) + …… +(1^96 xx 2^97 xx 3^(98) xx 4^(99) xx 5^(100)) is : by Maths experts to help you in doubts & scoring excellent marks in Class 14 exams.
Related Playlists
- BOOK - ARIHANT SSCCHAPTER - FUNDAMENTALS EXERCISE - TEST OF YOU - LEARNING - 140videos
FUNDAMENTALS
- BOOK - ARIHANT SSCCHAPTER - FUNDAMENTALS EXERCISE - TEST OF YOU - LEARNING - 240videos
FUNDAMENTALS
- BOOK - ARIHANT SSCCHAPTER - FUNDAMENTALS EXERCISE - LEVEL 2123videos
FUNDAMENTALS
- BOOK - ARIHANT SSCCHAPTER - FUNCTIONS AND GRAPHEXERCISE - Final Round40videos
FUNCTIONS AND GRAPH
- BOOK - ARIHANT SSCCHAPTER - GEOMETRYEXERCISE - EXERCISE(LEVEL 2)52videos
GEOMETRY
Similar Questions
Find the number of Zeros at the end of the product -
21×52×23×54×25×56×27×58×29×510View SolutionThe last digit of the expression
4×92×43×94×45×96×…..×499×9100 is:View SolutionThe unit digit of expression
1×2×3×4×5×6×7×8×9×10100.View SolutionFind the number of Zeros at the end of the product -
21×52×23×54×25×56×27×58×29×510View SolutionNumber of zeros in the end of (11×22×33×44×.......×9898×9999×100100)?
View SolutionThe last digit of the expression
4+92+43+94+45+96+…..+499+9100 is:View SolutionThe number of zeros at the end of the product of :
23×34×45×56+35×57×79×810+45×56×67×78−102×153×204 is :View SolutionFind the LCM of (23×3×52×7),(24×32×5×72×1) and (2×33×54).
View SolutionFind the LCM of (25×3×52×7)(24×33×5×72×11)and(2×35×54)
View SolutionWhat is the value of (1×2+2×3−3×4+4×5−5×6+6×7)?
View Solution