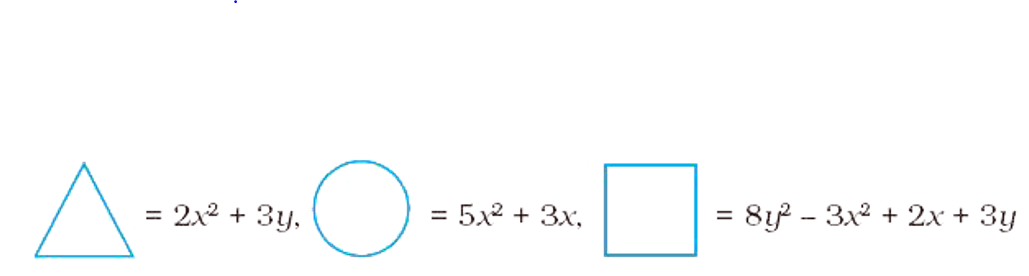When (55)10 represented in base 25 then the expression is
(25)25
(35)25
(55)25
none of these
The correct Answer is:A
To convert the decimal number (55)10 into base 25, we will follow these steps:
Step 1: Divide the number by the base
We start by dividing 55 by 25.
55÷25=2(quotient)
55−(25×2)=5(remainder)
Step 2: Record the remainder
The remainder from the division is 5.
Step 3: Divide the quotient by the base
Next, we take the quotient 2 and divide it by 25.
2÷25=0(quotient)
2−(25×0)=2(remainder)
Step 4: Record the remainder
The remainder from this division is 2.
Step 5: Write the base 25 representation
Now, we have the remainders from the divisions: the first remainder (from the first division) is 5 and the second remainder (from the second division) is 2.
To write the number in base 25, we read the remainders in reverse order:
(25)25=(25)10 is represented as (25)25=25
Thus, the representation of (55)10 in base 25 is (25)25.
Final Answer
The expression of (55)10 in base 25 is (25)25.
---
We start by dividing 55 by 25.
55−(25×2)=5(remainder)
The remainder from the division is 5.
Next, we take the quotient 2 and divide it by 25.
2−(25×0)=2(remainder)
The remainder from this division is 2.
Now, we have the remainders from the divisions: the first remainder (from the first division) is 5 and the second remainder (from the second division) is 2.
The expression of (55)10 in base 25 is (25)25.
Topper's Solved these Questions
- FUNDAMENTALS Book:ARIHANT SSCChapter:FUNDAMENTALS Exercise:FINAL ROUND
Explore 116 Videos
- FUNDAMENTALS Book:ARIHANT SSCChapter:FUNDAMENTALS Exercise:TEST OF YOU - LEARNING - 1
Explore 40 Videos
- FUNDAMENTALS Book:ARIHANT SSCChapter:FUNDAMENTALS Exercise:LEVEL 1
Explore 140 Videos
- FUNCTIONS AND GRAPHBook:ARIHANT SSCChapter:FUNCTIONS AND GRAPHExercise:Final Round
Explore 40 Videos
- GEOMETRYBook:ARIHANT SSCChapter:GEOMETRYExercise:EXERCISE(LEVEL 2)
Explore 52 Videos
Similar Questions
Knowledge Check
When (55)10 represented in base 25 then the expression is
A(25)25B(35)25C(55)25Dnone of theseA wave is represented by the equation y=Asin(10πx+15πl+π6) wher x is in metre and t in second. The expression represents
AA wave travelling in negative x - direction with a velocity of 1.5 m/sBA wave travelling in positive x direction with a velocity of 1.5 m/sCA wave travelling in position x - direction with wavelength 0.2 mDA wave travelling in negative x-direction with a velocity of 150 m/sThe osciallations represented by curve 1 in the graph are expressed by equation x=Asinεt. The equation for the oscillations represented by curve 2 is expressed as
 Ax=2Asin(ωt−π/2)Bx=2Asing(ωt+π/2)Cx=−2Asin(ωt−π/2)Dnone of these
Ax=2Asin(ωt−π/2)Bx=2Asing(ωt+π/2)Cx=−2Asin(ωt−π/2)Dnone of these
Illustrations based on range OF expressions and graphs
View SolutionIf the equations 4x+7y=10 and 10x+ky=25 represent coincident lines then find the value of k?
View Solution
The oscillations represented by curve 1 in the graph are expressed by equationx=Asinωt. The equation for the oscillation represented by curve 2 is expressed as
View SolutionA wave is represented by the equation
y=Asin(10πx+15πt+π3)
where x is in meter and t is in seconds. The expression represents :
View SolutionIf the equations 4x+7y=10 and 10x+ky=25 represent coincident lines then find the value of k?
View Solution