The largest possible length of a tape which can measure 525 cm, 1050 cm and 1155 cm length of cloths in a minium number of attempts without measuring the length of a cloth in a fraction of the tape's length
25
105
75
None of these
The correct Answer is:B
To find the largest possible length of a tape that can measure 525 cm, 1050 cm, and 1155 cm in a minimum number of attempts without measuring in fractions, we need to determine the highest common factor (HCF) of these three lengths. Here’s how to solve the problem step by step:
Step 1: Prime Factorization of Each Length
We will start by finding the prime factorization of each of the three lengths.
1. For 525:
- Divide by 5: 525÷5=105
- Divide 105 by 5: 105÷5=21
- Divide 21 by 3: 21÷3=7
- 7 is a prime number.
- Therefore, the prime factorization of 525 is:
525=52×31×71
2. For 1050:
- Divide by 5: 1050÷5=210
- Divide 210 by 5: 210÷5=42
- Divide 42 by 2: 42÷2=21
- Divide 21 by 3: 21÷3=7
- 7 is a prime number.
- Therefore, the prime factorization of 1050 is:
1050=52×21×31×71
3. For 1155:
- Divide by 5: 1155÷5=231
- Divide 231 by 3: 231÷3=77
- Divide 77 by 7: 77÷7=11
- 11 is a prime number.
- Therefore, the prime factorization of 1155 is:
1155=51×31×71×111
Step 2: Identify Common Factors
Next, we identify the common prime factors from the factorizations:
- Common factors:
- 5 appears in all three factorizations.
- 3 appears in all three factorizations.
- 7 appears in all three factorizations.
Step 3: Calculate the HCF
To find the HCF, we take the lowest power of each common prime factor:
- For 5: The lowest power is 51.
- For 3: The lowest power is 31.
- For 7: The lowest power is 71.
Now, we multiply these together to find the HCF:
HCF=51×31×71=5×3×7=105
Conclusion
The largest possible length of the tape that can measure 525 cm, 1050 cm, and 1155 cm is 105 cm.
We will start by finding the prime factorization of each of the three lengths.
- Divide by 5: 525÷5=105
- Divide 105 by 5: 105÷5=21
- Divide 21 by 3: 21÷3=7
- 7 is a prime number.
- Therefore, the prime factorization of 525 is:
525=52×31×71
- Divide by 5: 1050÷5=210
- Divide 210 by 5: 210÷5=42
- Divide 42 by 2: 42÷2=21
- Divide 21 by 3: 21÷3=7
- 7 is a prime number.
- Therefore, the prime factorization of 1050 is:
1050=52×21×31×71
- Divide by 5: 1155÷5=231
- Divide 231 by 3: 231÷3=77
- Divide 77 by 7: 77÷7=11
- 11 is a prime number.
- Therefore, the prime factorization of 1155 is:
1155=51×31×71×111
Next, we identify the common prime factors from the factorizations:
- 5 appears in all three factorizations.
- 3 appears in all three factorizations.
- 7 appears in all three factorizations.
To find the HCF, we take the lowest power of each common prime factor:
- For 3: The lowest power is 31.
- For 7: The lowest power is 71.
HCF=51×31×71=5×3×7=105
The largest possible length of the tape that can measure 525 cm, 1050 cm, and 1155 cm is 105 cm.
Topper's Solved these Questions
- FUNDAMENTALS Book:ARIHANT SSCChapter:FUNDAMENTALS Exercise:INTRODUCTORY EXERCISE - 1.5
Explore 9 Videos
- FUNDAMENTALS Book:ARIHANT SSCChapter:FUNDAMENTALS Exercise:INTRODUCTORY EXERCISE - 1.6
Explore 5 Videos
- FUNDAMENTALS Book:ARIHANT SSCChapter:FUNDAMENTALS Exercise:INTRODUCTORY EXERCISE - 1.3
Explore 3 Videos
- FUNCTIONS AND GRAPHBook:ARIHANT SSCChapter:FUNCTIONS AND GRAPHExercise:Final Round
Explore 40 Videos
- GEOMETRYBook:ARIHANT SSCChapter:GEOMETRYExercise:EXERCISE(LEVEL 2)
Explore 52 Videos
Similar Questions
Knowledge Check
Find the greatest possible length which can be used to measure 2 m,75 cm and 8 m ,65 cm
A35 cmB15 cmC5 cmD25 cmA car starts a journey of 290 km with the speed of 50 km/hr. After every two hours the speed of the car increase by 10 km/hr. So how much time will it take to cover the distance
A(a) 2 hr 10 minB(b) 4 hrC(c) 4 hr 45 minD(d) 5 hrWhat is the length of the longest tape which can be used to measure exactly 1 m 75 cm, 4 m 50 cm and 6 m 50 cm?
A25 cmB50 cmC55 cmD75 cm
The length of a cloth measured is 200 cm. Match the followign from
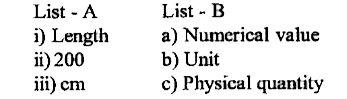
View SolutionFind the greatest possible length which can be used to measure exactly the lengths 7m, 3m 85cm and 12m 95cm.
View SolutionThe possible maximum length (in cm) that can be used to accurately measure 495 cm, 900 cm, 1665 cm is :
View SolutionFind the greatest possible length which can be used to measure exactly the lengths 7 m, 3m 85m and 12 m 95 m.
View SolutionIf edge lengths of a cuboid are measured to be 1.2cm,1.5cm, and 1.8cm, then volume of the cuboid is
View Solution