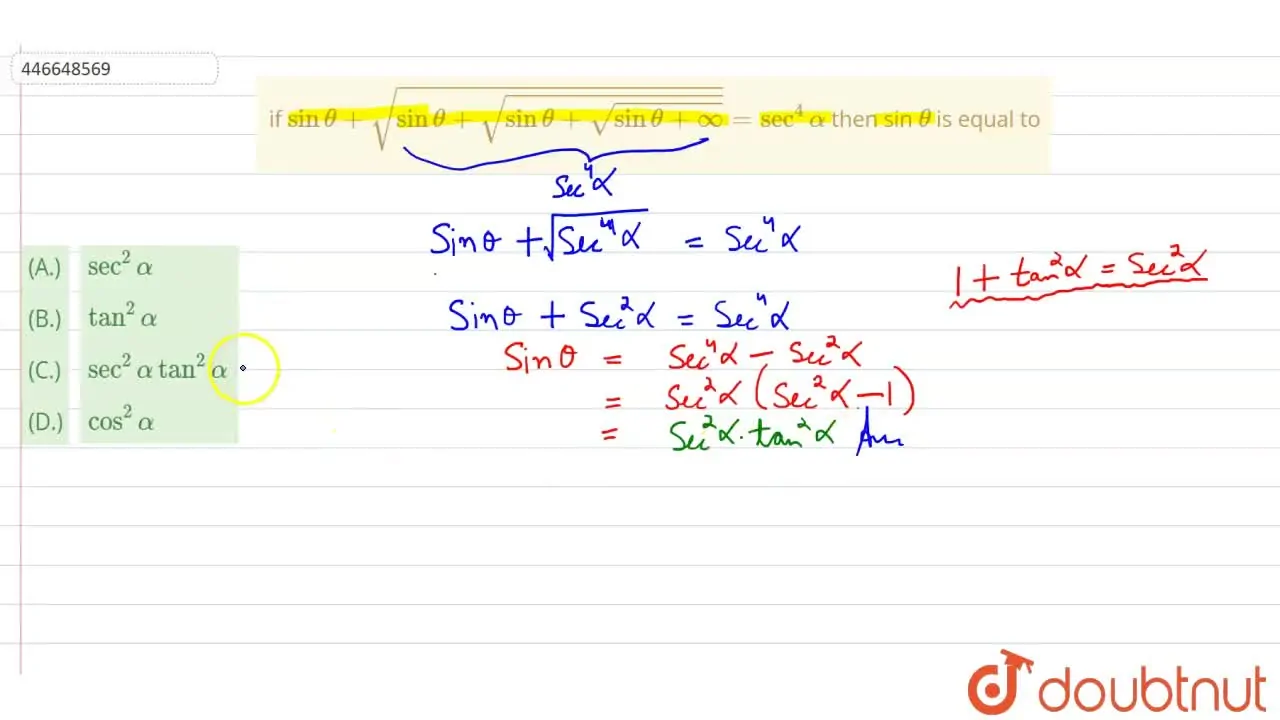
if sinθ+√sinθ+√sinθ+√sinθ+∞=sec4α then sin θ is equal to
A
sec2α
B
tan2α
C
sec2αtan2α
D
cos2α
Video Solution
Text Solution
The correct Answer is:C
|
Answer
Step by step video, text & image solution for if sin theta + sqrt(sin theta+ sqrt(sin theta+ sqrt(sin theta + oo )))=sec ^4 alpha then sin theta is equal to by Maths experts to help you in doubts & scoring excellent marks in Class 14 exams.
Updated on:21/07/2023
Related Playlists
- BOOK - ARIHANT SSCCHAPTER - PRACTICE SET EXERCISE - PRACTICE SET-450videos
PRACTICE SET
- BOOK - ARIHANT SSCCHAPTER - PRACTICE SET EXERCISE - PRACTICE SET-550videos
PRACTICE SET
- BOOK - ARIHANT SSCCHAPTER - PRACTICE SET EXERCISE - PRACTICE SET-250videos
PRACTICE SET
- BOOK - ARIHANT SSCCHAPTER - PIPES AND CISTERNS EXERCISE - EXERCISE HIGHER SKILL LEVEL QUESTION17videos
PIPES AND CISTERNS
- BOOK - ARIHANT SSCCHAPTER - PROBABILITYEXERCISE - EXERCISE (LEVEL-2)19videos
PROBABILITY
Similar Questions
√1+sinθ1−sinθ=?
View SolutionIf sinθ=√3cosθ,then θ is equal to
View SolutionIf √3sinθ−cosθ=√2, then θ=
View SolutionIf cosθ−sinθ=√2sinθ then cosθ+sinθ is equal to
View SolutionIf cosθ+sinθ=√2cosθ thencosθ−sinθ is equal to
View SolutionIf cosθ+sinθ=√2sinθ then sinθ−cosθ is
View SolutionLtx→0sin√θ√sinθ=
View SolutionIf θ=60∘ then 12√1+sinθ+12√1−sinθ=
View SolutionProve that √1+sinθ1−sinθ+√1−sinθ1+sinθ=2secθ
View SolutionProve that : √1−sinθ1+sinθ=secθ−tanθ
View Solution