Let a ∈R and let f: R→R be given by f(x) =x5−5x+a. then

A

f(x) has three real roots if a>4

B

f(x) has only one real root if a>4

C

f(x) has three real roots if a<−4

D

f(x) has three real roots if −4<a<4

Video Solution
Doubtnut Promotions Banner
Text Solution
Verified by Experts

The correct Answer is:B, D

Let g(x)x5−5x and h(x)=-a. Then, the number of real roots of f(x)=0 is the number of points of intersection of the curves y=g(x) and y=h(x). Clearly, these two curves intersect at three points, if −4<a<4.
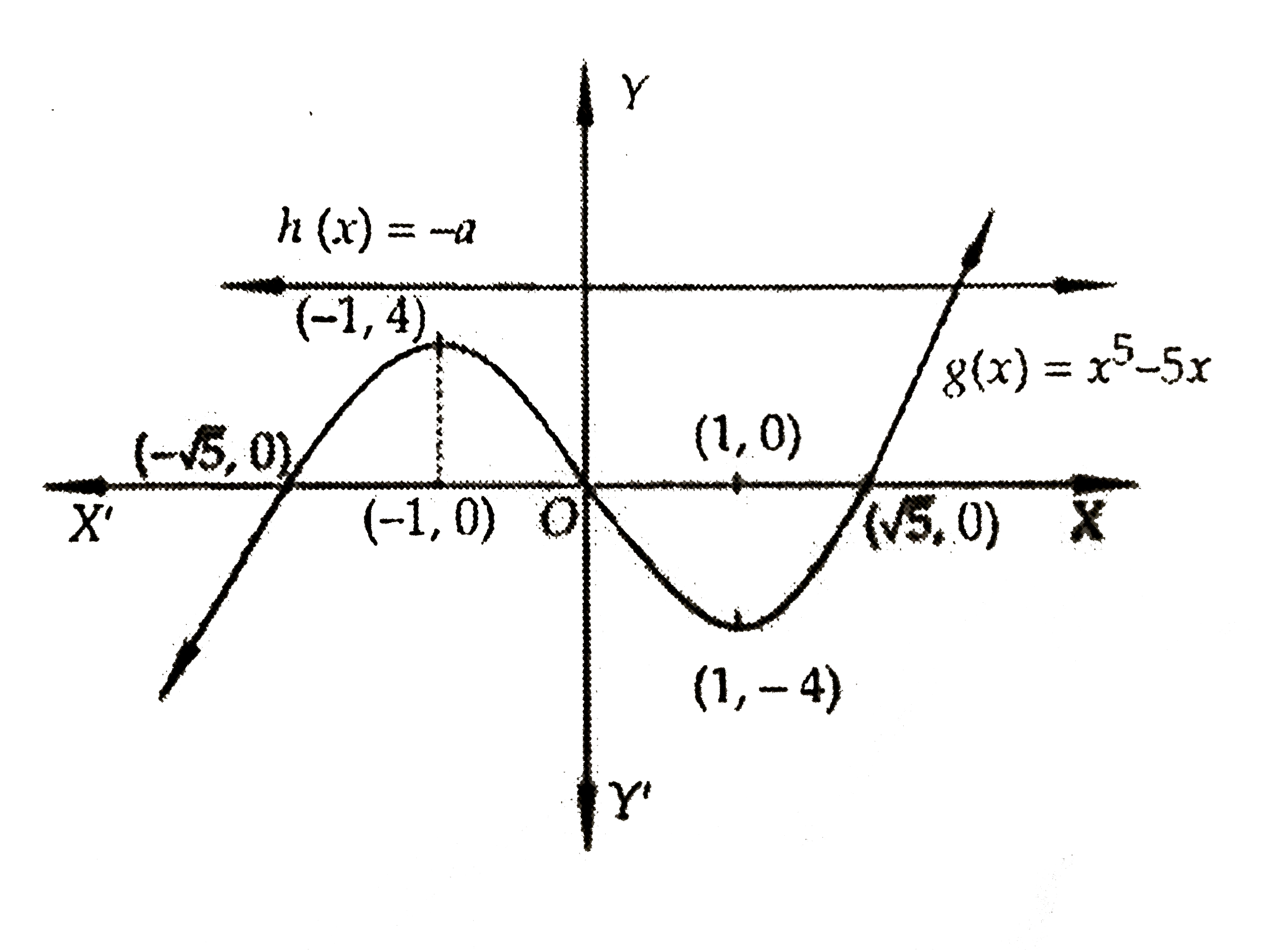
ocr_image
So, f(x) has three real roots, if 4<a<4.
The two curves y=g(x) and y=h(x) intersect at exactly one point if a>4 or a<−4.
So, f(x) has only one real root if a<−4 or a>4.

Updated on:21/07/2023
|
Class 12MATHSMISCELLANEOUS EQUATIONS AND INEQUATIONS

Topper's Solved these Questions

Similar Questions

Knowledge Check

  • Let f:R→Randg:R→R be given by f(x)=x2andg(x)=x3+1, then (fog) (x)

    Ax6+1
    Bx6−1
    C(x3−1)2
    D(x3+1)2

  • Let f:R→R be defined by f(x)=x4, then

    Af is one-one and onto
    Bf may be one-one and onto
    Cf is one-one but not onto
    Df is neither one-one nor onto

  • f:R→R given by f(x)=5-3 sin x, is

    Aone-one
    Bonto
    Cone-one and onto
    Dnone of these