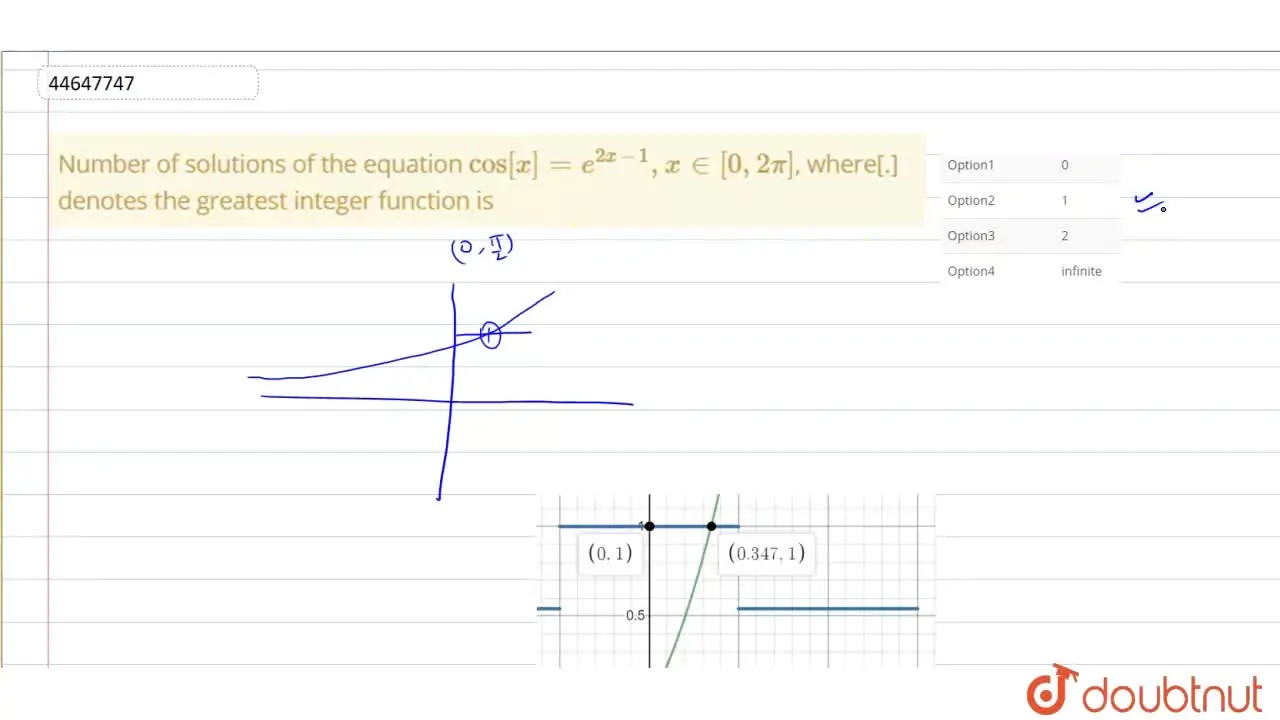
Number of solutions of the equation cos[x]=e2x−1,x∈[0,2π], where[.] denotes the greatest integer function is
0
1
2
infinite
The correct Answer is:B
It is evident from the graph that the two curves intersect at only one point.

Hence, the given equation has exactly one solution.
Related Playlists
- BOOK - OBJECTIVE RD SHARMACHAPTER - MISCELLANEOUS EQUATIONS AND INEQUATIONS EXERCISE - Exercise30videos
MISCELLANEOUS EQUATIONS AND INEQUATIONS
- BOOK - OBJECTIVE RD SHARMACHAPTER - MISCELLANEOUS EQUATIONS AND INEQUATIONS EXERCISE - Chapter Test30videos
MISCELLANEOUS EQUATIONS AND INEQUATIONS
- BOOK - OBJECTIVE RD SHARMACHAPTER - MEASURES OF CENTRAL TENDENCYEXERCISE - Chapter Test21videos
MEASURES OF CENTRAL TENDENCY
- BOOK - OBJECTIVE RD SHARMACHAPTER - PLANE AND STRAIGHT LINE IN SPACE EXERCISE - Chapter Test31videos
PLANE AND STRAIGHT LINE IN SPACE
Similar Questions
If f(x)=[2x], where [.] denotes the greatest integer function, then
View SolutionNumber of solutions of the equation x2−2=[sinx], where [.] denotes the greatest integer function, is
View SolutionFind the number of solution of the equations
|cosx|=[x], (where [.] denotes the greatest integer function ).View SolutionDraw the graph of [y]=cosx,x∈[0,2π], where [⋅] denotes the greatest integer function.
View SolutionThe range of the function f(x)=cosec−1[sinx] in [0,2π], where [⋅] denotes the greatest integer function , is
View SolutionDraw the graph of [y]=sinx,x∈[0,2π] where [⋅] denotes the greatest integer function
View SolutionTotal number of solutions of the equation x²−4−[x]=0 are (where (.) denotes the greatest integer function)
View SolutionTotal number of solution for the equation x2−3[sin(x−π6)]=3 is _____(where [.] denotes the greatest integer function)
View SolutionNumber of solutions of the equation 5{x}=x+[x] and x- [x] =12 (where [.] denotes greatest integer function and {x} denotes fractional part of x ) is
View SolutionThe range of the function y=[x2]−[x]2 x∈[0,2] (where [] denotes the greatest integer function), is
View Solution