The number of real solutions of the equation logax=|x|,0<a<1, is

A

0

B

1

C

2

D

None of these

Video Solution
Doubtnut Promotions Banner
Text Solution
Verified by Experts

The correct Answer is:B

The number of real solutions of the equation logax=|x| is equal to the number of points of intersection of the curves
y=loga x and y=|x|
The graphs of these two curves are shown in Fig.3.
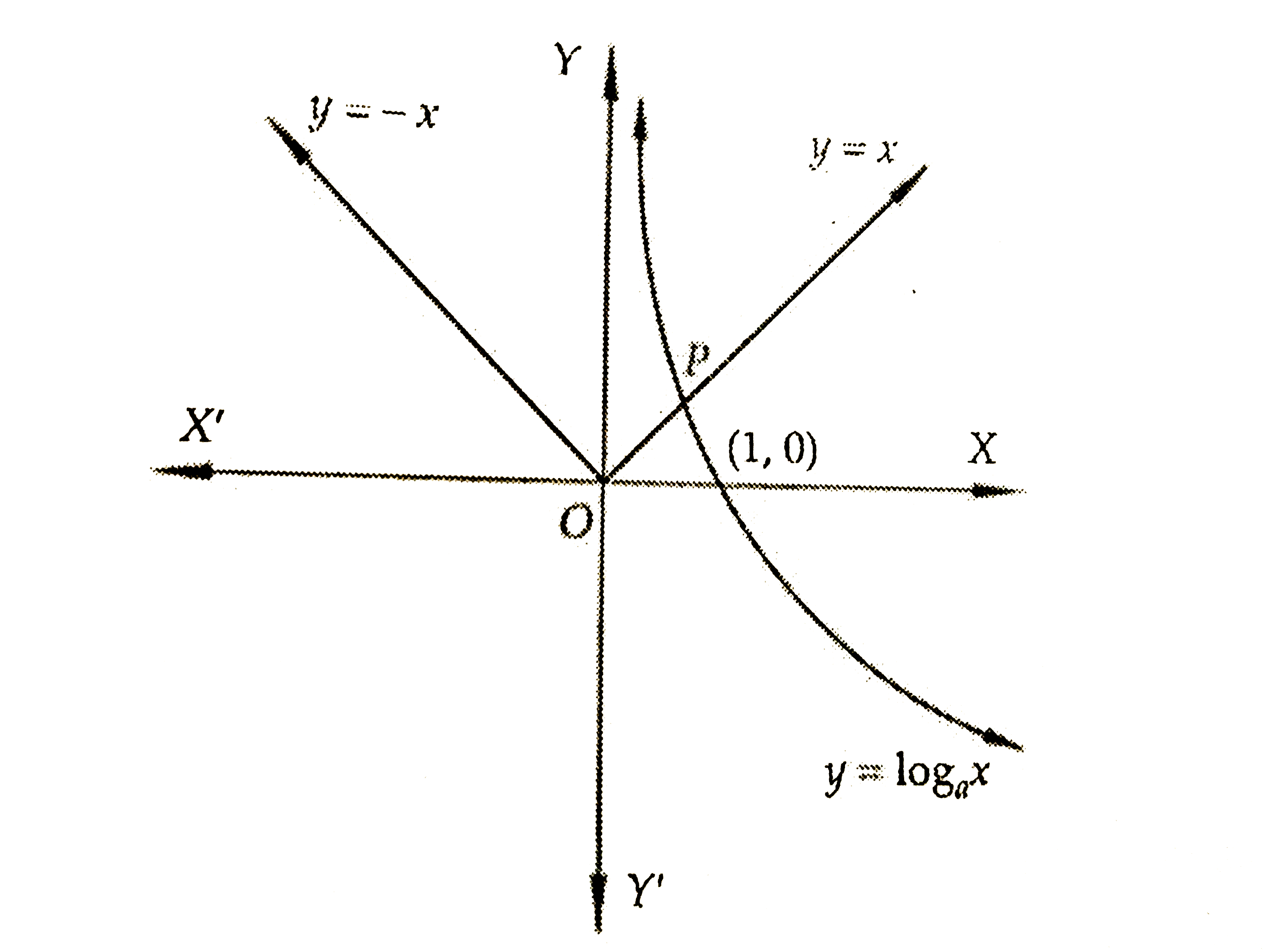
ocr_image
It is evident the graph that the two curves intersect at one point only. Hence, the given equation has one real solution lying between 0 and 1.

Updated on:21/07/2023
|
Class 12MATHSMISCELLANEOUS EQUATIONS AND INEQUATIONS

Topper's Solved these Questions

Similar Questions

Knowledge Check

  • The number of real solutions of the equation log(−x)=2log(x+1) is

    A1
    B2
    C0
    DNone of these

  • The set S={1,2,3,...,12} is to be partitioned into three sets, A, B, C of equal size. ThusA∪B∪C=S,A∩B=B∩C=A∩C=ϕ. The number of ways to partition S is

    A1
    B2
    C3
    Dmore than 3

  • The number of real solutions of log2x+|x|=0, is

    A0
    B1
    C3
    D4