Let P be a point on the ellipse x2a2+y2b2=1,0<b<a and let the line parallel to y-axis passing through P meet the circle x2+y2=a2 at the point Q such that P and Q are on the same side of x-axis. For two positive real numbers r and s, find the locus of the point R on PQ such that PR:RQ=r:s and P varies over the ellipse.
The correct Answer is:A, B
given .PRRQ=Rs
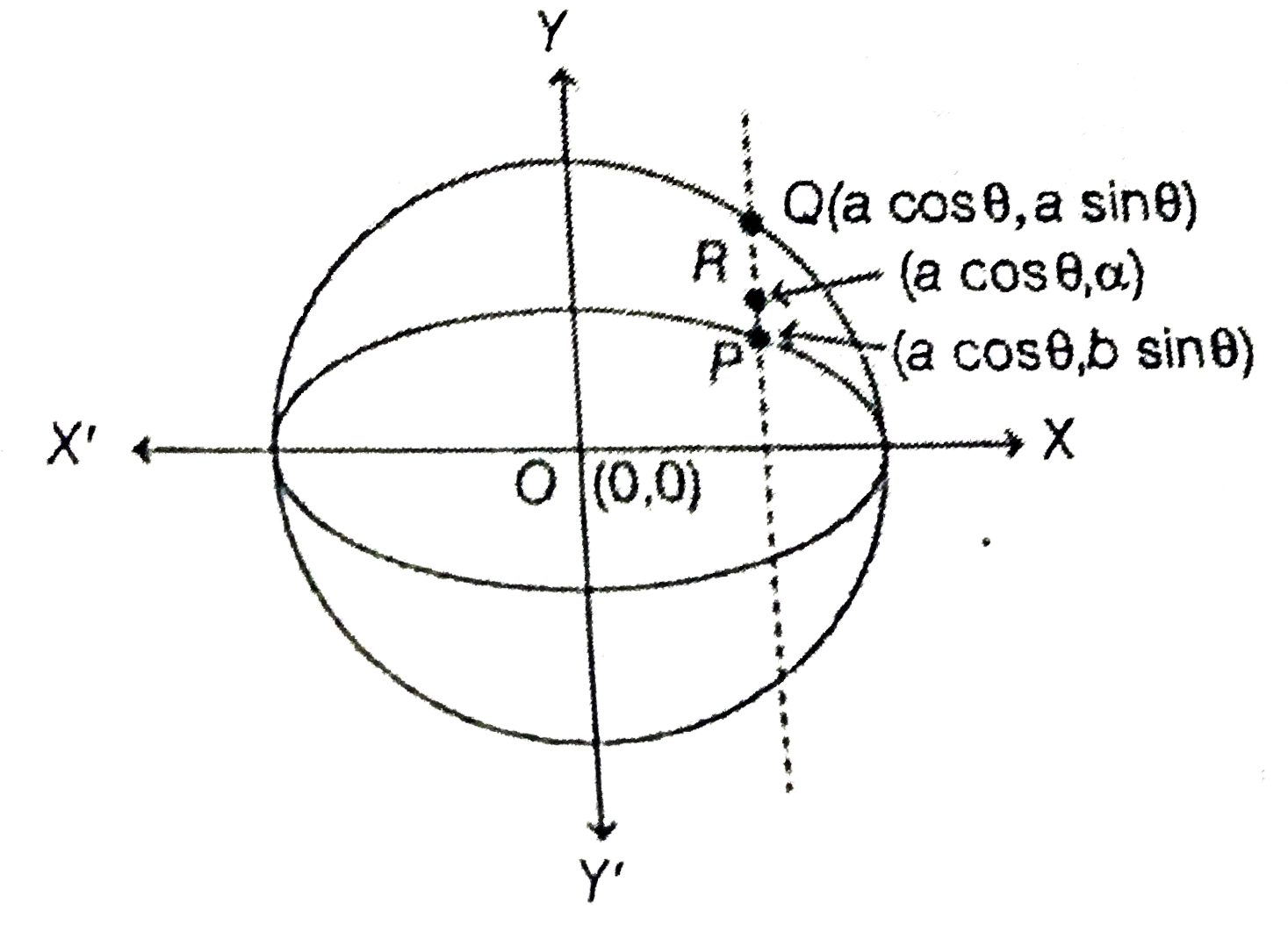
⇒α−bsinθα−θ−α=rs
⇒αs−bsinθ.s=rasinθ−αr
⇒αs+αr=rαsinθbsinθ.s
⇒α(s+r)=sinθ(ra+bs)
⇒α=sinθ(ra+bs)r+s
Let the coordinates of R be (h.k)
∴ h= a cos θ⇒cosθ=hα...(i)
andk=α=(ar+bs)bsinθr+s
On squaring and adding Eqs. (i) and (ii) , we get
sin2θ+cos2θ=h2a2+k2(r+s)2(ar+bs)2
⇒1=h2a2+k2(r+s)2(ar+bs)2=1.
hence , locus of R x2a2+y2(r+s)2(ar+bs)2=1.
Topper's Solved these Questions
- ELLIPSEBOOK - IIT JEE PREVIOUS YEARCHAPTER - ELLIPSEEXERCISE - EQUATION OF TANGENT AND NORMAL
23 Videos
- ELLIPSEBOOK - IIT JEE PREVIOUS YEARCHAPTER - ELLIPSEEXERCISE - EQUATION OF CHORD OF CONTACT, CHORD BISECTED AT A GIVEN POINT & DIAMETER
3 Videos
- DIFFERENTIAL EQUATIONS BOOK - IIT JEE PREVIOUS YEARCHAPTER - DIFFERENTIAL EQUATIONS EXERCISE - TOPIC 3 APPLICATIONS OF HOMOGENEOUS DIFFERENTIAL EQUATIONS <br> ANALYTICAL & DESCRIPTIVE QUESTIONS
7 Videos
- FUNCTIONSBOOK - IIT JEE PREVIOUS YEARCHAPTER - FUNCTIONSEXERCISE - INVERSE AND PERIODIC FUNCTIONS
11 Videos
Similar Questions
Let P be a point on the ellipse x2a2+y2b2=1 of eccentricity e. If A,A' are the vertices and S,S are the foci of the ellipse, then find the ratio area PSS'' : area APA′.
View SolutionLet P be a variable point on the ellipse x2a2+y2b2=1 with foci S(ae,0) and S'(−ae,0) If A is the area of the triangle PSS' then the maximum value of A is
View Solution
Knowledge Check
P is a point on the ellipse x2a2+y2b2=1(a>b) and Q is the point corresponding to P on the auxiliary circle x2+y2=a2. N is the foot of the perpendicular from P on the major axis of the ellipse. PNPQ is equal to:
Aba−bBaa−bCba+bDaa+bP1(θ1) and P2(θ2) are two points on the ellipse x2a2+y2b2=1 such that tan θ1 tan θ2=−a2b2. The chord joining P1 and P2 of the ellipse subtends a right angle at the
Afocus (ae,0)Bfocus (-ae, 0)Ccentre (0,0)Dvertex (a,0)Let P be a variable point on the elipse x2a2+y2b2=1 with foci F1andF2. If A is the area of the triangle PF1F2, then maximum value of A is
AabeBabe(c)C2abeDnone
Let P be a point on the ellipse x2100+y225=1 and the length of perpendicular from centre of the ellipse to the tangent to ellipse at P be 5√2andF1andF2 be the foci of the ellipse, then PF1.PF2.
View SolutionNumber of points on the ellipse x2a2+y2b2=1 at which the normal to the ellipse passes through at least one of the foci of the ellipse is
View SolutionLet P be a variable point on the ellipse x2a2+y2b2=1 with foci F1 and F2. If A is the area of the △PF1F2, then the maximum value of A is
View SolutionLet F1(0, 3) and F2(0, -3) be two points and P be any point on the ellipse 25x2+16y2=400, then value of (PF1+PF2) is
View SolutionLet P be a variable point on the ellipse x225+y216=1 with foci at S and S'. If A be the area of triangle PSS' then the maximum value of A, is
View Solution