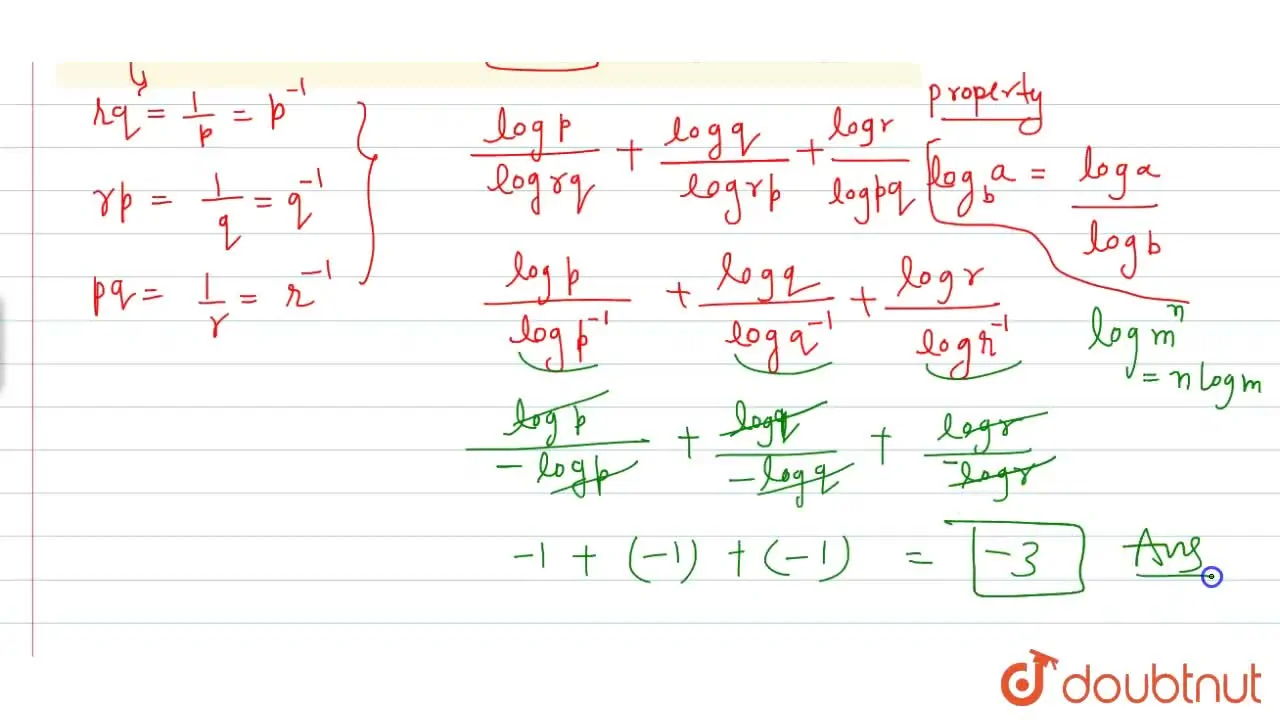
If pqr = 1 then find the value of logrqp+logrpq+logpqr.
A
0
B
-1
C
-3
D
1
Video Solution
Text Solution
The correct Answer is:C
(i) Use logaa=1.
(ii) Replace rq=p−1,rp=q−1 and pq=r−1 in the given expression.
(iii) Simplify and eliminate logarithms.
|
Updated on:21/07/2023
Related Playlists
- BOOK - PEARSON IIT JEE FOUNDATIONCHAPTER - LOGARITHMSEXERCISE - Level 315videos
LOGARITHMS
- BOOK - PEARSON IIT JEE FOUNDATIONCHAPTER - LOGARITHMSEXERCISE - Level 125videos
LOGARITHMS
- BOOK - PEARSON IIT JEE FOUNDATIONCHAPTER - LINEAR PROGRAMMINGEXERCISE - Level 310videos
LINEAR PROGRAMMING
- BOOK - PEARSON IIT JEE FOUNDATIONCHAPTER - MATHEMATICAL INDUCTION AND BIONOMIAL THEOREMEXERCISE - LEVEL 315videos
MATHEMATICAL INDUCTION AND BIONOMIAL THEOREM
Similar Questions
find the value of log2log2log216=
View SolutionFind the value of logslogs(3125)
View SolutionFind the value of log5log2log3log2512.
View SolutionFind the value of log2(17log70.125).
View SolutionFind the value of x, log4(x+3)−log4(x−1)=log48−2
View SolutionFind the value of log2[log2{log3(log3273)}]
View SolutionIf p and q are positive numbers other than 1, then the least value of ∣∣logqp+logpq∣∣ is ______.
View SolutionIf logp(q)+logq(r)+logr(p) vanishes where p,q,r are positive reals different than unity then the value of (logp(q))3+(logq(r))3+(logr(p))3
View SolutionIf a,b,c be the pth , qth, rth terms of a GP then the value of (q-r)log a+ (r-p) log b + (p-q) log c is:
View Solution1.If log102=p ,then the value of log105 is
View Solution