The minimum value of f=x+4y subject to the constraints $x + y \geq 8, 2 x + y \geq 10, x > 0, y > 0$ is __________

A

4

B

26

C

5

D

8

Video Solution

Text Solution

Verified by Experts

The correct Answer is:D

$x + y \geq 8, 2 x + y \geq 10, x \geq 0, y \geq 0$
Solving $x + y = 8, 2 x + y = 10, w e \geq t (x, y) = (2, 6)$
ABC is an open- convex polygon.

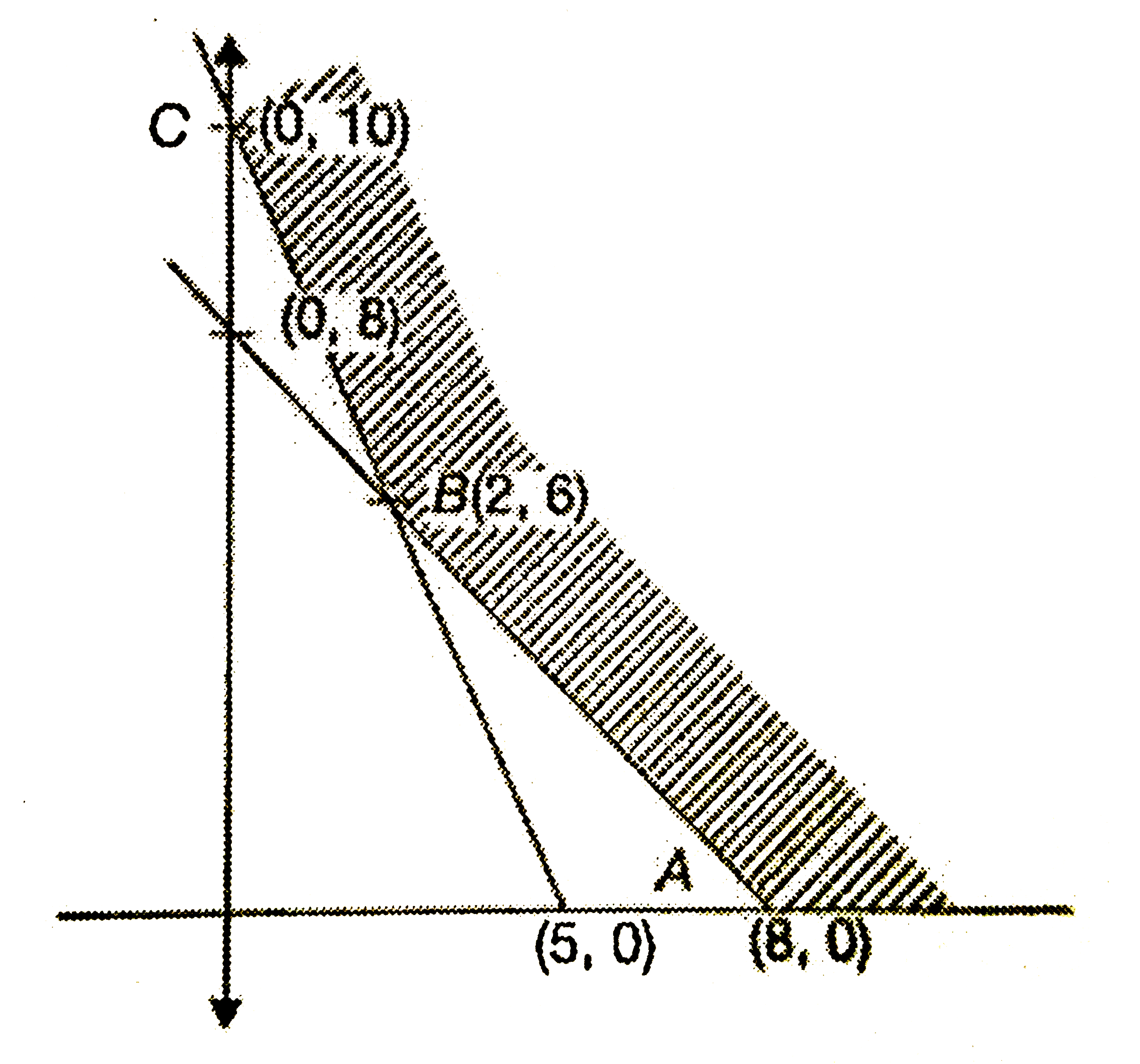
The vertices are A(8,0), B(2,6), C(0,10)
F=x+4y
A(8,0), f=8+4(0)=8
C(0,10),f=0+4(10)=40
B(2,6), f=2+4(6)=26
$∴$ The minimum value of f is 8.
It attains at A(8,0).

|

Updated on:21/07/2023

Class 10 MATHS LINEAR PROGRAMMING

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
The point at which the minimum value of $z = 8 x + 12 y$ subject to the constraints $2 x + y \geq 8, x + 2 y \geq 10 x \geq 0, y \geq 0$ is obtained is
A(8,0)
B(9, 1)
C(2, 4)
D(10, 0)
Question 2 - Select One
The minimum value of $Z = 4 x + 5 y$ subjected to the constraints $x + y \geq 6, 5 x + y \geq 10, x \geq 0, y \geq 0$ is
A28
B24
C30
D31
Question 3 - Select One
The maximum value of Z = 4x + 2y subject to the constraints $2 x + 3 y \leq 18, x + y \geq 10, x, y \geq 0$ is
A20
B36
C40
DNone of these

PEARSON IIT JEE FOUNDATION-LINEAR PROGRAMMING-Level 3

Home
Ask Doubt
Profile