The difference in energy of radition emitted (or) absorted during electronic transition is
directly proportional to frequency
directly proportional to wavelenght
inversely proportional to frequency
inversely proportional to velocity of light
To solve the question regarding the difference in energy of radiation emitted or absorbed during electronic transitions, we can follow these steps:
1. Understand the Relationship Between Energy and Frequency:
The energy (E) of radiation can be expressed using the formula:
E=hν
where E is the energy, h is Planck's constant, and ν (nu) is the frequency of the radiation.
2. Relate Frequency to Wavelength:
The frequency (ν) is related to the wavelength (λ) of the radiation by the equation:
ν=cλ
where c is the speed of light.
3. Substituting Frequency in the Energy Equation:
By substituting the expression for frequency into the energy equation, we get:
E=h(cλ)
This can also be expressed as:
E=hcλ
4. Analyzing the Relationships:
From the equation E=hν and E=hcλ, we can analyze the relationships:
- Energy is directly proportional to frequency (E∝ν).
- Energy is inversely proportional to wavelength (E∝1λ).
- Energy is directly proportional to the speed of light (E∝c).
5. Conclusion:
The question asks for the relationship of energy during electronic transitions. The correct answer is that the difference in energy of radiation emitted or absorbed during electronic transitions is directly proportional to frequency.
Final Answer:
The difference in energy of radiation emitted (or absorbed) during electronic transitions is directly proportional to frequency.
---
The energy (E) of radiation can be expressed using the formula:
E=hν
where E is the energy, h is Planck's constant, and ν (nu) is the frequency of the radiation.
The frequency (ν) is related to the wavelength (λ) of the radiation by the equation:
ν=cλ
where c is the speed of light.
By substituting the expression for frequency into the energy equation, we get:
E=h(cλ)
This can also be expressed as:
E=hcλ
From the equation E=hν and E=hcλ, we can analyze the relationships:
- Energy is directly proportional to frequency (E∝ν).
- Energy is inversely proportional to wavelength (E∝1λ).
- Energy is directly proportional to the speed of light (E∝c).
The question asks for the relationship of energy during electronic transitions. The correct answer is that the difference in energy of radiation emitted or absorbed during electronic transitions is directly proportional to frequency.
The difference in energy of radiation emitted (or absorbed) during electronic transitions is directly proportional to frequency.
Topper's Solved these Questions
- ATOMIC STRUCTURE Book:PEARSON IIT JEE FOUNDATIONChapter:ATOMIC STRUCTURE Exercise:LEVEL-2
Explore 24 Videos
- ATOMIC STRUCTURE Book:PEARSON IIT JEE FOUNDATIONChapter:ATOMIC STRUCTURE Exercise:LEVEL-3
Explore 8 Videos
- ATOMIC STRUCTURE Book:PEARSON IIT JEE FOUNDATIONChapter:ATOMIC STRUCTURE Exercise:Short Answer type Question
Explore 3 Videos
- ACIDS, BASES AND SALTSBook:PEARSON IIT JEE FOUNDATIONChapter:ACIDS, BASES AND SALTSExercise:Level 3
Explore 9 Videos
- CHEMICAL KINETICS AND EQUILIBRIUMBook:PEARSON IIT JEE FOUNDATIONChapter:CHEMICAL KINETICS AND EQUILIBRIUMExercise:Level 3
Explore 10 Videos
Similar Questions
Calculate the wavelength and energy of radiation emitted for the electron transition from infinity to stationary state of the hydrogen atom
View SolutionWhen an electron falls from a higher energy to a lower energy level, the difference in the energies appears in the forms of electromagnetic radiation. Why cannot it be emitted as other forms of energy?
View Solution
Knowledge Check
The wavelength of the radiation emitted by a hydrogen atom in the electronic transition from n=3 to n=2 is λ. For the same transition in the singly ionized helium, the wavelength of the emitted radiation is
Aλ/4Bλ/2C2λD4λAn argon-ion laser emits a blue-green beam of light with a wavelength of 488 nm in a vacuum. What is the difference in energy in joules between the two energy states for the atomic transition that produces this light?
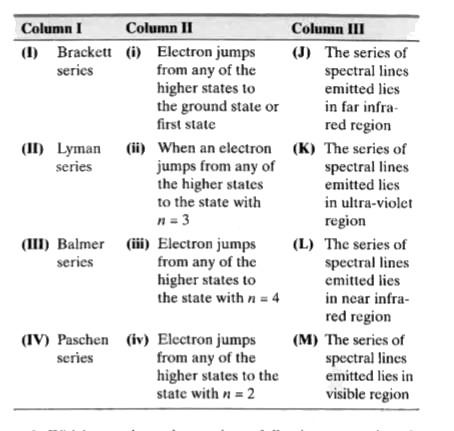
A4.08×10−19JB6.18×10−20JC1.05×10−20JD4.76×10−24JIn a hydrogen atom, whenever there is transition of electron between different energy levels, energy is emitted or absorbed, and hydrogen spectrum is obtained. In the given table, Column I shows the names of the different series of hydrogen atom spectrum, Column II shows the energy levels from where electron transition takes place and Column III shows the EM wave region where these different series exists.

Which series has the following wavelength v=RZ2(142−1n22)?A(II)(ii)(J)B(I)(iii)(J)C(IV)(i)(L)D(II)(iii)(M)
Hydrogen spectrum||Different series||Electronic transition
View SolutionThe electron in a given Bohr orbit has a total energy of -1.51 eV.
calculate the wavelength of radiation emitted, when this electron makes a transition of the ground state.
View SolutionWhen a photon is emitted from an atom , the atom recils The kinetic energy of recoils and the energy of the photon come from the difference in energy between the state involved in the transition suppose a hydrogen atom change its state from n=3→n=2 calculate the fractional change in the wavelength of light emitted , due to the recoil
View SolutionIn a hydrogen atom, whenever there is transition of electron between different energy levels, energy is emitted or absorbed, and hydrogen spectrum is obtained. In the given table, Column I shows the names of the different series of hydrogen atom spectrum, Column II shows the energy levels from where electron transition takes place and Column III shows the EM wave region where these different series exists.
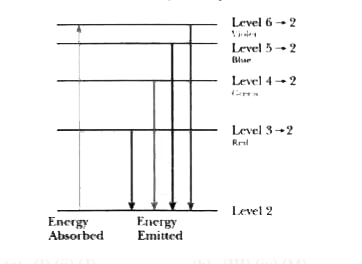
Which series does the diagram depict?
View SolutionKinetic energy of electrons emitted in photoelectric effect is
View Solution