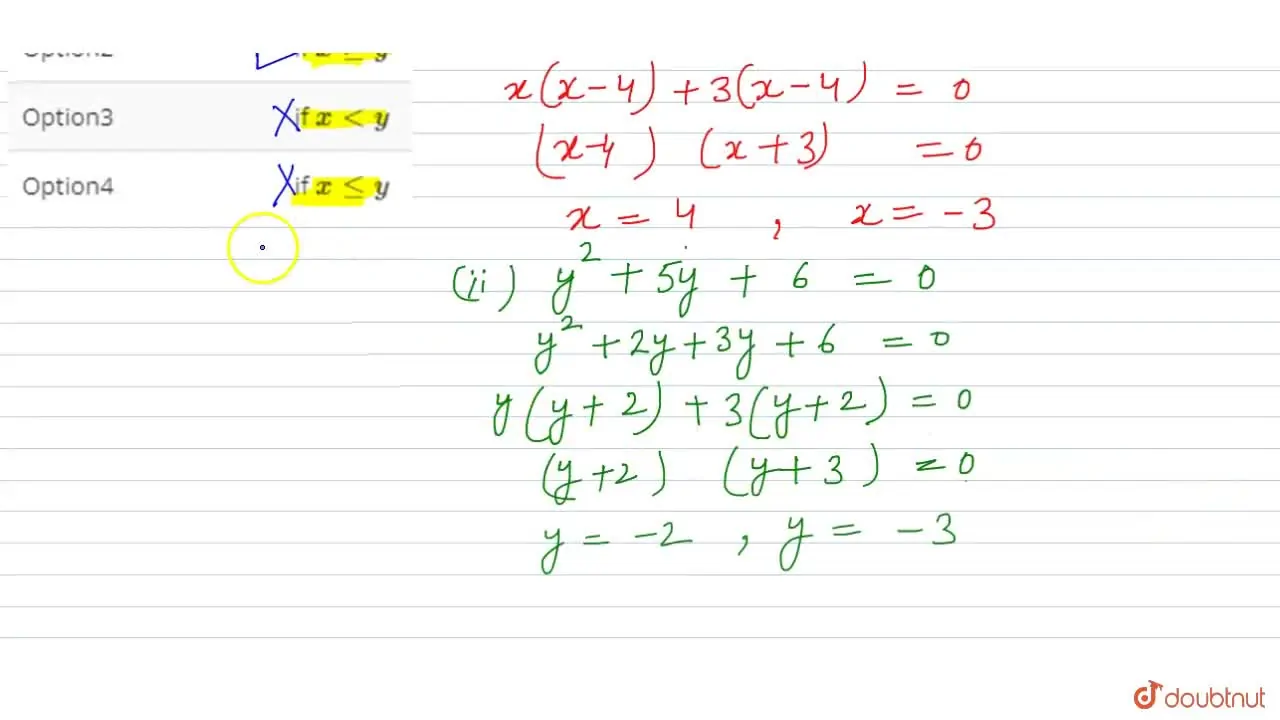
x2−x−12=0,y2+5y+6=0
if x>y
if x≥y
if x<y
if x≤y
The correct Answer is:B
Step by step video, text & image solution for x^(2)-x-12=0, y^(2) + 5y + 6=0 by Maths experts to help you in doubts & scoring excellent marks in Class 14 exams.
Related Playlists
- BOOK - ARIHANT SSCCHAPTER - QUADRATIC EQUATIONSEXERCISE - Exercise Higher Skill Level questions19videos
QUADRATIC EQUATIONS
- BOOK - ARIHANT SSCCHAPTER - QUADRATIC EQUATIONSEXERCISE - Multi concept questions3videos
QUADRATIC EQUATIONS
- BOOK - ARIHANT SSCCHAPTER - PROFIT, LOSS AND DISCOUNTEXERCISE - EXERCISE (LEVEL 2)45videos
PROFIT, LOSS AND DISCOUNT
- BOOK - ARIHANT SSCCHAPTER - RACES AND GAMES OF SKILLEXERCISE - FAST TRACK PRACTICE27videos
RACES AND GAMES OF SKILL
Similar Questions
In the following questions two equation numbered I and II are given You have to solve both equations and . . . . .
(i) x2−x−12=0
(ii) y2+5y+6=0View SolutionEquation of radical axis of the circles x2+y2−3x−4y+5=0 and 2x2+2y2−10x−12y+12=0 is
View SolutionIf line 2x−y+k=0 is a diameter of circle
x2+y2+6x−6y+5=0 , then k =View SolutionIn the following question, two equations numbered I and II are given. You have to solve both the equations and give the answer:
I. x2−x−12=0
II. y2+5y+6=0View SolutionI. x2+5x+6=0
II. y2+7y+12=0View SolutionIf 8x2+y2−12x−4xy+9=0 , then the value of (14x - 5y) is:
यदि 8x2+y2−12x−4xy+9=0 है, तो (14x - 5y) का मान ज्ञात करें |View SolutionLengths of intercepts by circle
x2+y2−6x+4y−12=0 on line 4x−3y+2=0 isView SolutionLet the equation of circle is x2+y2−6x+4y+12=0. Then the line 3x−4y+5 is a
View SolutionIf the distance from P to the points (5,-4),(7,6) are in the ratio 2:3 ,then the locus of P is
5x2+5y2−12x−86y+17=0,
5x2+5y2−34x+120y+29=0,
5x2+5y2−5x+y+14=0
3x2+3y2−20x+38y+87=0View SolutionI. 15x2−29x−14=0
II. 6y2−5y−25=0View Solution