A rectangular loop carryinig current I is located near an infinite long straight conductor carrying current I as shown in the figure. The loop,

The infinitely long wire which is carrying current I1 is providing non-uniform magnetic field for the square loop which is carrying current I2=1A . Forces on the wire CD and EA are equal and opposite as the magnetic field due long wire is same at their location.
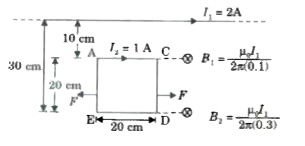
The wire AC and DA are present in uniform magnetic field B1 and B2 respectively,
So, they will experiences force as given below:
Force on wire AC is
FAC=I2B1asin90∘
I2×μ0I12π(0.1)×20100×1
=1×4π×10−7×22π×(0.1)×(0.2)
=8×10−7N , upwards
Force on wire DE is
FDE=I2B2asin90∘
I2μ0I12π(0.3)×0.2×1
=1×4π×10−7×2×0.22π×90.3
=2.67×10−7N, downwards
The net force on the square loop of wire :
F=FAC−FDE
=8×10−7−2.67×10−7
F=5.34×10−7N, upwards
Topper's Solved these Questions
- MOVING CHARGES AND MAGNETISMBOOK - MODERN PUBLICATIONCHAPTER - MOVING CHARGES AND MAGNETISMEXERCISE - COMPETITION FILE (A. MULTIPLE CHOICE QUESTIONS)
38 Videos
- MOVING CHARGES AND MAGNETISMBOOK - MODERN PUBLICATIONCHAPTER - MOVING CHARGES AND MAGNETISMEXERCISE - COMPETITION FILE (B. MULTIPLE CHOICE QUESTIONS)
79 Videos
- MOVING CHARGES AND MAGNETISMBOOK - MODERN PUBLICATIONCHAPTER - MOVING CHARGES AND MAGNETISMEXERCISE - Revision Exercises (Long Answer Questions )
12 Videos
- MAGNETISM AND MATTERBOOK - MODERN PUBLICATIONCHAPTER - MAGNETISM AND MATTEREXERCISE - CHAPTER PRACTICE TEST FOR BOARD EXAMINATION
16 Videos
- NUCLEIBOOK - MODERN PUBLICATIONCHAPTER - NUCLEIEXERCISE - CHAPTER PRACTICE TEST FOR BOARD EXAMINATION
15 Videos
Similar Questions
(a) Dfine mutaul indutace and write its S.I. unit.
(b) A square loop of side 'a' carrying a current I2 is kept at distance x from an infinitely long straight wire carrying a current I1 as shown in thefigure. Obtain the expression for the resultant force on the loop.

View SolutionA straight conductor of length ro carrying a current l is placed perpendicular to a long straight conductor which carries a current io as shown in the figure. Find the force of interaction between them.

View Solution
Knowledge Check

A square loop ABCD carrying a current i is placed near and coplanar with a long straight conductor XY carrying a current I.AThere is no net force on the loopBThe loop will be attracted by the conductor only if the current in the loop flows clockwise.CThe loop will be attracted by the conductor only if the current in the loop flows anticlockwise.DThe loop will always be attracted by the conductor.A square loop ABCD , carrying a current I, is placed near and coplanar with a long straight conductor XY carrying a current I.
 AThere is no net force on the loopBThe loop will be attracted by the conductor only if the current in the loop flows clockwise.CThe loop will be attracted by the conductor only if the current in the loop flows anticlockwiseDThe loop will always be attracted by the conductor
AThere is no net force on the loopBThe loop will be attracted by the conductor only if the current in the loop flows clockwise.CThe loop will be attracted by the conductor only if the current in the loop flows anticlockwiseDThe loop will always be attracted by the conductorA square loop ABCD, carrying a current I2 is placed near and coplanar with a long straight conductor XY, carrying a current I1 as shown in Figure. The net force on the loop will be
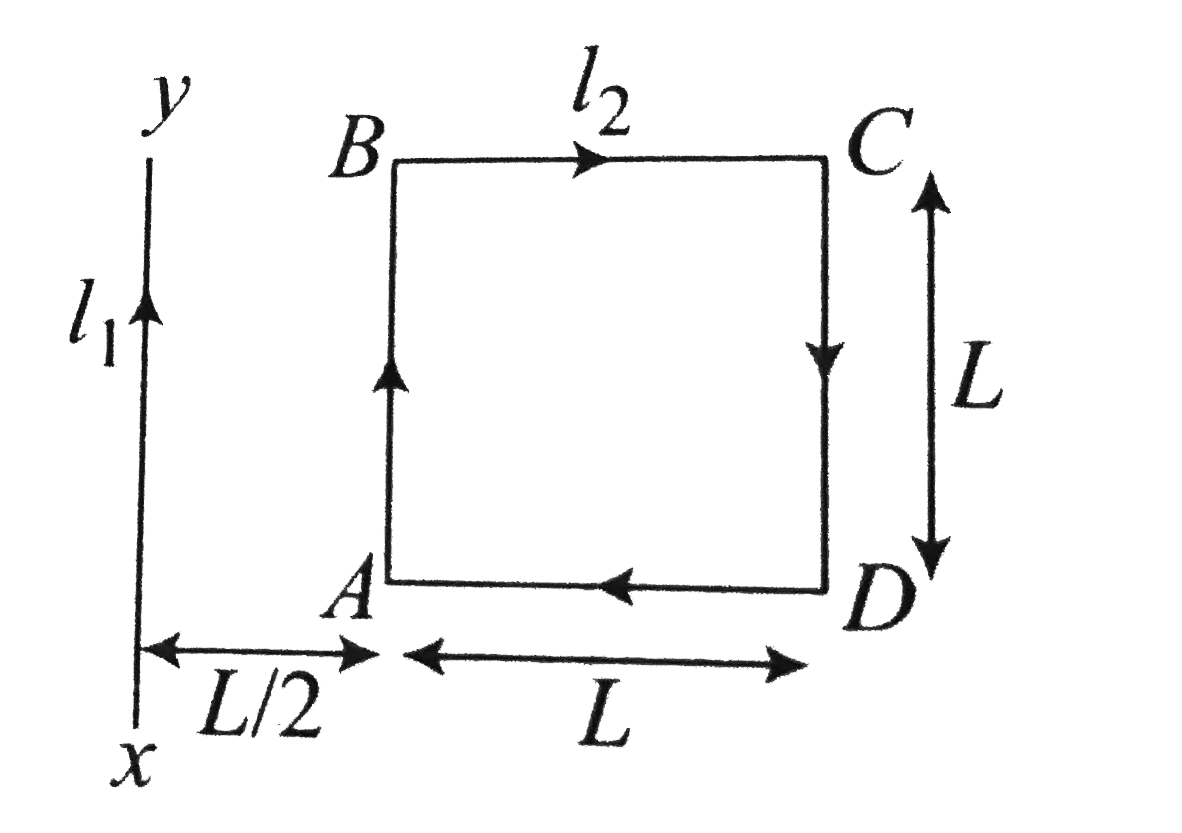 Aμ0I1I22πB2μ0I1I23πCμ0I1I2L2πD2μ0I1I2L3π
Aμ0I1I22πB2μ0I1I23πCμ0I1I2L2πD2μ0I1I2L3π
A square loop of ABCD carrying a current i, is placed near and coplanar with a long straight conductor XY carrying a current I, the net force on the loop will be
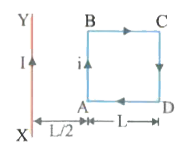
View SolutionA square loop ABCD carrying a current i, is placed near and coplanar with a long straight conductor XY carrying a current I, the net force on the loop will be
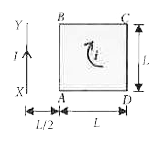
View SolutionA square loop of ABCD carrying a current i, is placed near and coplanar with a long straight conductor XY carrying a current I, the net force on the loop will be

View SolutionA conductor AB of length L carrying a current I2, IS placed perpendicular to a long straight conductor XY carrying current I2, as shown in figure. The force on AB in magnitude is

View Solution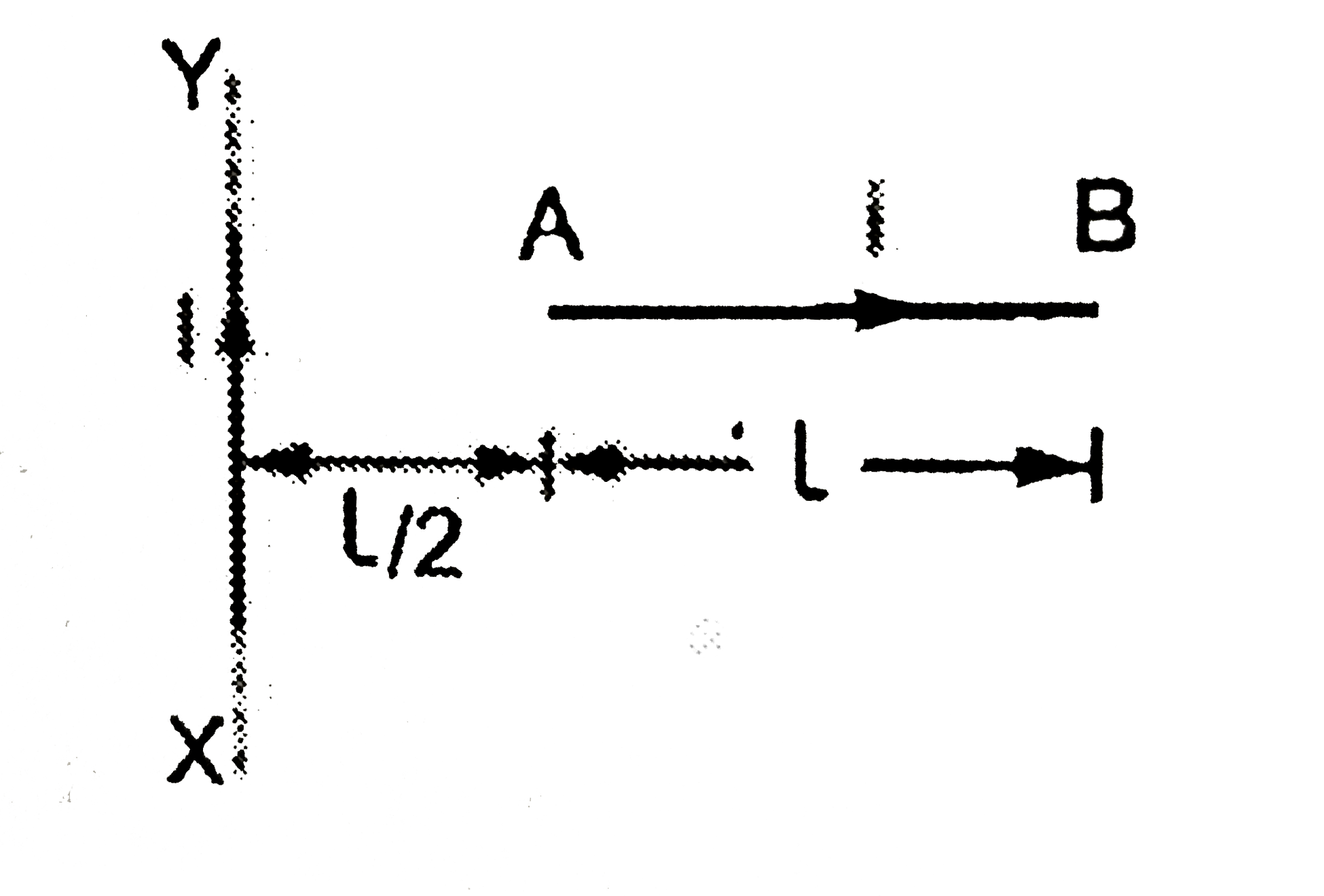
A conductor AB of length l carrying a current i is placed perpendicular to a long straight conductor XY carrying a current I, as shown. The force on AB will act
View Solution
