A very long straight current carrying conductor is carrying current of 2 A from west to east in a horizontal plane. Suddenly, a magnetic field of 4×10−5 T is switched on in vertically downward direction. Find the magnitude and direction of force per unit length of the conductor. How will the force unit length changes if the current in the wire is reversed ?
To solve the problem, we will use the formula for the magnetic force acting on a current-carrying conductor in a magnetic field. The formula is given by:
F=I⋅L⋅B⋅sin(θ)
Where:
- F is the force,
- I is the current (in Amperes),
- L is the length of the conductor (in meters),
- B is the magnetic field (in Tesla),
- θ is the angle between the direction of the current and the magnetic field.
Step 1: Identify the parameters
- Current I=2A (from west to east)
- Magnetic field B=4×10−5T (vertically downward)
- The angle θ=90∘ (since the current is horizontal and the magnetic field is vertical)
Step 2: Calculate the force per unit length
Since the angle θ is 90∘, we have:
sin(90∘)=1
Now, substituting the values into the formula:
F=I⋅B⋅sin(90∘)
F=2A⋅4×10−5T⋅1
F=8×10−5N
Step 3: Determine the direction of the force
To find the direction of the force, we can use the right-hand rule. Point your thumb in the direction of the current (east), and your fingers in the direction of the magnetic field (downward). Your palm will face in the direction of the force.
Using the right-hand rule:
- Thumb (current direction) points east.
- Fingers (magnetic field direction) point downward.
- Palm (force direction) will point towards the south.
Thus, the direction of the force is towards the south.
Step 4: Analyze the effect of reversing the current
If the current is reversed, it will flow from east to west. The magnetic field remains the same (downward).
Using the right-hand rule again:
- Thumb (current direction) now points west.
- Fingers (magnetic field direction) still point downward.
- Palm (force direction) will now point towards the north.
Thus, when the current is reversed, the force per unit length will still have the same magnitude 8×10−5N, but the direction will change to north.
Final Answer:
- Magnitude of force per unit length: 8×10−5N/m
- Direction of force: South (when current is from west to east) and North (when current is reversed).
- F is the force,
- I is the current (in Amperes),
- L is the length of the conductor (in meters),
- B is the magnetic field (in Tesla),
- θ is the angle between the direction of the current and the magnetic field.
- Current I=2A (from west to east)
- Magnetic field B=4×10−5T (vertically downward)
- The angle θ=90∘ (since the current is horizontal and the magnetic field is vertical)
Since the angle θ is 90∘, we have:
To find the direction of the force, we can use the right-hand rule. Point your thumb in the direction of the current (east), and your fingers in the direction of the magnetic field (downward). Your palm will face in the direction of the force.
- Thumb (current direction) points east.
- Fingers (magnetic field direction) point downward.
- Palm (force direction) will point towards the south.
If the current is reversed, it will flow from east to west. The magnetic field remains the same (downward).
- Thumb (current direction) now points west.
- Fingers (magnetic field direction) still point downward.
- Palm (force direction) will now point towards the north.
- Magnitude of force per unit length: 8×10−5N/m
- Direction of force: South (when current is from west to east) and North (when current is reversed).
Topper's Solved these Questions
- MOVING CHARGES AND MAGNETISMBOOK - MODERN PUBLICATIONCHAPTER - MOVING CHARGES AND MAGNETISMEXERCISE - PRACTICE PROBLEMS
59 Videos
- MOVING CHARGES AND MAGNETISMBOOK - MODERN PUBLICATIONCHAPTER - MOVING CHARGES AND MAGNETISMEXERCISE - Conceptual Questions
29 Videos
- MAGNETISM AND MATTERBOOK - MODERN PUBLICATIONCHAPTER - MAGNETISM AND MATTEREXERCISE - CHAPTER PRACTICE TEST FOR BOARD EXAMINATION
16 Videos
- NUCLEIBOOK - MODERN PUBLICATIONCHAPTER - NUCLEIEXERCISE - CHAPTER PRACTICE TEST FOR BOARD EXAMINATION
15 Videos
Similar Questions
If the current in a wire is flowing in the vertically downward direction and a magnetic field is applied from west to east, what is the direction of force on the wire ?
View SolutionFind the magnitude and direction of force on following current carrying wires. Direction of magnetic field is indicated in each case.
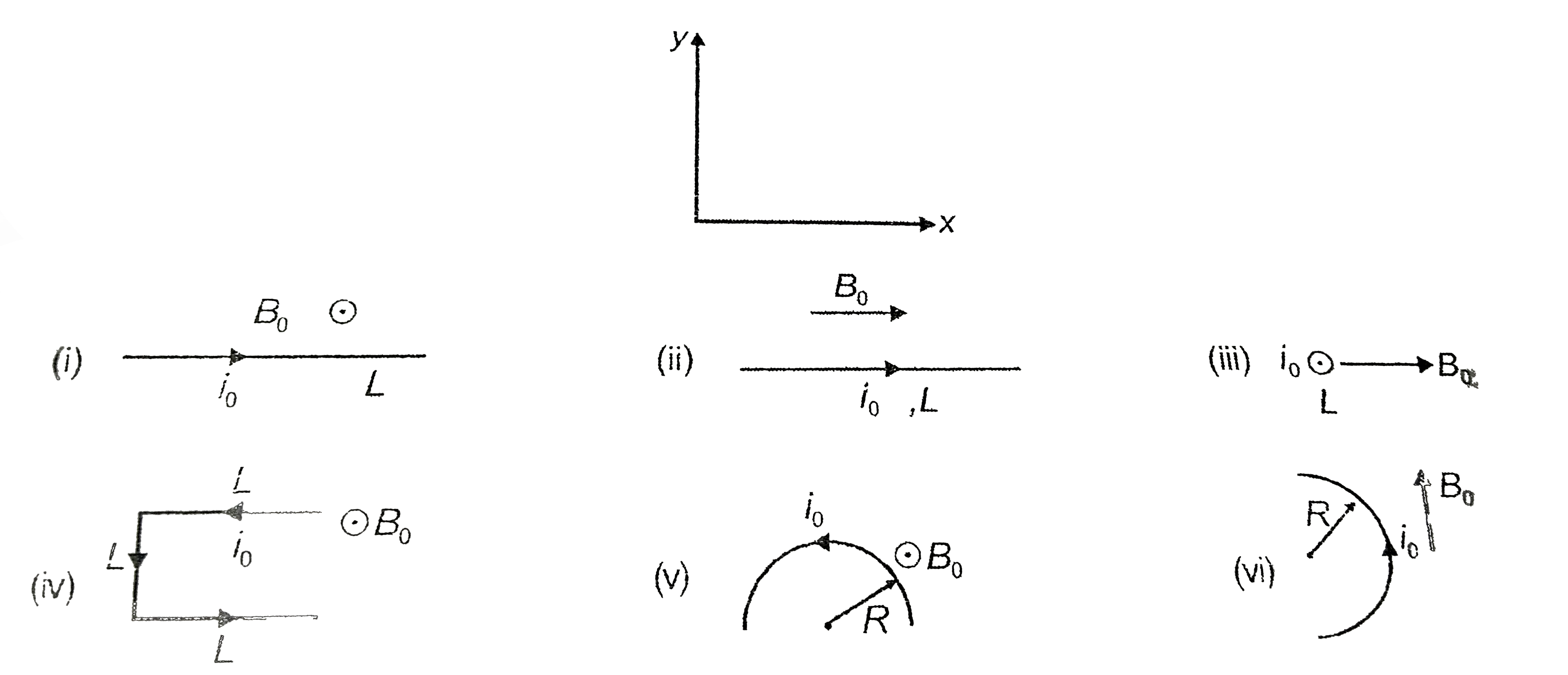
View Solution
Knowledge Check
The force acting per unit length on one conductor due to the other for two long parallel current - carrying wires is
Aμ04πI1I2rBμ04πI1I2rCμ04π2I1I2rDμ04πI1I24rA current carrying power line carries current from west to east. The direction of magnetic field 1m above is
Anorth to southBsouth to northCeast to westDwest to eastThe direction of force on a current carrying conductor in a magnetic field is given by
AFleming's left hand ruleBFleming's right hand ruleCRight hand thumb ruleDLeft hand thumb rule
Draw the magnetic field lines around a straight conductor carrying current. Name and state the rule to find the direction of magnetic field.
View SolutionThe graph of force per unit legth between two long parallel current carrying conductors and the reciprocal of the distance between them is
View SolutionThe direction of force on a current-carrying conductor in a magnetic field is given by (
View SolutionThe direction of force experienced by the current carrying conductor in a uniform magnetic field a given by
View SolutionThe direction of the force exerted by a magnetic field on a current -carrying wire is given by
View Solution
