Find the final temperature of a cooper rod. Whose area of cross section changes from 10m2 to 11m2 due to heating. The copper rod is initially kept at 90K. (Coefficient of superficial expansion is 0.0021/K)Solution in Tamil
Given: Area of copper rod A0=A1=10m2
Changes of area cross section ΔA=A2−A1
=11−10=1m2
nitial temperature t1=90K
ΔT=t2−t1
ΔT=t2=90
Coefficient of superficial expansion αA=0.0021/K
Then t2=?
αA=ΔAA0Δt
0.0021=110×(t2−90)⇒t2=90=10.0021×10
t2−90=10.021
t2=10.021+90
t2=137.6K
Topper's Solved these Questions
- THERMAL PHYSICS BOOK - FULL MARKSCHAPTER - THERMAL PHYSICS EXERCISE - TEXTUAL EVALUATION -VIII (Answer in detail)
2 Videos
- THERMAL PHYSICS BOOK - FULL MARKSCHAPTER - THERMAL PHYSICS EXERCISE - TEXTUAL EVALUATION - XI (HOT question)
1 Videos
- THERMAL PHYSICS BOOK - FULL MARKSCHAPTER - THERMAL PHYSICS EXERCISE - TEXTUAL EVALUATION -VI (Answer in brieifly)
8 Videos
- SAMPLE PAPER-9BOOK - FULL MARKSCHAPTER - SAMPLE PAPER-9EXERCISE - PART-IV
6 Videos
Similar Questions
Derive the ideal gas equation by combining the empirical gas laws.
View SolutionIf you keep lee at 0CC and water at 0∘C in either of your hands, in which hand you will feel more chillness? Why?
View SolutionDefine one calorie.
View SolutionDefine one roentgen.
View SolutionA heat flux of 4000 J/s is to be passed through a copper rod of length 10 cm and area of cross section 100cm2. The thermal conductivity of copper is 400W/m/∘C The two ends of this rod must be kept at a temperature difference of
View SolutionWhen a rod is heated, its linear expansions depends on (a) initial length (b) area of cross section (c) mass (d) temperature rise
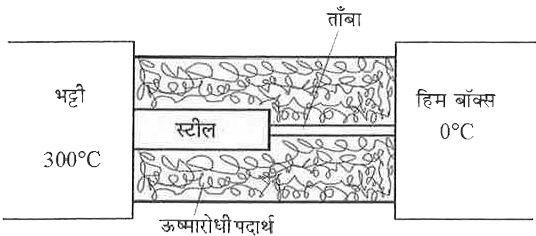
View Solutionचित्र 11.15 में दर्शाए गए निकाय की स्थाई अवस्था में स्टील - ताँबा संधि का ताप क्या है ? स्टील छड़ की लंबाई = 15.0 cm, ताँबे की छड़ की लंबाई = 10.0 cm, भट्ठी का ताप = 300∘C, दुसरे सिरे का ताप = 0∘C , स्टील की छड़ की अनुप्रस्थ काट के क्षेत्रफल ताँबे की छड़ की अनुप्रस्थ काट के क्षेत्रफल का दो गुना है | (स्टील की ऊष्मा चालकता = 50.2 J s−1m−1K−1, ताँबे की ऊष्मा चालकता = 385Js−1m−1K−1)
View SolutionA uniform metal rod of 2mm^(2) area of cross section is heated from 0^(@)C to 20^(@)C . The coefficient of linear expansion of the rod is 12xx10^(-6)//""^(@)C . Its Young's modulus of elasticity is 10^(11)N//m^(2) , then the energy stored per unit volume of rod is,
View SolutionA heat flux of 4000 J/s is to be passed through a copper rod of length 10 cm and area of cross-section 100cm2. The thermal conductivity of copper is 400 W/m/ °C. The two ends of this rod must be kept at a temperature difference of
View SolutionFind the final temperature of a cooper rod. Whose area of cross section changes from 10m2 to 11m2 due to heating. The copper rod is initially kept at 90K. (Coefficient of superficial expansion is 0.0021/K)
View Solution(a) Find the final temperature of a copper rod. Whose area of cross section changes from 10m2 to 11m2 due to heating. The copper rod is initially kept at 90K. (Coefficient of superficial expansion is 0.0021/K).
(b) The far position of a myopic person is 90 cm is front of the eye. what is the nature and power of the lens required to correct the problem?
View SolutionA copper rod and a steel rod maintain a difference in their lengths of 10 cm at all temperature . If their coefficients of expansion are 1.6×10−5K−1and1.2×10−5K−1 , then length of the copper rod is
View Solutionचित्र में दर्शाये गये निकाय की स्थायी अवस्था में स्टील तांबा संधि का माप क्या है? स्टील छड़ की लम्बाई =15.0cm तांबे की छड़ की लम्बाई =10.0cm भट्टी का ताप =300∘C, दूसरे सिरे का ताप =0∘C, स्टील की छा़ की अनुप्रस्थ काट का क्षेत्रफल तांबे की छड़ की अनुप्रस्थ काट के क्षेत्रफल का दोगुना है। स्टील की ऊष्मा चालकता =50.2JS−1m−1K−1, तांबे की ऊष्मा चालकता =385JS−1m−1K−1
View SolutionA Steel rod of length 25 cm has a cross - sectional area of 0.8cm2. The force required to stretch this rod by the same amount as the expansion produced by heating it through 10∘C is (coefficient of linear expansion of steel is 10−5/∘C and Young's modulus of steel is 2×1010N/m2).................... .
View SolutionThere is a change in length when a 33000 N tensile force is applied on a steel rod of area of cross - section 10^(-3) . The change of temperature required to produce the same elongation, if the steel rod is heated, is (modulus of elasticity of steel =3xx10^(11)N//m^(2) , coefficient of linear expansion of steel =1.1xx10^(-5)//^(@)C ........................
View Solution
