A parallel plate capacitor filled with mica having εr = 5 is connected to a 10V battery . The area of the parallel plate is 6m2 and separation distance is 6 mm.
(a) Find the capacitance and stored charge . (b) After the capacitor is fully charged the battery is disconnected and the dielectric is removed carefully .
Calculated the new values of capacitance stored energy and charge.Solution in Tamil
The capacitance of the capacitor in the presence of dielectric is
C=εrε0Ad=5×8.85×10−12×66×10−3
=44.25×10−9F=44.25nF
The stored charge is Q=CV= 44.25×10−9×10==44.2nC
The stored energy is U=12CV2=12×44.25×10−9×100=2.21×10−6J=2.21μJ
(b) After the removal of the dielectric since the battery is already disconnected the total charge will not change . But the potential difference between the the plates increases . As a result the capacitance is decreased .
New capacitance is C0=Cεr=44.25×10−95=8.85×10−9F=8.85nF
The stored charge remains same and 442.5 nC. Hence newly stored energy is
U0=Q22C0=Q2εr2C=εrU
=5×2.21μJ=11.05μJ
The increased energy is ΔU=11.05μJ−2.21μJ=8.84μJ
When the dielectric is removed it experience an inward pulling force due to the plates . To remove the dielectric an external agency has to do work on the dielectric which is stored as additional energy . This is the source for the extra energy 8.84μJ
Topper's Solved these Questions
- ELECTROSTATICS BOOK - FULL MARKSCHAPTER - ELECTROSTATICS EXERCISE - Textual Evaluation Solved (Multiple choice questions : )
15 Videos
- ELECTROSTATICS BOOK - FULL MARKSCHAPTER - ELECTROSTATICS EXERCISE - Textual Evaluation Solved (II. Short Answer Questions : )
21 Videos
- DUAL NATURE OF RADIATION AND MATTERBOOK - FULL MARKSCHAPTER - DUAL NATURE OF RADIATION AND MATTEREXERCISE - ADDITIONAL QUESTIONS -( ADDITIONAL NUMERICAL PROBLEMS : )
10 Videos
- MAGNETISM AND MAGNETIC EFFECTS OF ELECTRIC CURRENT BOOK - FULL MARKSCHAPTER - MAGNETISM AND MAGNETIC EFFECTS OF ELECTRIC CURRENT EXERCISE - ADDITIONAL QUESTIONS SOLVED - NUMERICAL PROBLEMS :
4 Videos
Similar Questions
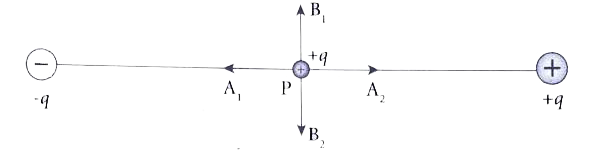
Two identical point charges'of magnitude -q are fixed as shown in the figure below. A third charge +q is placed midway between the two charges at the point P. Suppose this charge is displaced a small distance from the point P in the directions indicated by the arrows, in which direction(s) will +q be stable with respect to the displacement?
View SolutionWhat is meant by quantisation of charges ?
View SolutionIf a light of wavelength 4950 Å is viewed as a continuous flow of photons, what is the energy of each photon in eV ? (Given h=6.6×10−34Js, c =3×108ms−1)
View SolutionThe radius of the first orbit of hydrogen atom is 0.5 Å. The electron moves is an orbit with a uniform speed of 2.2×106ms−1. What is the magnetic field produced at the centre of the uncleus due to the mation of this electron ? Use μ0=4π×10−7Hm−1 and electric charge =1.6×10−19 C
View SolutionA parallel capacitor of capacitance C is charged and disconnected from the battery. The energy stored in it is E. If a dielectric slab of dielectric constant 6 is inserted between the plates of the capacitor then energy and capacitance will become.
View SolutionThe plates of a parallel plate capacitor have an area of 100cm^(2) each and area separated by 2.5 mm. the capacitor is charged to 200V. Calculate the energy stored in the capacitor.
View Solutionएक समांतर-प्लेट संधारित्र को बैटरी से आवेदित किया जाता है। बैटरी का संबंध संधारित्र से विच्छेदित करने के उपरांत प्लेटो के बीच की दूरी दोगुनी करने पर संधारित्र की (i) धारिता तथा (ii) संग्रहित ऊर्जा पर के प्रभाव पड़ेगा?
View SolutionA capacitor of capacitance C is charged fully by connecting it to a battery of emf B. It is then disconnected from the battery. If the separation between the plates of the capacitor is now doubled, how will the following change ?
charge stored by the capacitor.
View SolutionA capacitor of capacitance C is charged fully by connecting it to a battery of emf B. It is then disconnected from the battery. If the separation between the plates of the capacitor is now doubled, how will the following change ? Energy stored by the capacitor.
View Solutionएक समान्तर प्लेट संधारित्र की प्रत्येक प्लेट का क्षेत्रफल 100 सेमी2 है तथा प्लेटों के बीच दूरी 1 मिमी है। प्लेट अभ्रक की है जिसका परावैद्युतांक 6 है। इस संधारित्र की धारिता ज्ञात कीजिए । यदि ε0=8.86×10−12 फैरड/मीटर है ?
View Solutionएक समान्तर प्लेट संधारित्र की धारिता 100 pF है । 500 V तक इसे आवेशित किया जाता है। आवेशन के पश्चात् (after charging) बैटरी हटाकर प्लेटों के बीच की दूरी आधी कर दी जाती है। संधारित्र पर नया विभवान्तर तथा संचित ऊर्जा (stored energy) ज्ञात कीजिए।
View SolutionA parallel plate capacitor has plates of area 4 m^2 separated by distance of 0.5 mm. The capacitor is connected across a cell of emf 100 V. Find the capacitance, charge and energy stored in the capacitor.
View SolutionIn a circular parallel plate capacitor radius of each plate is 5 cm and they are separated by a distance of 2 mm. Calculate the capacitance and the energy stored, when it is charged by connecting the battery of 200 V.
View SolutionA parallel plate capacitor has square plate of side 5 cm and separated by a distance of 1 mm . (a) Calculate the capacitance of this capacitor . (b) If a 10 V battery is connected to the capacitor what is the charge stored in any one of the plates? ( The value of epsilon_(0)= 8.85 xx10^(-12)Nm^(2)C^(-2)).
View SolutionA parallel plate capacitor is charged by a battery . After sometime the battery is disconnected and a dielectric slab with its thickness equal to the plate separation is inserted between the plates . How will the energy stored in the capacitor be affected ? Justify your answer
View Solution
