Consider four equal charges q1,q2,q3 and q4=q=+1μC located at four different points on a circle of radius 1m , as shown in the figure. Calculate the total force acting on the charge q1 due to all the other charges .
 Solution in Tamil
Solution in Tamil
According to the superposition principle the total electrostatic force on charge q1 is the vector sum of the forces due to the other charges,
→Ftot1=→F12+→F13+→F14
The following diagram shows the direction of each force on the charge q1
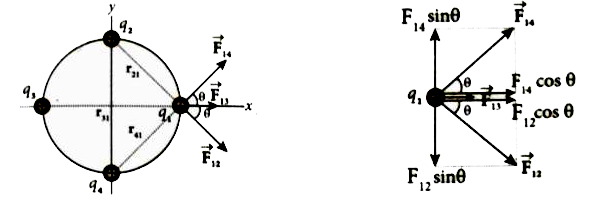
The charges q2 and q4 are equi-distant from q1 . As a result the strengths ( magnitude ) of the forces →F12 and →F14 are the same even though their directions are different . Therefore the vectors representing these two forece are drawn with equal length . But the charge q3 is located farther compared to q2 and q4 . Since the strength of the electrostatic force decreases as distance increases the strength of the force →F13 is drawn with smaller length compared to that for forces →F12 and →F14 .
From the figure r21=√2m=r41and r31=2m
The m agnitudes of the forces are given by
F13=kq2r331=9×109×10−124=2.25×10−3N
F12=kq2r221=F14=9×109×10−122=4.5×10−3N
From the figure the angle θ=45∘ . In terms of the components we have
→F12=F12cosθˆi−F12sinθˆj=4.5×10−3×1√2ˆi−4.5×10−3×1√2ˆj
→F13=F13ˆi=2.25×10−3Nˆi
→F14=F14cosθˆi+F14sinθˆj=4.5×103×1√2ˆi+4.5×10−3×1√2ˆj
Then the total force on q1 is
→Ftot1=(F12cosθˆi−F12sinθˆj)+F13ˆi+(F14cosθˆi+F14sinθˆj)
→Ftot1=(F12cosθ+F13+F14cosθ)ˆi+(−F12sinθ+F14sinθ)ˆj
Since F12=F14 the jth component is zero . Hence we have
→Ftot1=(F12cosθ+F13+F14cosθ)ˆi
Substituting the values in teh above equation
=4.5√2+2.25+4.5√2ˆi=(4.5√2+2.25)ˆi
→Ftot1=8.61×10−3Nˆi
The resultant force is along the positive x axis .
Topper's Solved these Questions
- ELECTROSTATICS BOOK - FULL MARKSCHAPTER - ELECTROSTATICS EXERCISE - Textual Evaluation Solved (Multiple choice questions : )
15 Videos
- ELECTROSTATICS BOOK - FULL MARKSCHAPTER - ELECTROSTATICS EXERCISE - Textual Evaluation Solved (II. Short Answer Questions : )
21 Videos
- DUAL NATURE OF RADIATION AND MATTERBOOK - FULL MARKSCHAPTER - DUAL NATURE OF RADIATION AND MATTEREXERCISE - ADDITIONAL QUESTIONS -( ADDITIONAL NUMERICAL PROBLEMS : )
10 Videos
- MAGNETISM AND MAGNETIC EFFECTS OF ELECTRIC CURRENT BOOK - FULL MARKSCHAPTER - MAGNETISM AND MAGNETIC EFFECTS OF ELECTRIC CURRENT EXERCISE - ADDITIONAL QUESTIONS SOLVED - NUMERICAL PROBLEMS :
4 Videos
Similar Questions
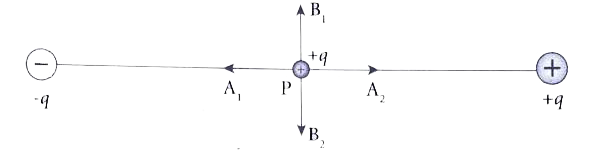
Two identical point charges'of magnitude -q are fixed as shown in the figure below. A third charge +q is placed midway between the two charges at the point P. Suppose this charge is displaced a small distance from the point P in the directions indicated by the arrows, in which direction(s) will +q be stable with respect to the displacement?
View SolutionWhat is meant by quantisation of charges ?
View SolutionIf a light of wavelength 4950 Å is viewed as a continuous flow of photons, what is the energy of each photon in eV ? (Given h=6.6×10−34Js, c =3×108ms−1)
View SolutionThe radius of the first orbit of hydrogen atom is 0.5 Å. The electron moves is an orbit with a uniform speed of 2.2×106ms−1. What is the magnetic field produced at the centre of the uncleus due to the mation of this electron ? Use μ0=4π×10−7Hm−1 and electric charge =1.6×10−19 C
View SolutionThree charges -q_(1), +q_(2) and -q_(3) are placed as shown in the figure. The x -component of the force on -q_(1) is proportional to
View SolutionTwo large , parallel conducting plates X and Y , kept close to each other , are given Q1 and Q2(Q1>Q2). The four surfaces of the plates are A,B,C and D , as shown
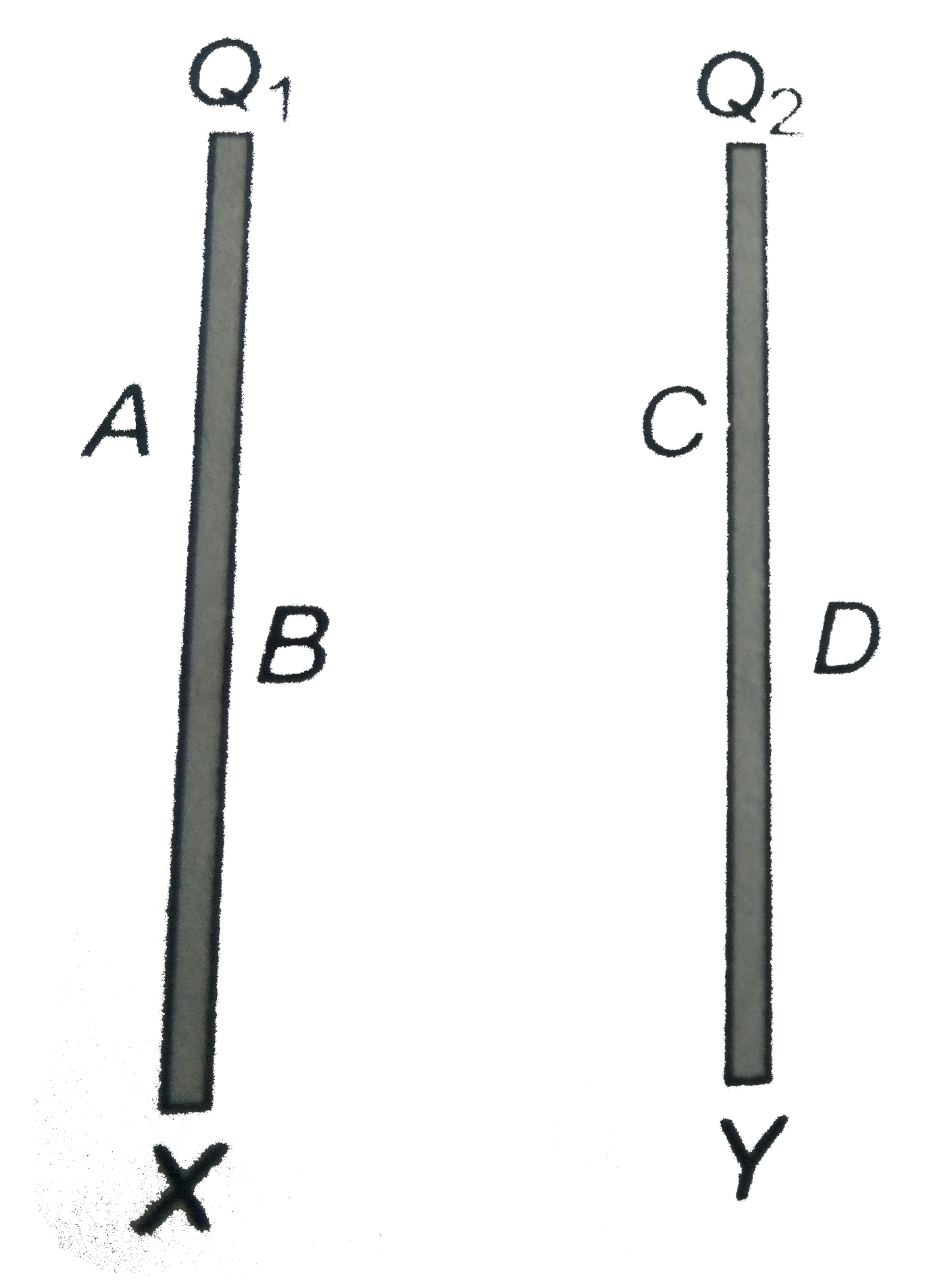
(i) The charge on A is 12(Q1+Q2)
(ii) The charge on B is 12(Q1−Q2)
(iii) The charge on C is 12(Q2−Q1)
(iv) The charge on D is 12(Q1+Q2)
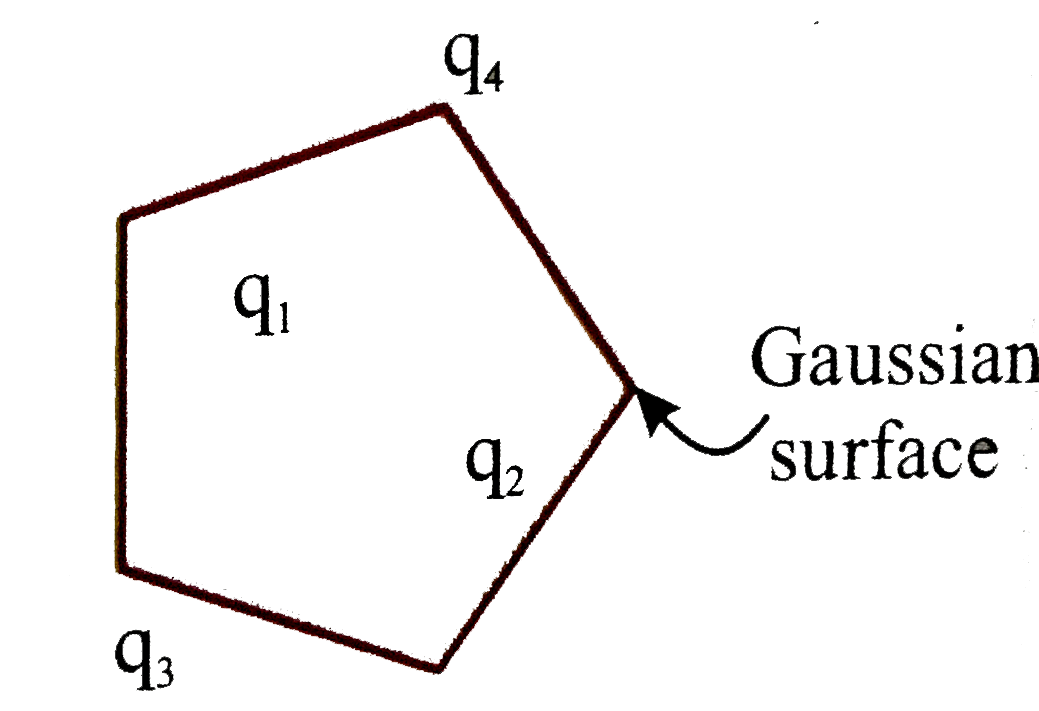
View SolutionAssertion: Four point charges q1,q2,q3 and q4 are as shown in Fig,The flux over the shown Gaussians surface depends only on charges q1 and q2
Reason:Electric field at all points on Gaussians surface depends on any charges q1 and q2
View SolutionThree charges q_(1) = 1 mu C, q_(2) = -2 muC and q_(3) = 3mu C are placed on the vertices of an equilateral triangle of side 1.0 m. find the net electric force acting on charge q_(1) . How to proceed Charge q_(2) will attract charge q_(1) (along the line joining them) and charge q_(3) will repel charge q_(1) . Therefore, two forces will act on q_(1) , one due to q_(2) and another due to q_(3) . Since , the force is a vector quantity both of these force (say F_(1) and F_(2) ) will be added by vector method. Following are two methods of their addition
View SolutionA charge q1 exerts some force on a second charge q2 If a third charge q3 is brought near q2, then the force exterted by q1 on q2
View SolutionRepresent the resultant force acting on a charge q0 due to point charges q1,q2,q3,q4. Assume the position vectors as →r0,→r1,→r2,→r3,→r4 respectively. Give the expression for net force on q0
View SolutionThree charges −q1,+q2 and −q3 are placed as shown in the figure. The x-component of the force on −q1 is proportional to

View Solutionचित्र 2.52 में सभी चार आवेशों का परिमाण एक जैसा है। बिन्दु P पर इन द्वारा उत्पन्न वैद्युत क्षेत्र बाईं दिशा में हैं। आवेश q1 ऋणात्मक है। आवेशों q2,q3 और q4, के क्या चिह्न हैं?
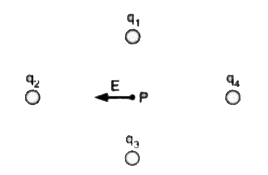
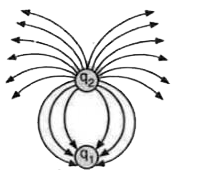
View Solutionचित्र 2.58 में बिन्दु आवेश q1 और q2 की वैद्युत क्षेत्र रेखाएँ दिखाई गई है। q1 और q2 के क्या-क्या चिह्न हैं? यदि रेखाएँ आवेश के अनुपात में खींची गईं हों, तो अनुपात q1/q2 क्या है?
View SolutionThree charges (-q1),(+q_(2)) And (-q_(3)) Are placed as shown in Figure I.1. Charge (-q_(1)) The x-component of the force is proportional to
View Solutionचार आवेश q1=1μC,q2=2μC,q3=−3μC तथा q4=4μC,1 मी भुजा के वर्ग के शीर्षो पर रखे है। आवेशों के इस निकाय की वैद्युत स्थितिज ऊर्जा ज्ञात कीजिए।
View Solution
