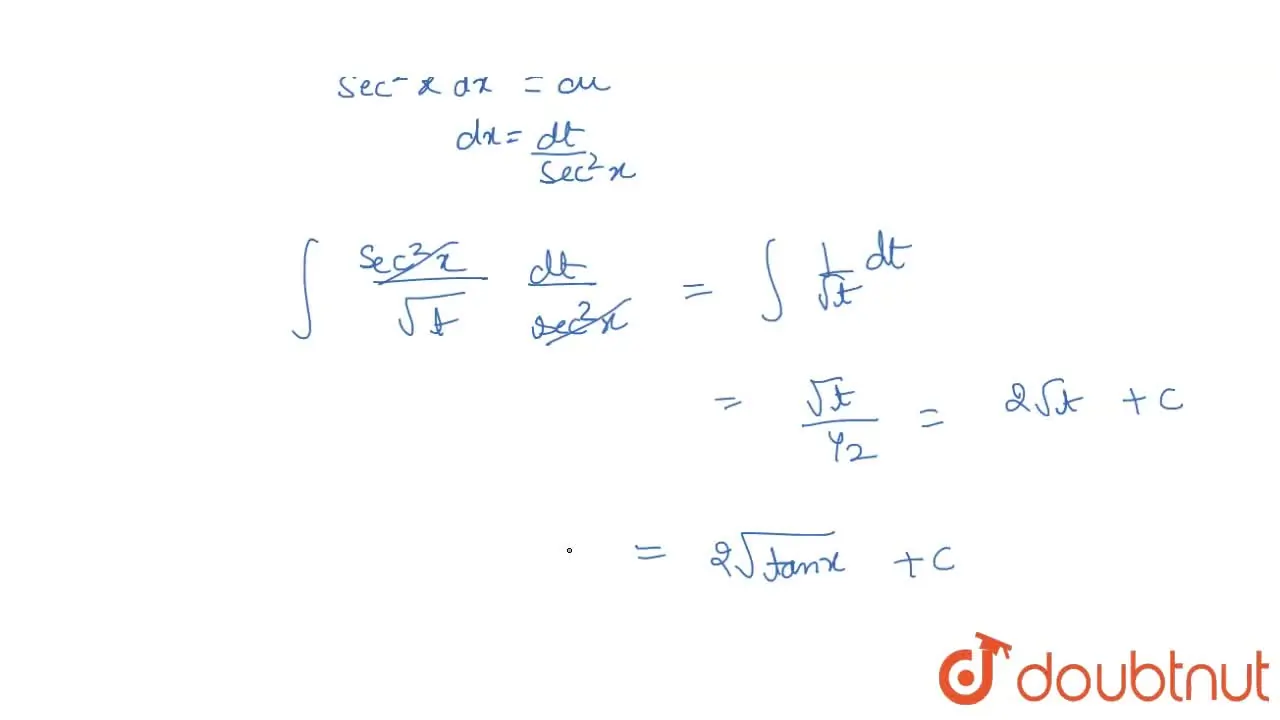
∫sec2x√tanxdx
Video Solution
Text Solution
The correct Answer is:2√tanx+c
|
Answer
Step by step video, text & image solution for int(sec^(2)x)/(sqrt(tanx))dx by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023
Related Playlists
- BOOK - NAGEEN PRAKASHANCHAPTER - INTEGRATIONEXERCISE - Exercise 7f24videos
INTEGRATION
- BOOK - NAGEEN PRAKASHANCHAPTER - INTEGRATIONEXERCISE - Exercise 7g31videos
INTEGRATION
- BOOK - NAGEEN PRAKASHANCHAPTER - INTEGRATIONEXERCISE - Exercise 7d38videos
INTEGRATION
- BOOK - NAGEEN PRAKASHANCHAPTER - DIFFERENTIAL EQUATIONSEXERCISE - Miscellaneous Exercise18videos
DIFFERENTIAL EQUATIONS
- BOOK - NAGEEN PRAKASHANCHAPTER - INVERES TRIGONOMETRIC FUNCTIONSEXERCISE - Miscellaneous Exercise (prove That )9videos
INVERES TRIGONOMETRIC FUNCTIONS
Similar Questions
∫sec2x√1+tanx dx
View SolutionIntegrate : ∫sec2x√1+tanxdx
View Solution∫sec2x(1+tanx)dx
View Solution∫sec2x√1−tan2xdx=?
View SolutionEvaluate: ∫sec2x1+tanxdx
View SolutionEvaluate: ∫sec2√x√xdx
View SolutionEvaluate: ∫sec2√x√x dx
View Solution∫sec2x+1x+tanxdx
View SolutionEvaluate: (i) ∫sec2√x√x dx (ii) ∫tan32xsec2x dx
View Solution∫tan4√xsec2√x√xdx
View Solution