The ratio in which the line $x - 1 = 0$ divides the area bounded by the curves $2 x + 1 = \sqrt{4 y + 1}, y = x and y = 2$ is

Video Solution

Text Solution

Verified by Experts

$We have 2 x + 1 = \sqrt{4 y + 1} ...(1)$
$or {(2 x + 1)}^{2} = 4 y + 1$
$or y = x^{2} + x ...(2)$
$∴ Curve (1) is part of the parabola (2), for x \geq - 1 / 2$ .
Differentiating (2), w.r.t. x, we get
$\frac{d y}{d x} = 2 x + 1$
$∴ {(\frac{d y}{d x})}_{x = 0} = 1 .$
$∴$ line y=x is tangent to curve (2) at (0,0).

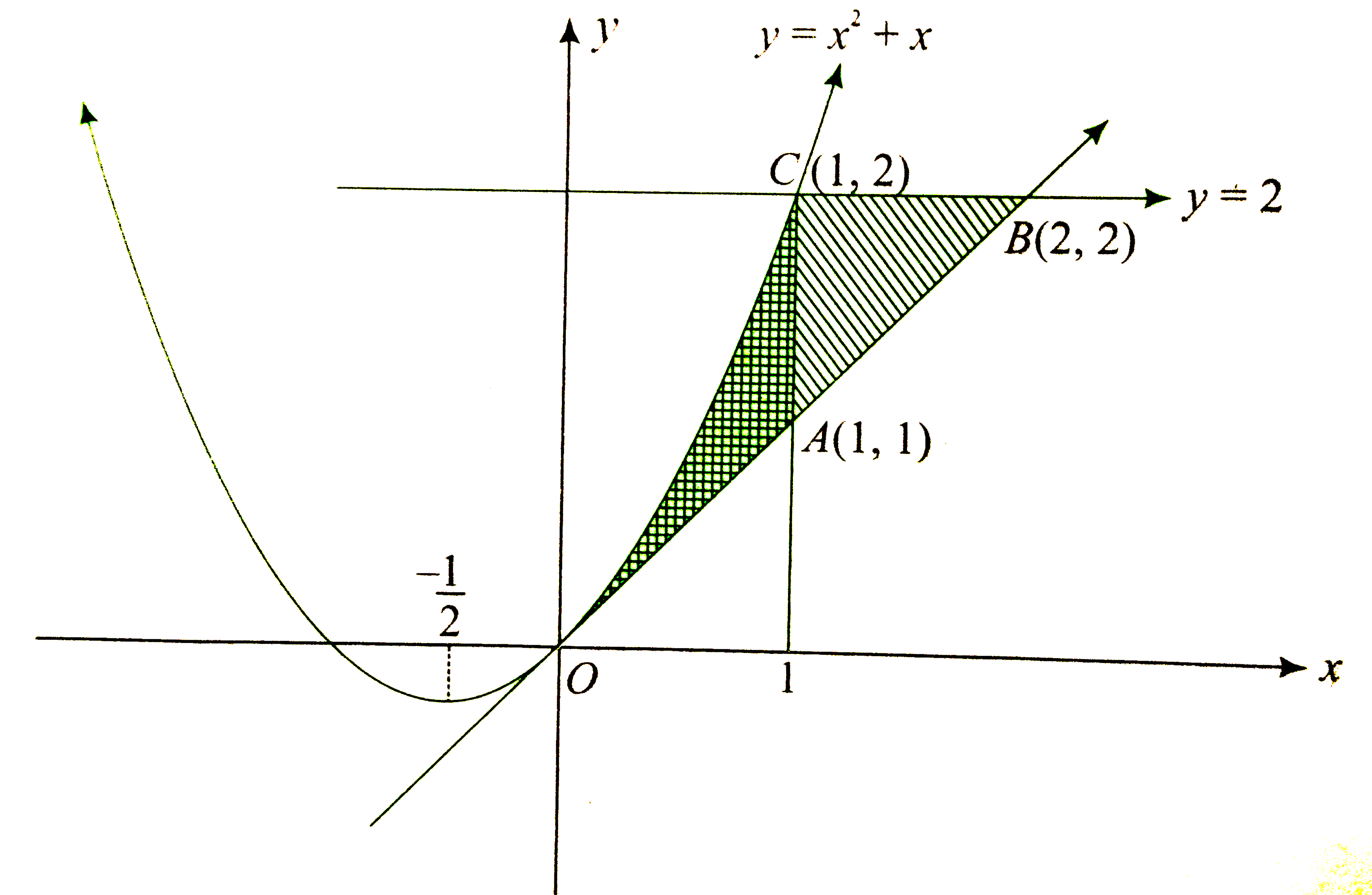
From the figure,
$Area of the region O A C O i s = 1 \int 0 (x^{2} + x - x) d x$
$= {[\frac{x^{3}}{3}]}_{0}^{1} = \frac{1}{3}$
$Area of the triangle A C B i s = \frac{1}{2} \times 1 \times 1 = \frac{1}{2}$
$∴ Required ratio = \frac{1 / 3}{1 / 2} = \frac{2}{3}$

Updated on:21/07/2023

The area bounded by the curves $y = \sqrt{5 - x^{2}}$ and $y = | x - 1 |$ is
A $(\frac{5 π}{4} - 2)$ sq units
B $(\frac{5 π - 2}{4})$ sq units
C $(\frac{5 π - 2}{2})$ sq units
D $(\frac{π}{2} - 5)$ sq units
Consider the curves $C_{1} = y - 4 x + x^{2} = 0$ and $C_{2} = y - x^{2} + x = 0$
The raio in which the line y = x divide the area of the region bounded by the curves $C_{1} = 0$ and $C_{2} = 0$ is
A $\frac{93}{32}$
B $\frac{91}{16}$
C $\frac{91}{4}$
D $\frac{93}{16}$
Consider the curves $C_{1} = y - 4 x + x^{2} = 0$ and $C_{2} = y - x^{2} + x = 0$
The ratio in which the x-axis divide the area of the region bounded by the curves $C_{1} = 0$ and $C_{2} = 0$ is
A $\frac{125}{121}$
B $\frac{121}{4}$
C $\frac{21}{125}$
D $\frac{121}{6}$