Find the area bounded by
(i) y $= {log}_{e} | x | and y = 0$
(ii) $y = | {log}_{e} | x | | and y = 0$

Video Solution

Text Solution

Verified by Experts

(i) y $= {log}_{e} | x | and y = 0$

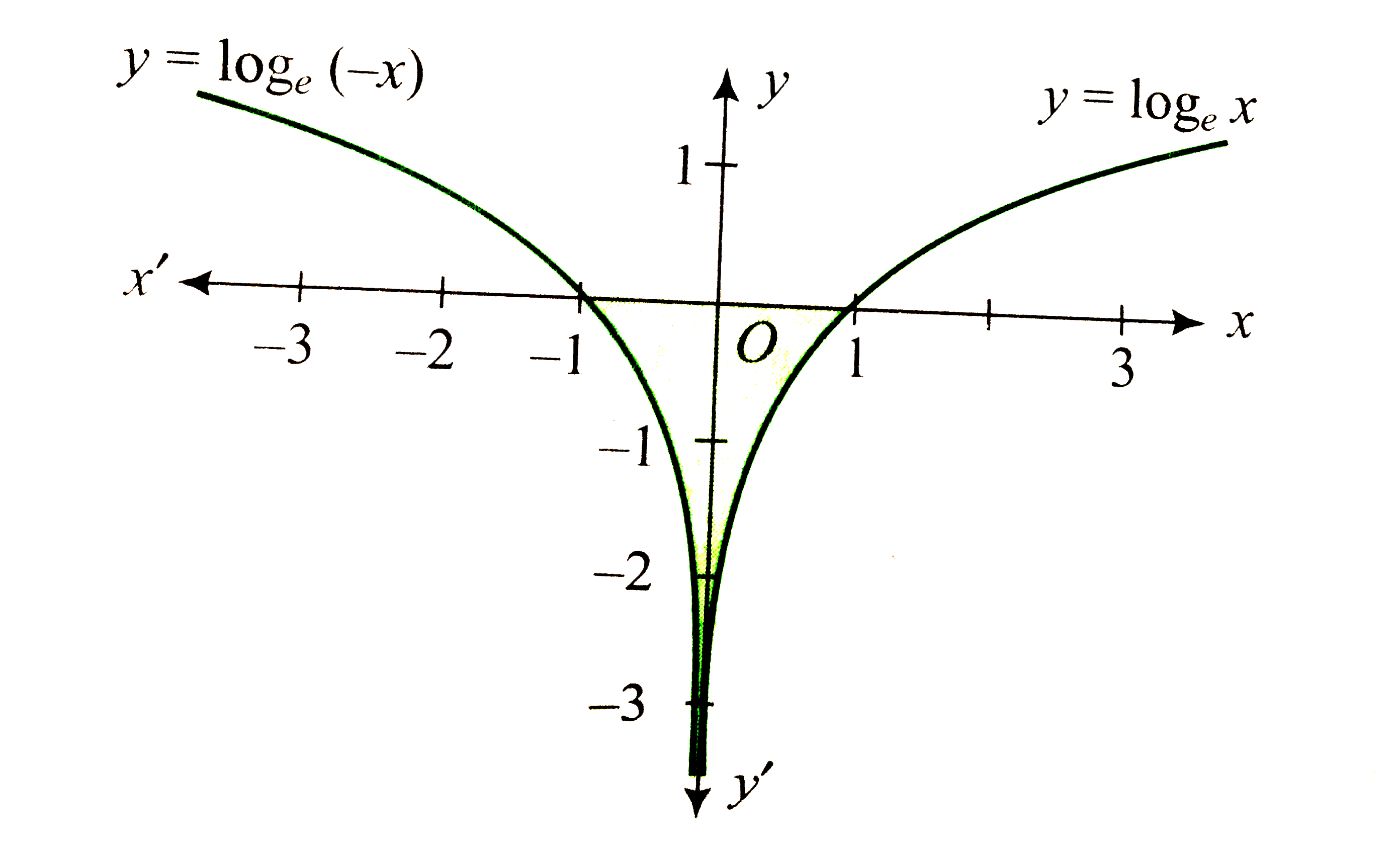
From the figure, required area = area of the shaded region = 1 + 1 =2 sq. units (as we know that area bounded by $y = {log}_{e} x, x = 0 and y = 0$ is 1 sq. units)
(ii) $y = | {log}_{e} | x | | and y = 0$

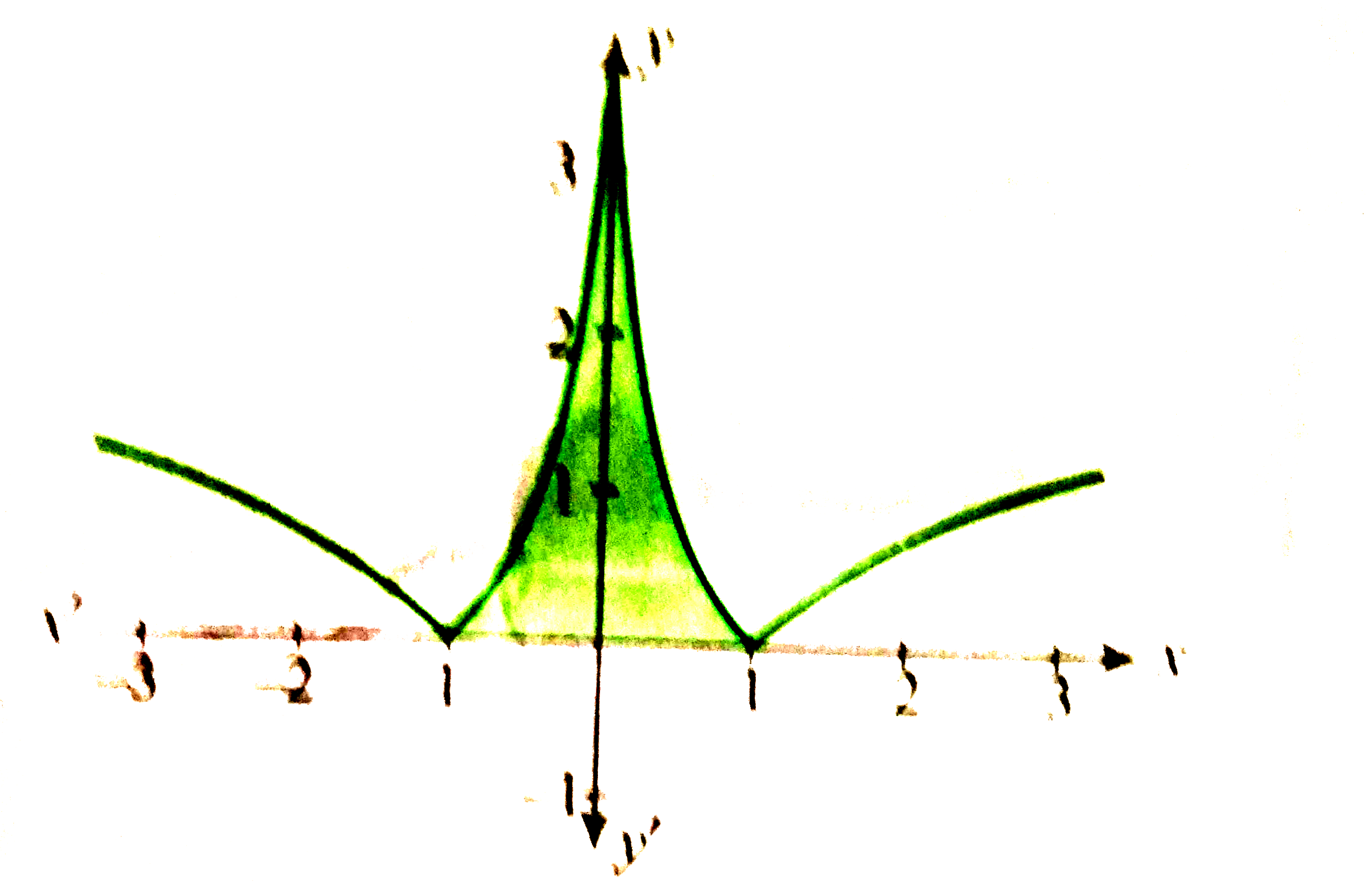
From the figure,
Required area = Area of the shaded region
= 1 + 1 =2 sq. units.

Updated on:21/07/2023

|

Class 12 MATHS APPLICATION OF INTEGRALS

Topper's Solved these Questions

Similar Questions

Knowledge Check

The area bounded by the curve $y = x (1 - {log}_{e} x)$ and x-axis is
A $\frac{e^{2}}{4}$
B $\frac{e^{2}}{2}$
C $\frac{e^{2} - e}{2}$
D $\frac{e^{2} - e}{4}$
Area bounded by $y = log (x - 2)$ , x - axis and x = 4 is
A $2 log 2 + 1$
B $log 2 - 1$
C $log 2 + 1$
D $2 log 2 - 1$

CENGAGE-AREA-Comprehension Type

Home
Ask Doubt
Profile