Let be a function Then find the nature of each of the following functions.
(i)
(iii)
(i) .
Graph of the function is as shown in the following figure.
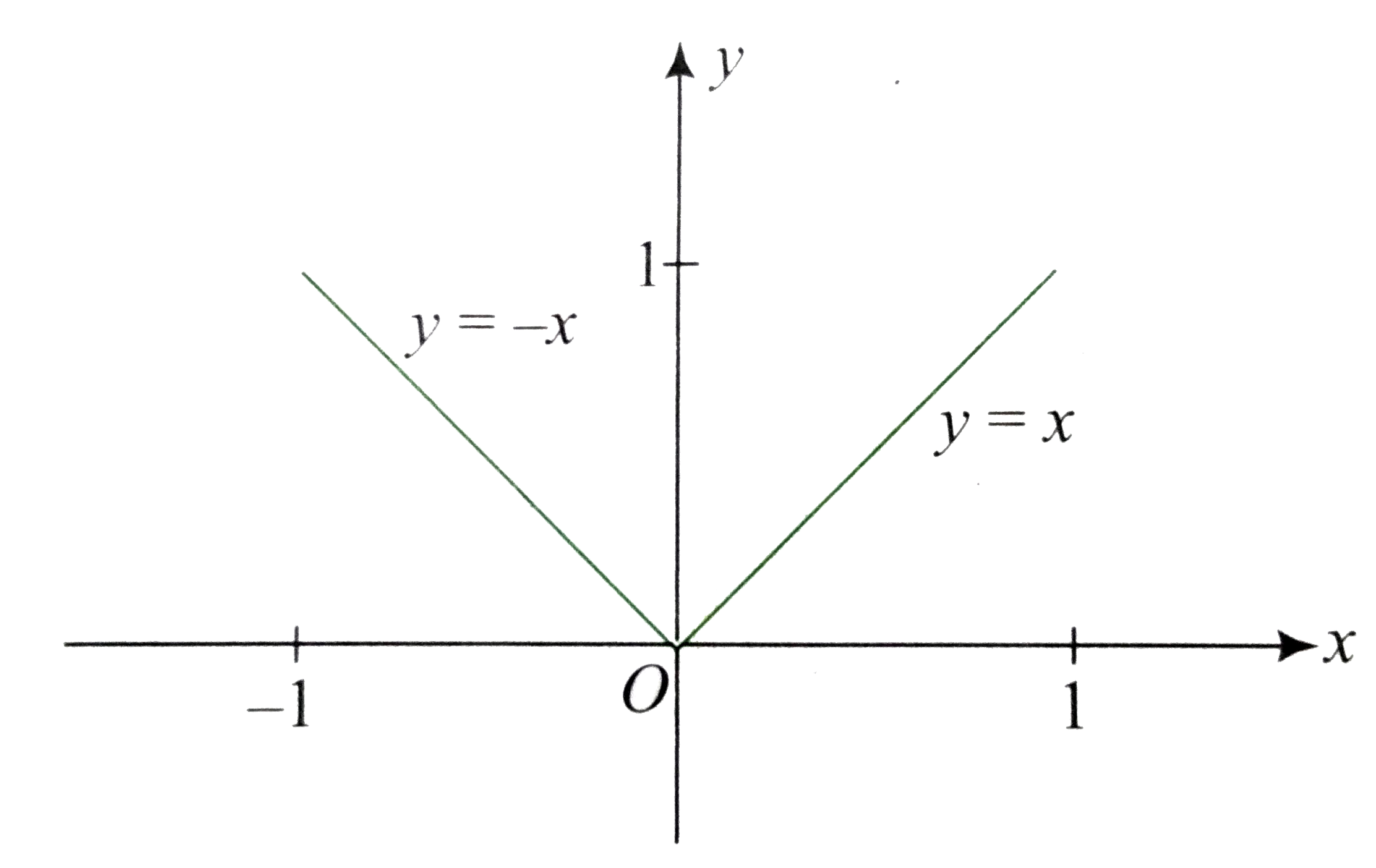
From the graph we observe that f(x) is many-one.
Also, we observe that the range is . Hence f(x) is into.
(ii) where
Now
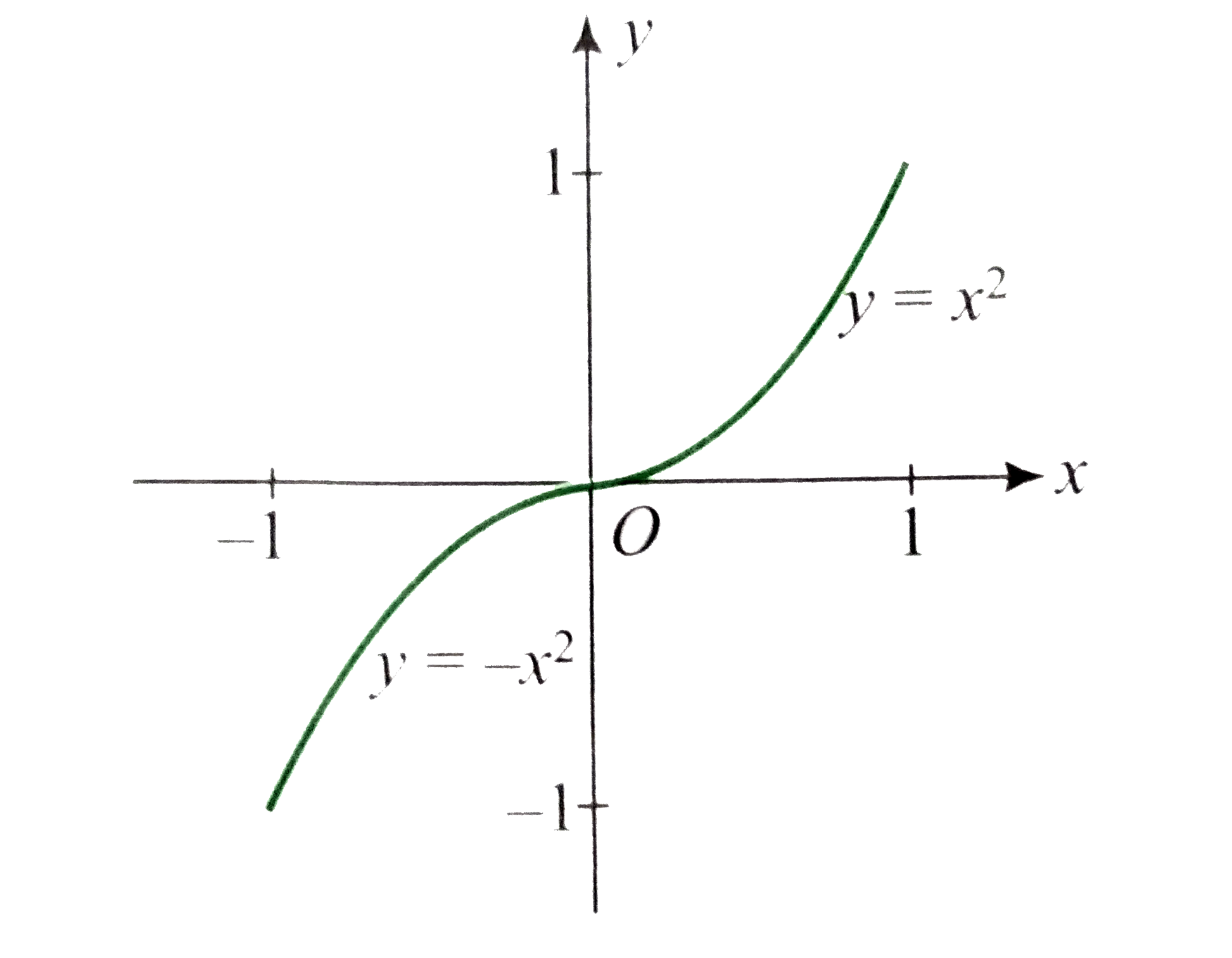
From the graph we observe that f(x) is one-one as there is no horizontal line which intersect the curve more than once.
Also, we observe that the range is . Hence f(x) is onto.
Thus f(x) is bijective.
(iii)
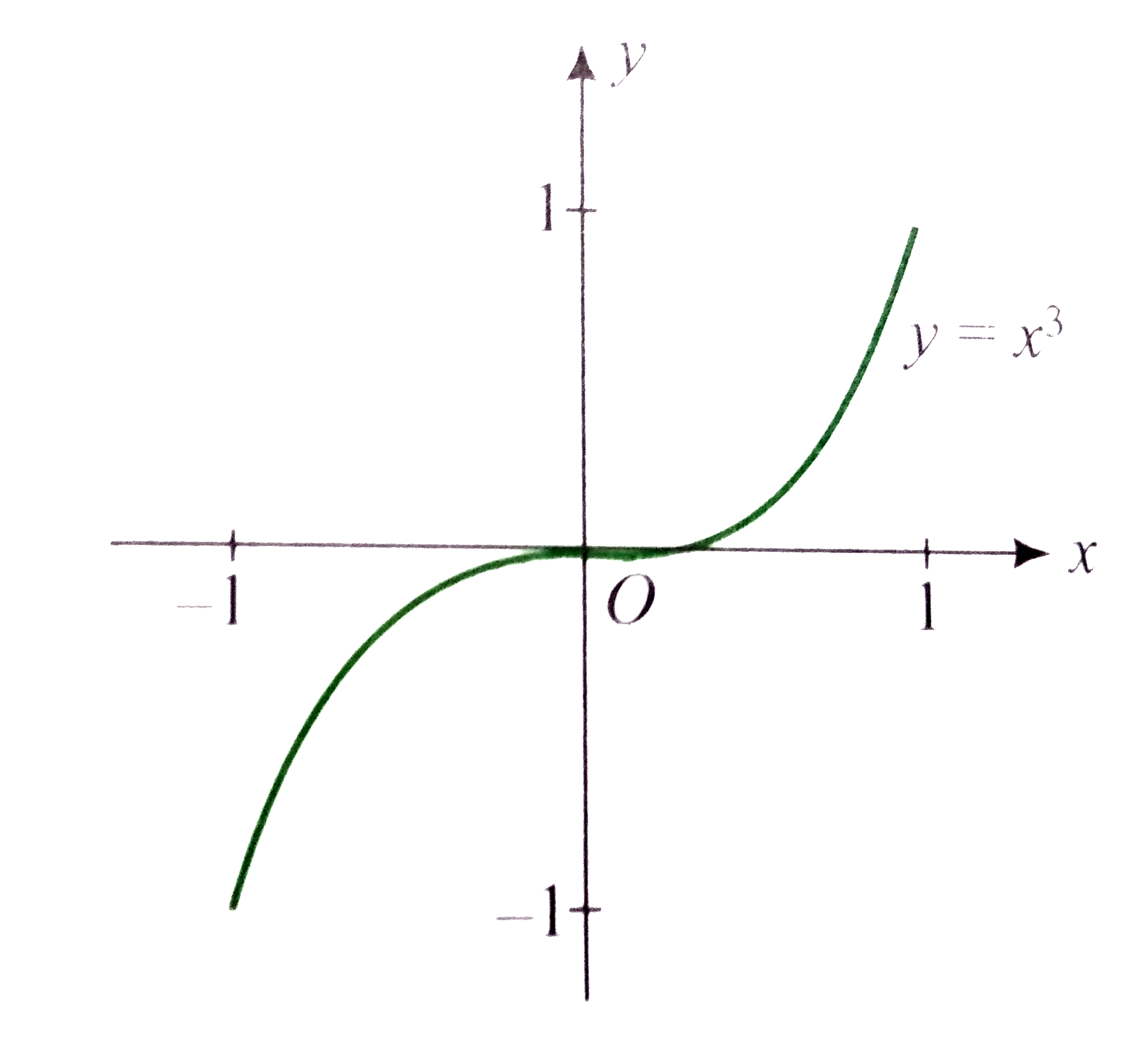
Clearly, f(x) is one-one.
Also, for
So, f(x) is onto.
Thus, f(x) is bijective.
(iv)
For
So, graph of f(x) is as shown in the following figure.
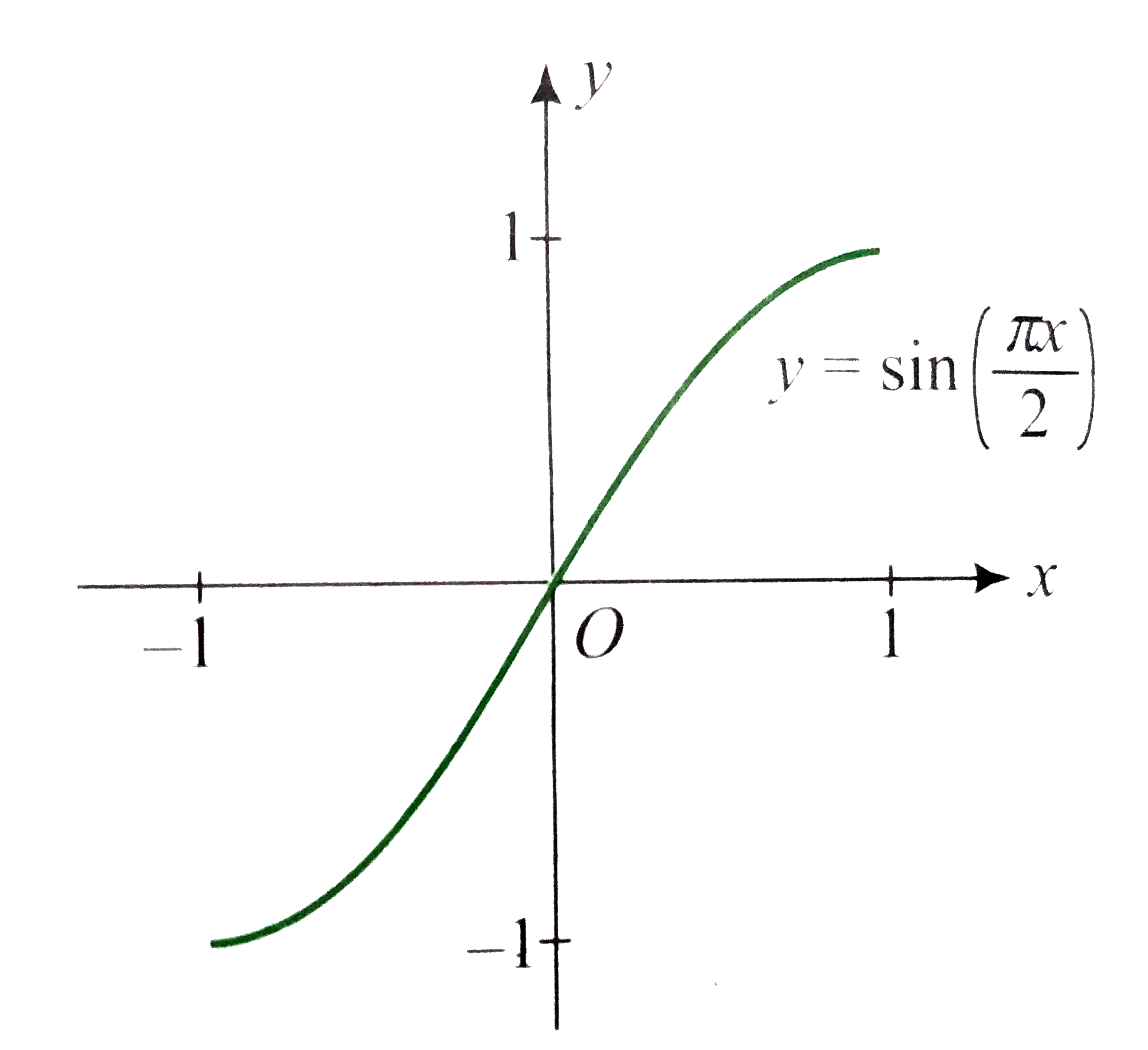
From the graph we observe that f(x) is one-one.
Also, range of the f(x) is Hence f(x) is onto.
Thus, f(x) is bijective.
Topper's Solved these Questions
- RELATIONS AND FUNCTIONSBOOK - CENGAGECHAPTER - RELATIONS AND FUNCTIONSEXERCISE - Exercise 1.1
15 Videos
- RELATIONS AND FUNCTIONSBOOK - CENGAGECHAPTER - RELATIONS AND FUNCTIONSEXERCISE - Exercise 1.2
5 Videos
- Quadratic Equations, Inequalities, Modulus and LogarithmsBOOK - CENGAGECHAPTER - Quadratic Equations, Inequalities, Modulus and LogarithmsEXERCISE - Question Bank
31 Videos
- SCALER TRIPLE PRODUCTS BOOK - CENGAGECHAPTER - SCALER TRIPLE PRODUCTS EXERCISE - DPP 2.3
11 Videos
Similar Questions
Knowledge Check
The function f(x)=cosx,0≤x≤π is
AdecreasingBincreasingCneither increasing nor decreasingDincreasing for 0≤x≤π
Find the domain of each of the following functions:
(i) f(x)=sin−1x2
(ii) f(x)=sin−1x+sinx
View SolutionFind the domain and range of the following functions:
(i) f(x)=√(x−1)(3−x)
(ii) f(x)=11−7sinx
(iii) f(x)=1−|x|
View SolutionFind the range of the following functions
(i) f(x)=log2(√x−4+√6−x),4≤x≤6
(ii) f(x)=9x−3x+1
View SolutionFind the intervals of monotonicity of the following functions.
(i)f(x)=−x3+6x2−gx−2
(ii)f(x)=x+1x+1
(iii) f(x)=x.ex−x2
(iv) f(x)=x−cosx
View SolutionFind the domain of each of the following functions given by f(x)=1√|x|−x
View SolutionFind the points of local maximinima of following functions
(i)f(x)=(2'−1)(2'−2)2 (ii)f(x)=−(x−1)3(x+1)2
(iii)f(x)=xenx
View SolutionIf f(x) is a real valued function, then which of the following is injective function ?
(i) f(x)=x5 (ii) f(x)=x+sinx
(iii) f(x)=x2 (iv) f(x)=ex
(v) f(x)=x3+x2+4x+4
View Solution
