Solve: −1|x|−2≥1.
Video Solution
Text Solution
Given, −1|x|−2≥1
⇒−1|x|−2−1≥1
⇒−1−(|x|−2)|x|−2≥0
⇒1−|x||x|−2≥0
⇒|x|−1|x|−2≤0
⇒y−1y−2≤0, where y=|x|
⇒1≤y<2
⇒1≤|x|<2
⇒x∈(−2,−1]∪[1,2)
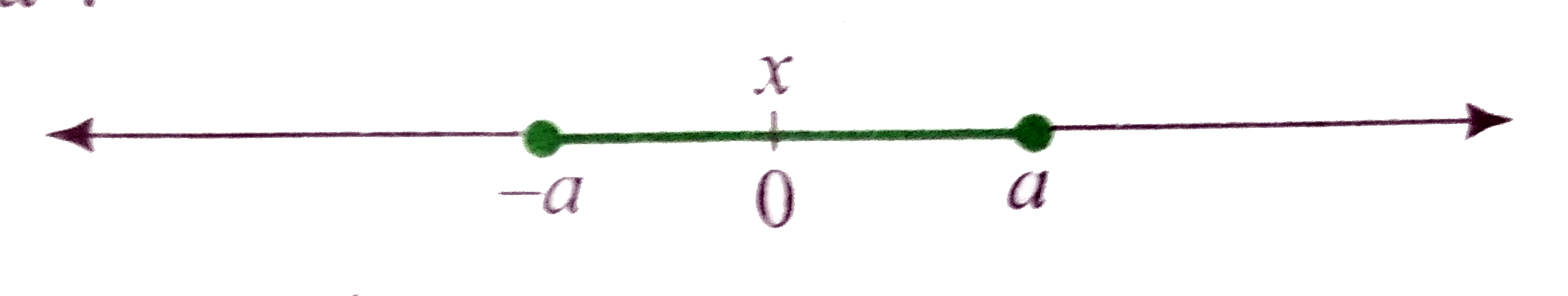
|
Topper's Solved these Questions
- RELATIONS AND FUNCTIONSBOOK - CENGAGECHAPTER - RELATIONS AND FUNCTIONSEXERCISE - Exercise 1.1
15 Videos
- RELATIONS AND FUNCTIONSBOOK - CENGAGECHAPTER - RELATIONS AND FUNCTIONSEXERCISE - Exercise 1.2
5 Videos
- Quadratic Equations, Inequalities, Modulus and LogarithmsBOOK - CENGAGECHAPTER - Quadratic Equations, Inequalities, Modulus and LogarithmsEXERCISE - Question Bank
31 Videos
- SCALER TRIPLE PRODUCTS BOOK - CENGAGECHAPTER - SCALER TRIPLE PRODUCTS EXERCISE - DPP 2.3
11 Videos
Similar Questions
Knowledge Check
Solve : 2x+3≥0
A(2,3)B(5,∞)C(3,∞)D(−3,∞)
Solve the following systems of inequalities for all x∈R
Solve (|x|−1)(|x|−2)≥0,x∈R,x is not equal to ±2
View SolutionSolve:
12−|x|≥1,x∈R−{−2,2}
View SolutionSolve log1−x(x−2)≥−1.
View SolutionSolve:
x−7x−2≥0,x∈R
View SolutionSolve : |x|−|x−2|≥1
View SolutionSolve ∣∣∣1−|x|1+|x|∣∣∣≥12
View Solution4xx2+3≥1.
View Solution
