A light source emitting three wavelength 5000Å,6000Å,7000Å has a total power of 10−3 m. W and the beam of diameter 2×10−3m. The power density is distributed equally amongst the three wavelengths. The beam shines normally on a metallic surface of area 104m2 which has a work function of 1.9 eV. Assuming that each proton liberates an electron, calculate the charge emitted per unit area in one second : Solution in Kannada
94 C
54 C
47 C
74 C
The correct Answer is:A
The threshold wavelngth λ0 of metallic surface is given by
w=hcλ0∴Λ0=6513Å
For photoelectric emission λ<λ0, so cut of the given
wavelength λ1=5000Åandλ2=6000Å will cause photo emission.
Power of source =10−3W
Diameter of beam D=2×10−3m
Radius of beam r=10−3m
∴ Area of cross section of beam
A=πr2=π×106m2
Power density of beam =PA
=10−3π×10−6=1π×103W/m3
Power density of each wavelength,
I.=1033πW/m3
Energy of photon of wavelength λ1
E1=hcλ1=3.97×10−19J
Number of photons of wavelength λ1 incident on metallic surface per unit area per sec.
N1=IE1=1033π×3.97×10−19=2.67×1020
Energy of photon of wavelength λ2
E2=hcλ2=3.31×10−19J
Then N2=I.E2=3.21×1020
As each photon liberates an electron, the number of electrons emitted per sec unit area
N=N1+N2
=2.67×1020+3.21×1020
=5.88×1020
∴ Charge emitted per sec. per unit area is
q=Ne
=5.88×1020×1.6×10−19
orq=94C
Topper's Solved these Questions
- BOOK - MODERN PUBLICATIONCHAPTER - ELECTRONS & PHOTONSEXERCISE - MULTIPLE CHOICE QUESTIONS (LEVEL-III)9videos
ELECTRONS & PHOTONS
- BOOK - MODERN PUBLICATIONCHAPTER - ELECTRONS & PHOTONSEXERCISE - RECENT COMPETITIVE QUESTIONS20videos
ELECTRONS & PHOTONS
- BOOK - MODERN PUBLICATIONCHAPTER - ELECTRONS & PHOTONSEXERCISE - RECENT COMPETITIVE QUESTIONS20videos
ELECTRONS & PHOTONS
- BOOK - MODERN PUBLICATIONCHAPTER - ELECTROMAGNETIC WAVESEXERCISE - RECENT COMPETITIVE QUESTIONS4videos
ELECTROMAGNETIC WAVES
- BOOK - MODERN PUBLICATIONCHAPTER - ELECTROSTATICSEXERCISE - RECENT COMPETITIVE QUESTIONS39videos
ELECTROSTATICS
Similar Questions
Energy required for the electron excitation in Li++ from the first to the third Bohr orbit is :
04:39View SolutionAccording to Einstein's photoelectric equation the graph of K.E. of the photoelectron emitted from the metal versus the frequency of the incident radiation gives a straight line graph whose slope
07:22View SolutionAccording to Einstein's photoelectric equation the graph of K.E. of the photoelectron emitted from the metal versus the frequency of the incident radiation gives a straight line graph whose slope
07:59View SolutionThe speed of electromagnetic wave in vacuum depends upon the source of radiation
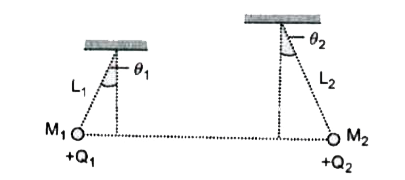
03:51View SolutionTwo small spheres of masses M1andM2 are suspended by weightless insulating threads of lengths L1andL2 The spheres carry charges Q1andq2 respectively . The spheres are suspended such that they are in level with one another and the threads are inclined to the vertical at angles of θ1andθ2 as shown . Which one o fthe following conditions , if θ1=θ2 ?
 06:39View Solution
06:39View SolutionA beam of light has three wavelengths 4144 Å , and 6216 Å with a total instensity of 3.6 xx 10^(-3) Wm^(-2) equally distributed amongst the three wavelengths. The beam falls normally on an area 1.0 cm^2 of a clean metallic surface of work function 2.3 eV. Assume that there is no loss of light by reflection number of photoelectrons liberated in two seconds.
03:29View SolutionA uniform monochromatic beam of light of wavelength 365xx10^(-9) m and intensity 10^(-8) Wm^(-2) falls on a surface having avsorption coefficient 0.8 and work functoin1.6 eV. Determine the rate of number of electrons emitted per m^(2) , power absorbed per m^(2) and the maximum kinetic energy of emitted photoelectrons.
04:20View SolutionA light source, emitting there wavelengths 500nm, 600nm and 700 nm, has a total power of 10^(-3) W and a beam diameter 2xx10^(-3)m . The density is distributed equally amongst the three wavelength. The beam shines normally on a metallic surface of area on 10^(-4) m^(2) and having a work function of 1.9 eV. Assuming that each photon liberates an electron, calculate the charge emitted per unit area in one second.
02:42View SolutionA beam of light consists of four wavelength 4000Å , 4800 A, 6000 A and 7000 Å , each of intensity 1.5xx10^(-3)Wm^(-2) . The beam falls normally on an area 10^(-4)m^(2) of a clean metalic surface of work function 1.9 eV . Assuming no loss of light energy (i.e. each capable photon emits one electron) calculate the number of photoelectrons liberated per second.
02:33View SolutionA beam of light has three lambda, 4144 "Å", 4972 "Å" "and"6216 "Å" with a total intensity of 3.6 xx10^(-3) Wm^(-2) equally distributed amongst the three lambda. The beam falls normally on an area 1.0 cm^(2) of a cleam metallic surface of work function 2.3 eV. Assume that there is no loss of light by reflection etc. Calculate the no. of photoelectrons emitted in 2 sec, in scientific notation, x xx 10^(y) find the value of y.
04:56View SolutionCalculate the kinetic energy of a photoelectron (in eV) emitted on shining light of wavelength 6.2×10−6m on a metal surface. The work function of the metal is 0.1 eV ?
11:03View SolutionA beam of light has three wavelengths 4000Å,5000Å,6000Å with a total intensity 3×10−3W/m2 equally distributed amongst the three wavelenth. The beam falls normally on an area 2cm2 of clean metallic surface of work function 2.4eV. Calculate photo current. (Assume each energetically suitable photon emits one electron)
03:54View SolutionFind the number of photons emitted per second by a source of power 25W. Assume, wavelength of emitted light = 6000Å. h = 6.62 xx 10^(-34)J.s
03:06View SolutionLight of wavelength 5000Å falls on a sensitive plate with photoelectric work function of 1.9 eV. The K.E. of the photo electron emitted will be
06:38View SolutionA light source emits a parallel beam of light of power 20 W. Wavelength of the light is 600 nm and it is incident on a perfectly absorbing surface . Find the number of photons absorbed by the surface per second and the total force exerted on the surface.
06:38View Solution
