If the gravitional force between two objects were proportional to 1/R (and not as 1R2) where R is separation between them , then a particle in circular orbit under such a force would have its orbital speed v proportional to Solution in Kannada
R1
1/R
R0
1/R2
The correct Answer is:C
Gravitational force provides necessary centripetal force
∴GMmR=mv2R
⇒v=√GM.
i.e. v is independent of R.
Thus correct choice is (c ).
Topper's Solved these Questions
- GRAVITATIONBOOK - MODERN PUBLICATIONCHAPTER - GRAVITATIONEXERCISE - Multiple Choice Questions (LEVEL-II)
64 Videos
- GRAVITATIONBOOK - MODERN PUBLICATIONCHAPTER - GRAVITATIONEXERCISE - Multiple Choice Questions (LEVEL-III Questions From AIEEE/JEE Examination)
6 Videos
- ELECTROSTATICSBOOK - MODERN PUBLICATIONCHAPTER - ELECTROSTATICSEXERCISE - RECENT COMPETITIVE QUESTIONS
39 Videos
- HEAT AND THERMODYNAMICSBOOK - MODERN PUBLICATIONCHAPTER - HEAT AND THERMODYNAMICSEXERCISE - Recent Competitve Questions
17 Videos
Similar Questions
In order that a body of 15 kg weighs zero at the equator, then the angular speed of earth is :
(g=10ms−1)
View SolutionTwo bodies of masses m and 4 m are placed at a distance r. The gravitational potential at a point on the line joining them where the gravitational field is zero is :
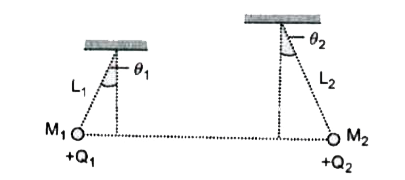
View SolutionTwo small spheres of masses M1andM2 are suspended by weightless insulating threads of lengths L1andL2 The spheres carry charges Q1andq2 respectively . The spheres are suspended such that they are in level with one another and the threads are inclined to the vertical at angles of θ1andθ2 as shown . Which one o fthe following conditions , if θ1=θ2 ?
View SolutionThe temperature of a gas contained in a closed vessel of constant volume increases by 1∘C when the pressure of the gas is increased by 1%. The initial temperature of the gas is:
View SolutionThe gravitational force between two objects is proportional to 1/R (and not as 1/R2) where R is separation between them, then a particle in circular orbit under such a force would have its orbital speed v proportional to
View SolutionIf the gravitational force were potential to 1/r , then a particle in a circular orbit under such a force would have its original speed:
View SolutionSuppose that the gravitational force between two bodies separated by a distance R becomes inversely proportional to R (and not as 1R2 as given by Newton's law of gravitation). Then the speed of a particle moving in a circular orbit of radius R under such a force would be proportional to
View SolutionIf the gravitional force between two objects were proportional to 1/R (and not as 1R^(2) ) where R is separation between them , then a particle in circular orbit under such a force would have its orbital speed v proportional to
View SolutionIf the gravitational force between two objects were proportional to 1/R (and not as 1/R^2 ) where R is the distance between them, then a particle in a circular path (under such a force) would have its orbital speed v_1 proportional to .........
View SolutionIf the gravitational force between two objects were proportional to 1/R (and not as 1/R2) where R is separation between them, then a particle in circular orbit under such a force would have its orbital speed v proportional to,
View SolutionIf the gravitational force between two objects were propotional to 1/R (and not as 1//R^(2) ) where R is separation between them, then a particle in circular orbit under such a force would have its orbital speed v proportional to
View SolutionIf the gravitational force between two objects were proportional to 1/R (and as 1/R2) where R is separation between them, then a particle in particular orbit under such a force would have its orbital speed v proportional to
View SolutionIf the gravitational force between two objects were propotional to 1/R (and not as 1/R2) where R is separation between them, then a particle in circular orbit under such a force would have its orbital speed v proportional to
View SolutionIf the gravitational force between two objects were proportional to 1/R (and as 1/R2) where R is separation between them, then a particle in particular orbit under such a force would have its orbital speed v proportional to
View SolutionIf the gravitational force between two objects were proportional to 1//R (and not as 1//R^(2) ), where R is the distance between them, then a particle in a circular path (under such a force) would have its orbital speed v, proportional to
View Solution
