A chain AB of length l is lying in a smooth horizontal tube so that the fraction 'h' of its length hangs freely and just touches the surface of the table with its end B. At a certain moment the end A of the chain is set free. The velocity of end A of the chain when it just slips out of tube is :
(##MOD_RPA_OBJ_PHY_C04_A_E01_089_Q01.png" width="80%">Solution in Kannada
h√2glh
√2ghlog(lh)
√2gllog(lh)
None of these
The correct Answer is:b
Here let M be the total mass of the chain Mland themass per unit length. Then accelerating force is weight of the hanging part. For a certain length x of the chain, the equation of motion is :
Ml×h×g=Ml×X×dυdt
orhg.dx=xdυdt.dx
or∫lhhg(dx)x=∫v0νdν
[logex]lh=υ22orυ=(2hgloge(l)h)1/2
(b) is the choice.
Topper's Solved these Questions
- LAWS OF MOTIONBook:MODERN PUBLICATIONChapter:LAWS OF MOTIONExercise:MULTIPLE CHOICE QUESTION (LEVEL-III)
Explore 2 Videos
- LAWS OF MOTIONBook:MODERN PUBLICATIONChapter:LAWS OF MOTIONExercise:RECENT COMPETITIVE QUESTIONS
Explore 7 Videos
- LAWS OF MOTIONBook:MODERN PUBLICATIONChapter:LAWS OF MOTIONExercise:Revision test
Explore 43 Videos
- HEAT AND THERMODYNAMICSBook:MODERN PUBLICATIONChapter:HEAT AND THERMODYNAMICSExercise:Recent Competitve Questions
Explore 17 Videos
- MAGNETOSTATICSBook:MODERN PUBLICATIONChapter:MAGNETOSTATICSExercise:RECENT COMPETITIVE QUESTION
Explore 5 Videos
Similar Questions
A block of mass m is placed on a surface with a vertical cross section given by y=x36If the coefficient of friction is 0.5, the maximum height above the ground at which the block can be placed without slipping is :
View SolutionA ball rests upon a flat piece of paper on a table top.The paper is pulled horizontally but quickly towards right as shown. Relative to its initial position with respect to the table, the ball :
(A) remains stationary if there is no friction between the paper and the ball.
(B) moves to the left and starts rolling backwards, i.e.to the left if there is a friction between the paper and the ball
(C) moves forward, i.e. in the direction in which the paper is pulled Here, the correct statement/s is/are :
View SolutionWhen a carpet is beaten with a stick, dust comes out of it. Explain.
View SolutionThe temperature of a gas contained in a closed vessel of constant volume increases by 1∘C when the pressure of the gas is increased by 1%. The initial temperature of the gas is:
View SolutionA gyromagnetic ratio of the electron revolving in a circular orbit of hydrogen atom is 8.8×1010Ckg−1. What is the mass of the electron? Given charge of the electron =1.6×10−19C.
View SolutionA chain (AB) of length l loaded in a smooth horizontal table so that its fraction of length h hangs freely and toucher the surface of the table with its end B. At a certain moment ,the end A of the chain is set free. With what velocity will this end the chain slip out of the table ? .
View SolutionA chain AB of length l is located in a smooth horizontal tube so that its fraction of length h hangs freely and touches the surface of the table with its end B(figure). At a certain moment the end A of the chain is set free. With what velocity will this end of the chain slip out of the tube?
View SolutionA uniform chain of mass m and length l is placed on a smooth table so that one−third length hanges freely as shown in the figure. Now the chain is released, with what velocity chain slips off the table?
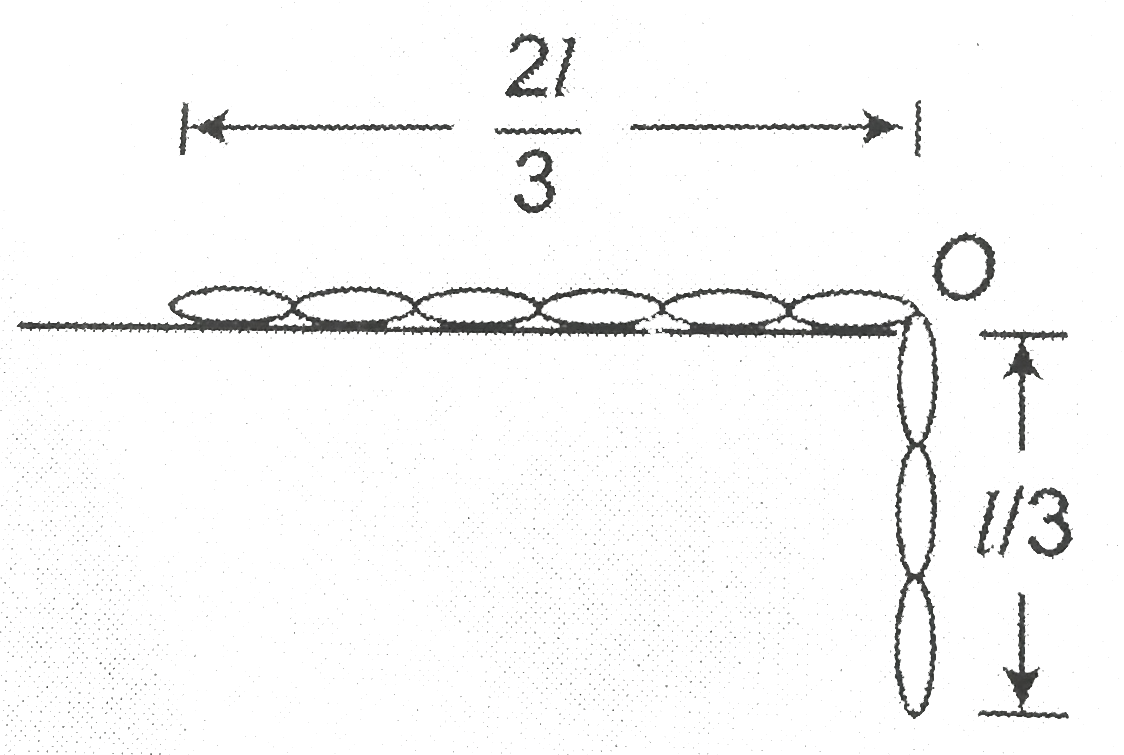
View SolutionA uniform chain of mass m and length l is lying on a horizontal table with one-third of its length hanging over the edge of the table.

What is the speed of the chain, when it just loses contact with the table?
View SolutionA chain of mass M and length l is held vertically such that its bottom end just touches the surface of a horizontal table. The chain is released from rest. Assume that the portion of chain on the table does not form a heap. The momentum of the portion of the chain above the table after the top end of the chain falls down by a distance l/8 .
View SolutionA chain AB of length l is located in a smooth horizontal tube so that its fraction of length h hangs freely and touches the surface of the table with its end B(figure). At a certain moment the end A of the chain is set free. With what velocity will this end of the chain slip out of the tube?

View SolutionA uniform chain of mass m and length l is on a smooth horizontal table with (1/n)^th part of its leght is hanging from one end of the table. The velocity of the chain, when it completely slips off the table is
View SolutionA chain AB of length l is located on a smooth horizontal table so that its fraction of length h hange freely with end B on the table. At a certain moment, the end A of the chain is set free. With what velocity with this end of the chain slip off the table?
View SolutionA chain AB of length l is located on a smooth horizontal table so that its fraction of length h hange freely with end B on the table. At a certain moment, the end A of the chain is set free. With what velocity with this end of the chain slip off the table?
View SolutionA unifrom chain of length .L. is placed on a smooth table of height .h. (h > L) with a length . L . hanging from the edge of the table. The chain begins to slide down the table. When the end of the chain is about to leave the edge of the table its velocity is
View Solution