Question

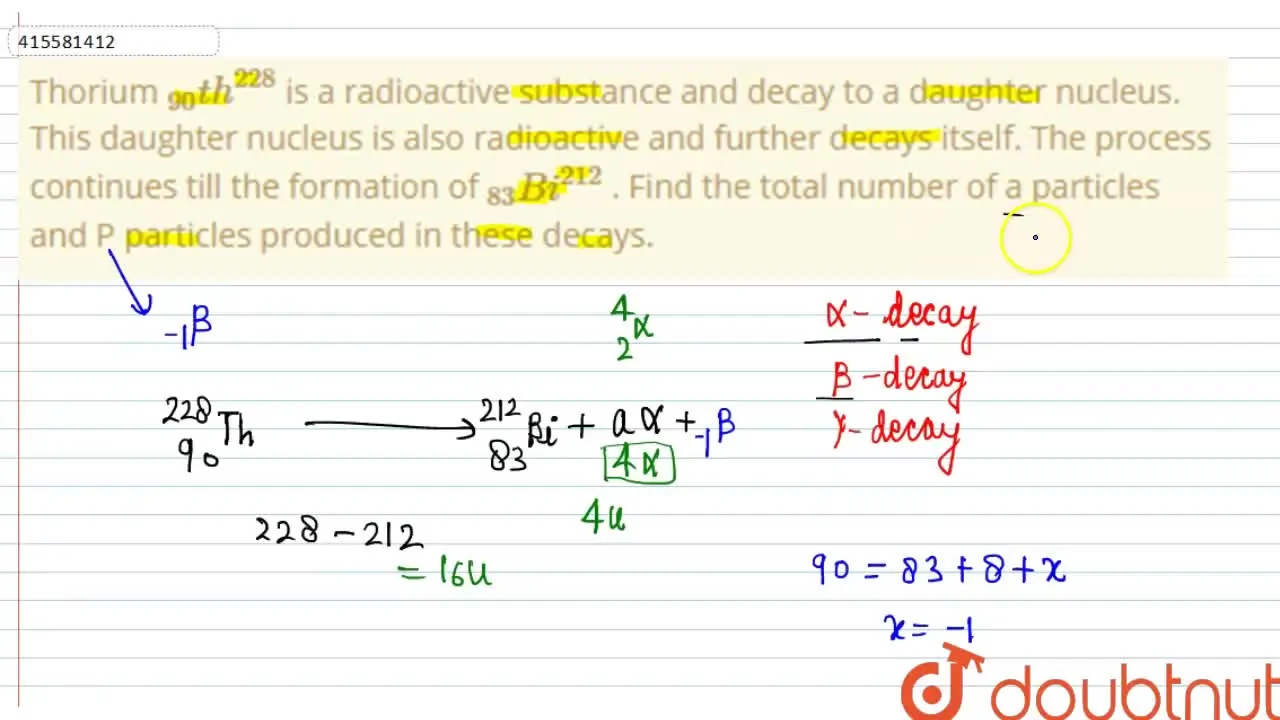
Thorium ${}_{90}t{h}^{228}$ is a radioactive substance and decay to a daughter nucleus. This daughter nucleus is also radioactive and further decays itself. The process continues till the formation of ${}_{83}B{i}^{212}$ . Find the total number of a particles and P particles produced in these decays.

Explanation

To solve the problem of determining the total number of alpha (α) particles and beta (β) particles produced during the decay of Thorium-228 to Bismuth-212, we can follow these steps:

Step 1: Identify the initial and final nuclei
- The initial nucleus is Thorium-228, represented as \( _{90}^{228}\text{Th} \).
- The final nucleus is Bismuth-212, represented as \( _{83}^{212}\text{Bi} \).

Step 2: Determine the change in atomic number and mass number
- The atomic number of Thorium is 90, and for Bismuth, it is 83.
- The mass number of Thorium is 228, and for Bismuth, it is 212.

Step 3: Calculate the change in atomic number
- Change in atomic number = Initial atomic number - Final atomic number
- Change in atomic number = 90 - 83 = 7

Step 4: Calculate the change in mass number
- Change in mass number = Initial mass number - Final mass number
- Change in mass number = 228 - 212 = 16

Step 5: Determine the number of alpha particles
- Each alpha particle (α) has an atomic number of 2 and a mass number of 4.
- Let \( n \) be the number of alpha particles emitted.
- The total change in atomic number due to alpha decay is \( 2n \).
- The total change in mass number due to alpha decay is \( 4n \).

From the change in atomic number:
\( 2n = 7 \)
From the change in mass number:
\( 4n = 16 \)

Step 6: Solve for \( n \)
- From \( 4n = 16 \):
\( n = \frac{16}{4} = 4 \)
- Thus, 4 alpha particles are emitted.

Step 7: Determine the number of beta particles
- The total charge change must also be balanced.
- The initial charge (atomic number) is 90, and after emitting 4 alpha particles, the charge contributed by them is \( 4 \times 2 = 8 \).
- Therefore, the final charge after alpha decay will be:
\( 90 - 8 + x = 83 \)
Where \( x \) is the number of beta particles (β) emitted.

Step 8: Solve for \( x \)
- Rearranging gives:
\( 90 - 8 + x = 83 \)
\( x = 83 - 82 \)
\( x = -1 \)
- Since each beta particle has a charge of -1, we need 1 beta particle to balance the charge.

Final Answer
- Total number of alpha particles (α) produced = 4
- Total number of beta particles (β) produced = 1