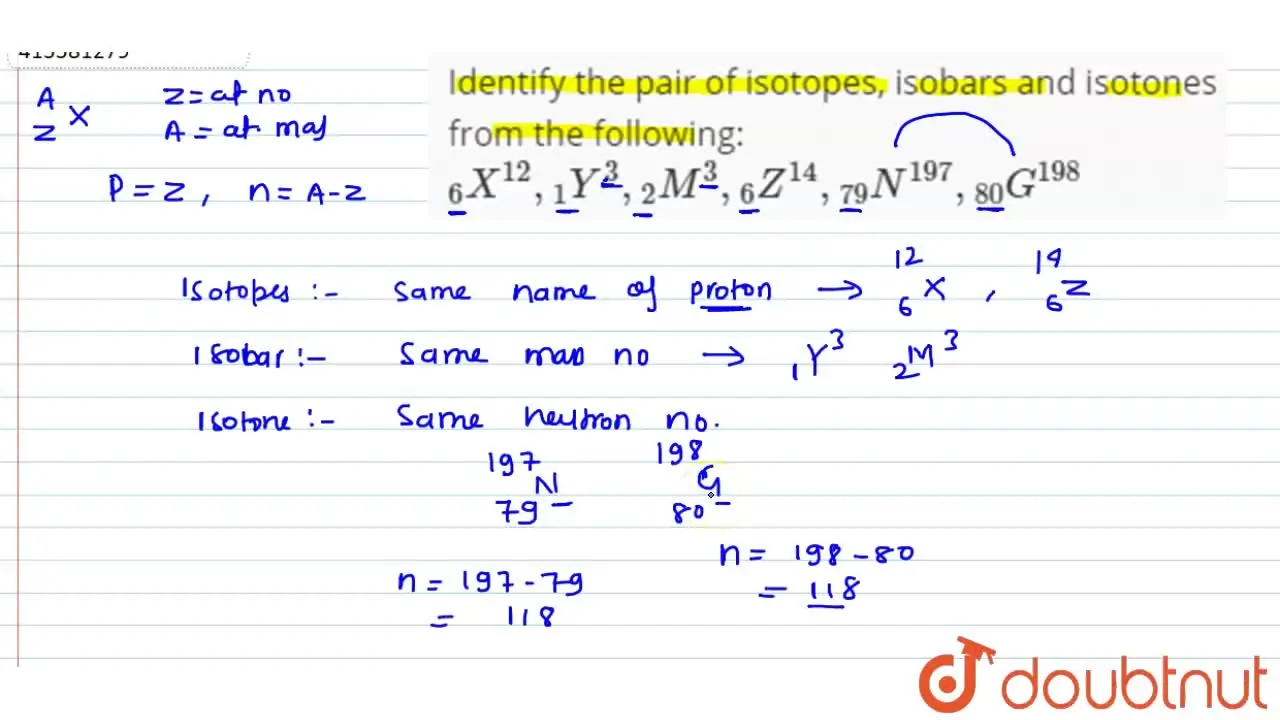
Identify the pair of isotopes, isobars and isotones from the following:
6X12,1Y3,2M3,6Z14,79N197,80G198
Isotopes: 6X12 and 6Z14
Isobars: 1Y3 and 2M3
Isotones: 79N197 and 80G198
Related Playlists
- BOOK - MODERN PUBLICATIONCHAPTER - NUCLEIEXERCISE - TOUGH & TRICKY PROBLEMS9videos
NUCLEI
- BOOK - MODERN PUBLICATIONCHAPTER - NUCLEIEXERCISE - NCERT FILE SOLVED (TEXTBOOK EXERCISES)22videos
NUCLEI
- BOOK - MODERN PUBLICATIONCHAPTER - NUCLEIEXERCISE - PRACTICE PROBLEMS38videos
NUCLEI
- BOOK - MODERN PUBLICATIONCHAPTER - MOVING CHARGES AND MAGNETISMEXERCISE - CHAPTER PRACTICE TEST13videos
MOVING CHARGES AND MAGNETISM
- BOOK - MODERN PUBLICATIONCHAPTER - RAY OPTICS AND OPTICAL INSTRUMENTSEXERCISE - CHAPTER PRACTICE TEST14videos
RAY OPTICS AND OPTICAL INSTRUMENTS
Similar Questions
Select the pairs of isotops and isotons form the following nuclei .6C12,.7N14,.15P30,.15P31.
View SolutionSelect the pairs of isobars and isotons form the following nuclei .6C14,.7N13,.7N14,.8O16:
View SolutionClassify the following six nuclides into (i) isotones, (ii) isotopes, and (iii) isobars :
126C, 32He, 19880Hg, 31H, 19779Au, 146CView SolutionIdentify the pairs which are not of isotopes?
(1) 126X,163 (2) 3517X,3717 (3) 146X,147 (4) 84X,85YView SolutionGroup the following six nuclides into three pairs of (i) isotones (ii) isotopes and (iii) isobars.
.6C12,.2He3,.80Hg198,.1H3,.79Au197,.6C14View SolutionGive one example of each of the following:
(i) Isotope of .3517Cl (ii) Isobar of .4018Ar (iii) Isotone of .146CView SolutionThe direction ratios of the line x+1=2y=−12z are
(1) (6,6,-1) (2) (12,6,1) (3) (6,-6,1) (4) (12,6,-1)View SolutionSimplify the following: 7x3+8y3−(4x+3y)(16x2−12xy+9y2)
View SolutionFind the angle between the following pairs of line: x−12=y−23=z−36,x+1=y+22=z−32
View SolutionThe distance of the plane 6x−3y+2z−14=0 from the origin is
View Solution