An electron move in a circular path of radius 2 cm under the influence of a magnetic field of 0.1 teals applied perpendicular to its plane of motion . Calculate the de Broglie wavelength of electron.
To solve the problem of finding the de Broglie wavelength of an electron moving in a circular path under the influence of a magnetic field, we can follow these steps:
Step 1: Convert the radius from centimeters to meters
The radius of the circular path is given as 2 cm. We need to convert this to meters for our calculations.
Radius(r)=2cm=2×10−2m
Hint: Remember that 1 cm = 0.01 m, so to convert cm to m, multiply by 10−2.
Step 2: Identify the charge and mass of the electron
The charge (q) of the electron is given as:
q=1.6×10−19C
The mass (m) of the electron is:
m=9.1×10−31kg
Hint: These constants are fundamental properties of the electron and can be found in standard physics tables.
Step 3: Use the formula for the radius of the circular motion in a magnetic field
The radius of the circular path of a charged particle in a magnetic field is given by the formula:
r=mvqB
Where:
- r is the radius,
- m is the mass of the electron,
- v is the velocity of the electron,
- q is the charge of the electron,
- B is the magnetic field strength.
Rearranging this formula to solve for velocity v:
v=qBrm
Hint: This formula comes from equating the centripetal force to the magnetic force acting on the electron.
Step 4: Substitute the values to find the velocity
Substituting the known values into the equation:
B=0.1T(Tesla)
v=(1.6×10−19C)(0.1T)(2×10−2m)9.1×10−31kg
Calculating this gives:
v≈3.5×108m/s
Hint: Ensure that you perform the multiplication and division carefully, keeping track of units.
Step 5: Calculate the de Broglie wavelength
The de Broglie wavelength (λ) is given by the formula:
λ=hp
Where p (momentum) is given by p=mv. Thus, we can express the wavelength as:
λ=hmv
Substituting the values:
h=6.626×10−34Js(Planck′sconstant)
λ=6.626×10−34Js(9.1×10−31kg)(3.5×108m/s)
Calculating this gives:
λ≈2.08×10−13m
Hint: Make sure to keep track of significant figures and units when performing calculations.
Final Answer
The de Broglie wavelength of the electron is approximately:
λ≈2.08×10−13m
The radius of the circular path is given as 2 cm. We need to convert this to meters for our calculations.
The charge (q) of the electron is given as:
The radius of the circular path of a charged particle in a magnetic field is given by the formula:
- r is the radius,
- m is the mass of the electron,
- v is the velocity of the electron,
- q is the charge of the electron,
- B is the magnetic field strength.
Substituting the known values into the equation:
v=(1.6×10−19C)(0.1T)(2×10−2m)9.1×10−31kg
The de Broglie wavelength (λ) is given by the formula:
The de Broglie wavelength of the electron is approximately:
Topper's Solved these Questions
- DUAL NATURE OF RADIATION AND MATTER BOOK - MODERN PUBLICATIONCHAPTER - DUAL NATURE OF RADIATION AND MATTER EXERCISE - COMPETITION FILE (OBJECTIVE TYPE QUESTIONS ( A - Multiple Choice Questions))
15 Videos
- DUAL NATURE OF RADIATION AND MATTER BOOK - MODERN PUBLICATIONCHAPTER - DUAL NATURE OF RADIATION AND MATTER EXERCISE - COMPETITION FILE (OBJECTIVE TYPE QUESTIONS ( B - Multiple Choice Questions) (AIPMT/NEET & Other State Boards for Medical Entrance))
26 Videos
- DUAL NATURE OF RADIATION AND MATTER BOOK - MODERN PUBLICATIONCHAPTER - DUAL NATURE OF RADIATION AND MATTER EXERCISE - Revision Exercises (Long Answer Questions)
3 Videos
- CURRENT ELECTRICITYBOOK - MODERN PUBLICATIONCHAPTER - CURRENT ELECTRICITYEXERCISE - Chapter Practice Test
15 Videos
- ELECTRIC CHARGES AND FIELDS BOOK - MODERN PUBLICATIONCHAPTER - ELECTRIC CHARGES AND FIELDS EXERCISE - Chapter Practice Test
15 Videos
Similar Questions
A particle having the same charge as of electron moves in a circular path of radius 0.5 cm under the influence of a magnetic field of 0.5 T. If an electric field of 100V/m makes it to move in a straight path, then the mass of the particle is ( Given charge of electron =1.6×10−19C)
View SolutionAn electron after being accelerated through a potential difference of 100 V enters a uniform magnetic field of 0⋅004T, perpendicular to its direction of motion. Calculate the radius of the path described by the electron.
View Solution
Knowledge Check
An electron is moving on a circular path of radius r with speed v in a transverse magnetic field B. e/m for it will be
AvBrBBrvCBvrDvrBAn electron is moving in a circular path under the influence of a transverse magnetic field of 3.57×10−2 T.If the value of e/m is 1.76×1011 c/kg, the frequency of revolution of the electron is
A6.82 MHzB1 GHzC100 MHzD62.8 MHzThe momentum of α-particles moving in a circular path of radius 10 cm in a perpendicular magnetic field of 0.05 tesla will be :
A1.6×10−20km m/sB1.6×10−21kb m/sC1.6×10−19kg m/sD1.6×10−18kg m/s
A α -parhticle moves in a circular path of radius 0.83cm in the presence of a magnetic field of 0.25Wb/m2. The de-Broglie wavelength assocaiated with the particle will be
View SolutionAn electron is moving in a circular path under the influence fo a transerve magnetic field of 3.57×10−2T. If the value of e/m is 1.76×10141C/kg. The frequency of revolution of the electron is
View SolutionIn a H-atom an electron is in 2nd excited state and its radius =4.75Å calculate the de-broglie wavelength of the electron
View SolutionAn electron is moving through a field. It is moving (i) opposite an electric field (ii) perpendicular to a magnetic field as shown. For each situation the de - Broglie wave length of electron
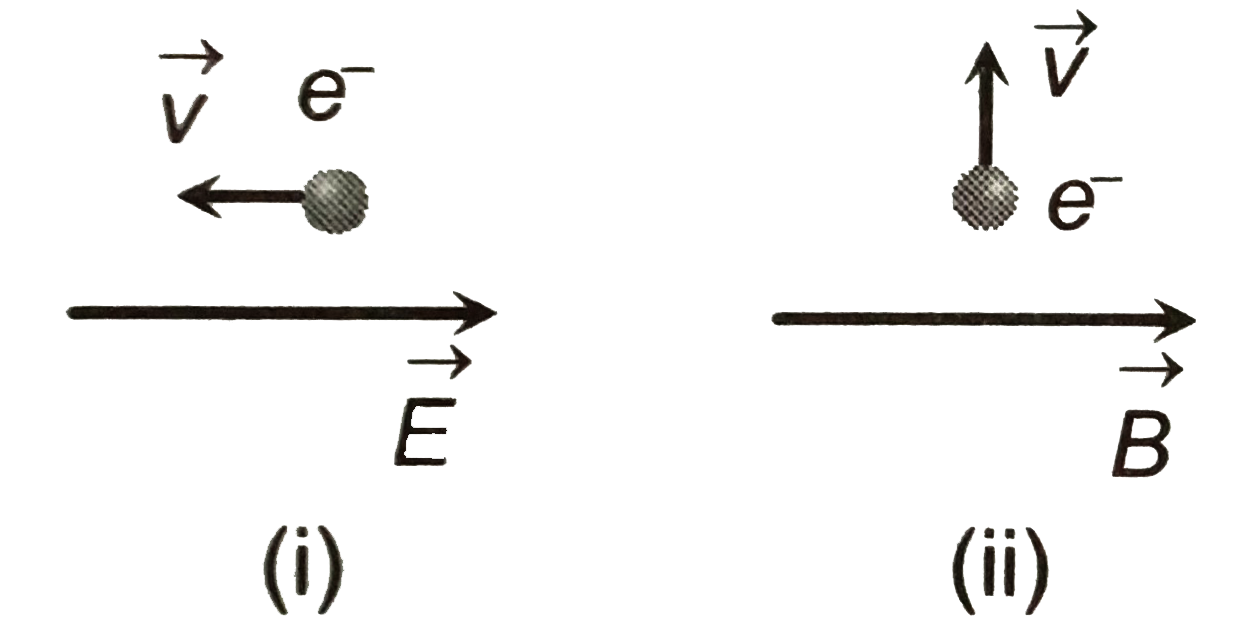
View SolutionIf an electron moves in a circular path with velocity v in a magnetic field B, then during a semicircular journey, its gain in energy will be-
View Solution
