Question

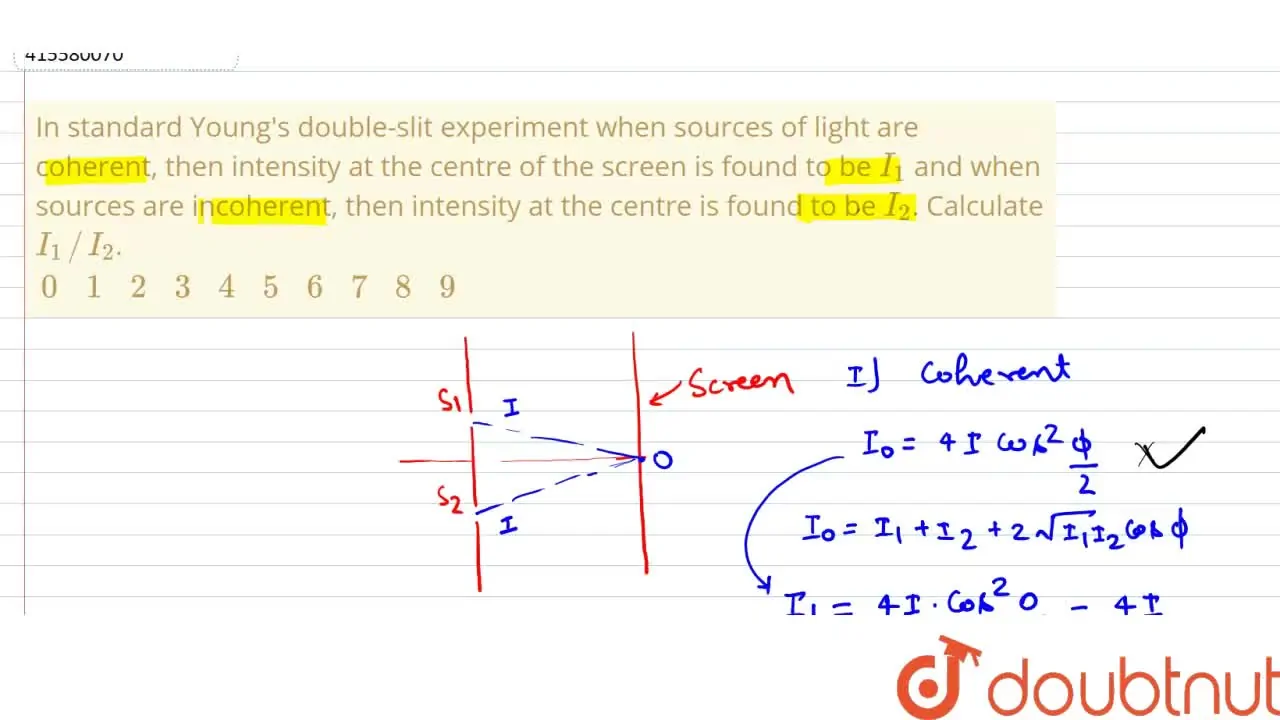
In standard Young's double-slit experiment when sources of light are coherent, then intensity at the centre of the screen is found to be ${\displaystyle I_1}$ and when sources are incoherent, then intensity at the centre is found to be ${\displaystyle I_2}$. Calculate ${\displaystyle I_1/I_2}$.

${\displaystyle \begin{array}{llllllllll}0& 1& 2& 3& 4& 5& 6& 7& 8& 9\end{array}}$

Answer

2

Explanation

To solve the problem of calculating the ratio $$\frac{I_1}{I_2}$$ in Young's double-slit experiment, we will analyze the situation for both coherent and incoherent light sources.

1. Understanding the Setup:
In Young's double-slit experiment, we have two slits (S1 and S2) illuminated by a coherent light source. The light waves from these slits interfere with each other, creating a pattern of bright and dark fringes on a screen.

2. Intensity with Coherent Sources:
When the sources are coherent, the intensity at the center of the screen (where the path difference is zero) is given by:
\( I_1 = 4I \)
Here, \( I \) is the intensity of light from each slit. The factor of 4 comes from the constructive interference of the two coherent waves.

3. Intensity with Incoherent Sources:
When the sources are incoherent, the light from the two slits does not interfere in the same way. Instead, the intensities simply add up:
\( I_2 = I + I = 2I \)

4. Calculating the Ratio:
Now, we can find the ratio \( \frac{I_1}{I_2} \):
\( \frac{I_1}{I_2} = \frac{4I}{2I} = 2 \)

Final Answer:
Thus, the ratio \( \frac{I_1}{I_2} \) is:
\( \frac{I_1}{I_2} = 2 \)