How does the sign of the phase angle ϕ, by which the supply voltage leads the current in an LCR serices circuit, change as the supply frequency is gradually increased from very low to very high values ?
Let ϕ be the phase angle by which voltage leads the current in an LCR circuit .
Let XL be the reactance offered by inductance, L, XC be the reactance offered by capacitance C, R be the resistance in the circuit and ω be the angular frequency , them
tan ϕ=XL−XCR=ωL−1ωCR
If omega is lower than resonant frequency (ω0) tan ϕ is negative which means ϕ is negative when ω=ω0,
ωL=1ωC , which gives tan ϕ = 0
∴ ϕ = 0
when ω is higher than resonant frequency , tan ϕ is positive which means ϕ is positive.
Topper's Solved these Questions
- ALTERNATING CURRENT BOOK - MODERN PUBLICATIONCHAPTER - ALTERNATING CURRENT EXERCISE - HIGHER ORDER THINKING SKILLS & ADVANCED LEVEL
7 Videos
- ALTERNATING CURRENT BOOK - MODERN PUBLICATIONCHAPTER - ALTERNATING CURRENT EXERCISE - Revision Exercises ( Very short Annwer Qustion )
71 Videos
- ALTERNATING CURRENT BOOK - MODERN PUBLICATIONCHAPTER - ALTERNATING CURRENT EXERCISE - NCERT FILE ADDITIONAL EXERCISES
15 Videos
- ATOMS BOOK - MODERN PUBLICATIONCHAPTER - ATOMS EXERCISE - Chapter Practice Test
16 Videos
Similar Questions
How does the sign of the phase angle o, by which the supply voltage leads the current in an LCR series circuit, change as the supply frequency is gradually increased from very low to very high values ?
View SolutionAn inductor, a resistor and a capacitor are joined in series with an AC source. As the frequency of the source is slightly increased from a very low value, the reactance
View Solution
Knowledge Check
In series LCR circuit, the phase angle between supply voltage and current is
Atanϕ=XL−XCRBtanϕ=RXL−XCCtanϕ=RXL+XCDtanϕ=XL+XCRWhen the frequency of the AC voltage applied to a series LCR circuit is gradually increased from a low value, the impedance of the circuit
Amonotonically increasesBfirst increases and then decreasesCfirst decreases and then increasesDmonotonically decreasesAn inductor, a capacitor, and a resistor are connected in series with a frequency generator to complete a circuit. The frequency generator can supply alternating current at frequencies ranging from about 0 Hz to 100 MHz. As the frequency is increased from the lowest to the highest value of the generator there is a particular value for which the voltage across the resistor is maximum. At that frequency
AThe voltage across the inductor is zero at all timesBThe voltage across the capacitor is zero at all timesCThe voltage across the inductor and the capacitor are zero at all timesDThe voltage across the inductor and the capacitor are opposite in phase at all times
Find the values of the phase difference between the current and the voltage in the series LCR circuit shown below, which one leads in phase: current or voltage?
View Solution(i) Find the value of the phase difference between the current and the voltage in the series LCR circuit shown below. Which one leads in phase : current or voltage ?
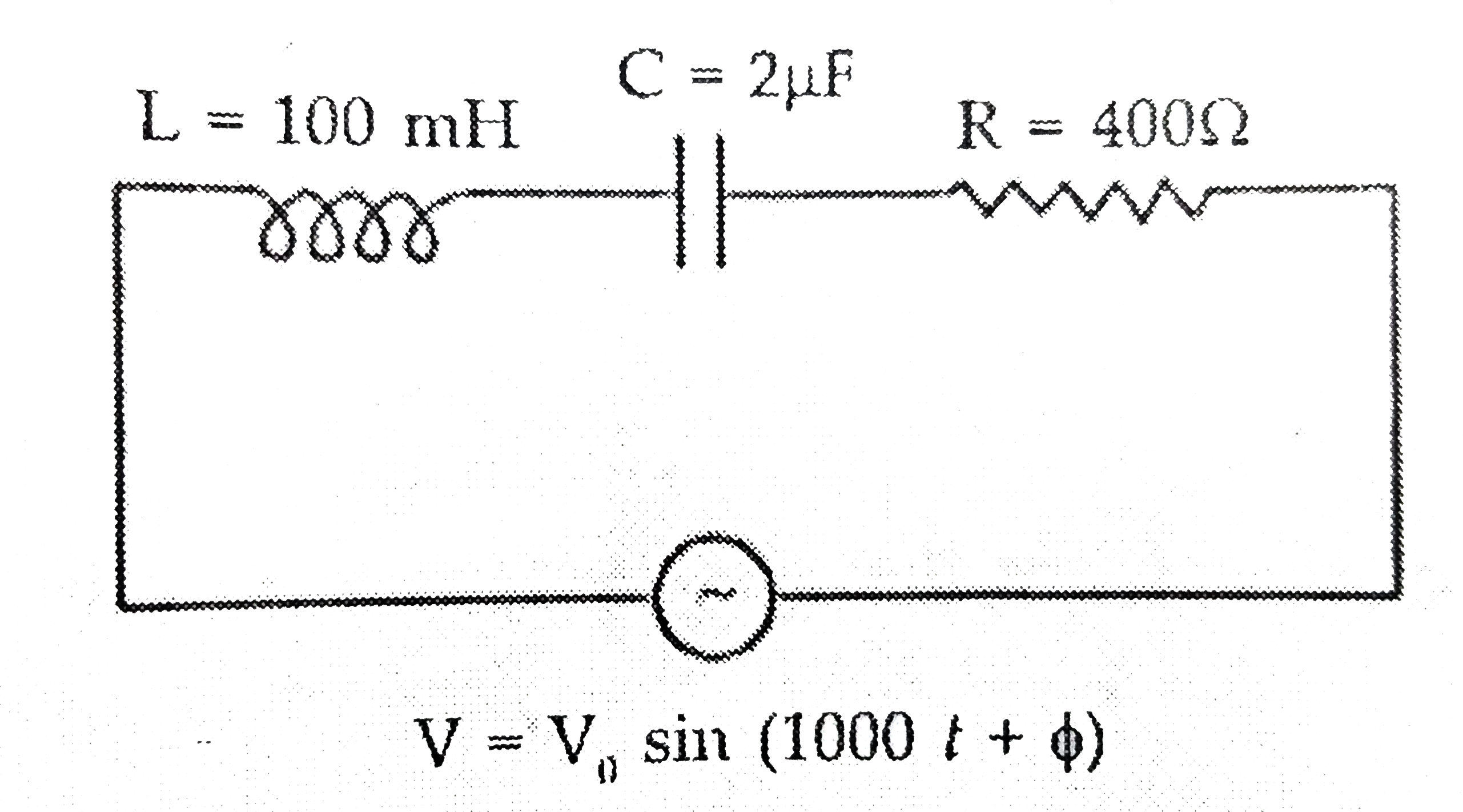
(ii) What making any other change, find the value of the additional capacitor C, to be connected in parallel with the capacitor C, in order to make the power factor of the circuit unity.
View SolutionHow do the energy gaps between successive electron energy levels in an atom very from low to high n values ?
View SolutionIn an a.c circuit, value of voltage and current change every instant. Therefore, power of an a.c. circuit at any instant is the product of instantaneous voltages (E) and instantaneous current (I). The average power supplied to a pure resistance R over a complete cycle of a.c is P=Eυ.Iυ. When circuit is inductive, average power/cycle =EυIυcosϕ, where ϕ is the phase angle between alternating voltage an altenating current in the circuit.
In an a.c. circuit, 800mH inductor and a 60μF capacitor are connected in series with 15ohm resistance. The a.c. supply to the circuit is 230V,50Hz
The electrial energy spent in running the circuit for one hour is
View SolutionIn an a.c circuit, value of voltage and current change every instant. Therefore, power of an a.c. circuit at any instant is the product of instantaneous voltages (E) and instantaneous current (I). The average power supplied to a pure resistance R over a complete cycle of a.c is P=Eυ.Iυ. When circuit is inductive, average power/cycle =EυIυcosϕ, where ϕ is the phase angle between alternating voltage an altenating current in the circuit.
In an a.c. circuit, 800mH inductor and a 60μF capacitor are connected in series with 15ohm resistance. The a.c. supply to the circuit is 230V,50Hz
The total power abosrbed per cycle by all the three circuit elements is
View Solution
