Consider an x-y plane and mark the locations A, B and C whose coordinates are given to be (5, 2), (5, 8) and (-5, 8) respectively. A uniform electric field of 200 N/C exists in space directed along the negative x-direction. Calculate (i)VB−VA(ii)VC−VA(iii)VB−VC.
To solve the problem, we need to calculate the potential differences between the points A, B, and C in the presence of a uniform electric field. The electric field is given as E=200N/C directed along the negative x-direction.
Step 1: Identify the coordinates of points A, B, and C
- Point A: (5,2)
- Point B: (5,8)
- Point C: (−5,8)
Step 2: Calculate VB−VA
To find the potential difference VB−VA, we use the formula:
VB−VA=−E⋅d
where d is the displacement vector from A to B.
1. Calculate the displacement vector →dAB:
→dAB=(xB−xA,yB−yA)=(5−5,8−2)=(0,6)
2. The electric field vector →E is:
→E=−200^i
3. Calculate the dot product →E⋅→dAB:
→E⋅→dAB=(−200^i)⋅(0^i+6^j)=0
4. Therefore, the potential difference is:
VB−VA=−0=0V
Step 3: Calculate VC−VA
Now, we calculate VC−VA using the same method.
1. Calculate the displacement vector →dAC:
→dAC=(xC−xA,yC−yA)=(−5−5,8−2)=(−10,6)
2. Calculate the dot product →E⋅→dAC:
→E⋅→dAC=(−200^i)⋅(−10^i+6^j)=2000
3. Therefore, the potential difference is:
VC−VA=−2000V
Step 4: Calculate VB−VC
Finally, we calculate VB−VC.
1. Calculate the displacement vector →dBC:
→dBC=(xC−xB,yC−yB)=(−5−5,8−8)=(−10,0)
2. Calculate the dot product →E⋅→dBC:
→E⋅→dBC=(−200^i)⋅(−10^i)=2000
3. Therefore, the potential difference is:
VB−VC=−2000V
Summary of Results
- VB−VA=0V
- VC−VA=−2000V
- VB−VC=−2000V
- Point A: (5,2)
- Point B: (5,8)
- Point C: (−5,8)
To find the potential difference VB−VA, we use the formula:
VB−VA=−E⋅d
where d is the displacement vector from A to B.
→dAB=(xB−xA,yB−yA)=(5−5,8−2)=(0,6)
→E=−200^i
→E⋅→dAB=(−200^i)⋅(0^i+6^j)=0
VB−VA=−0=0V
Now, we calculate VC−VA using the same method.
→dAC=(xC−xA,yC−yA)=(−5−5,8−2)=(−10,6)
→E⋅→dAC=(−200^i)⋅(−10^i+6^j)=2000
VC−VA=−2000V
Finally, we calculate VB−VC.
→dBC=(xC−xB,yC−yB)=(−5−5,8−8)=(−10,0)
→E⋅→dBC=(−200^i)⋅(−10^i)=2000
VB−VC=−2000V
- VB−VA=0V
- VC−VA=−2000V
- VB−VC=−2000V
Topper's Solved these Questions
- ELECTROSTATIC POTENTIAL AND CAPACITANCE BOOK - MODERN PUBLICATIONCHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITANCE EXERCISE - PRACTICE PROBLEMS 1
10 Videos
- ELECTROSTATIC POTENTIAL AND CAPACITANCE BOOK - MODERN PUBLICATIONCHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITANCE EXERCISE - PRACTICE PROBLEMS 2
21 Videos
- ELECTROMAGNETIC WAVESBOOK - MODERN PUBLICATIONCHAPTER - ELECTROMAGNETIC WAVESEXERCISE - CHAPTER PRACTICE TEST
14 Videos
- MAGNETISM AND MATTERBOOK - MODERN PUBLICATIONCHAPTER - MAGNETISM AND MATTEREXERCISE - CHAPTER PRACTICE TEST FOR BOARD EXAMINATION
16 Videos
Similar Questions
A uniform electric field E exists in a region of space. Refer to the given figure for the location of three points A, B and C.
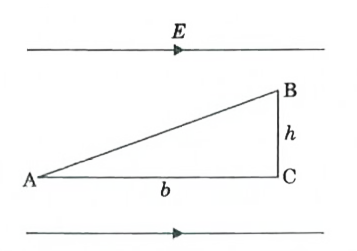
Find: (a)VB−VA (b)VC−VA
View SolutionA uniform electric field of 30NC−1 exists along the X-axis calculate the potential difference VB−VA between the points A (4m,2m) and B(10m,5m).
View Solution
Knowledge Check
In uniform electric field E=10N/C, find

a. VA−VB , b. VB−VCA−10V and 20VB10V and −10VC30V and 20VD−10V and 40VA uniform electric field of 400Vm is directed at 45∘ above the x-axis as shown in the figure. The potential difference VA−VB is given by
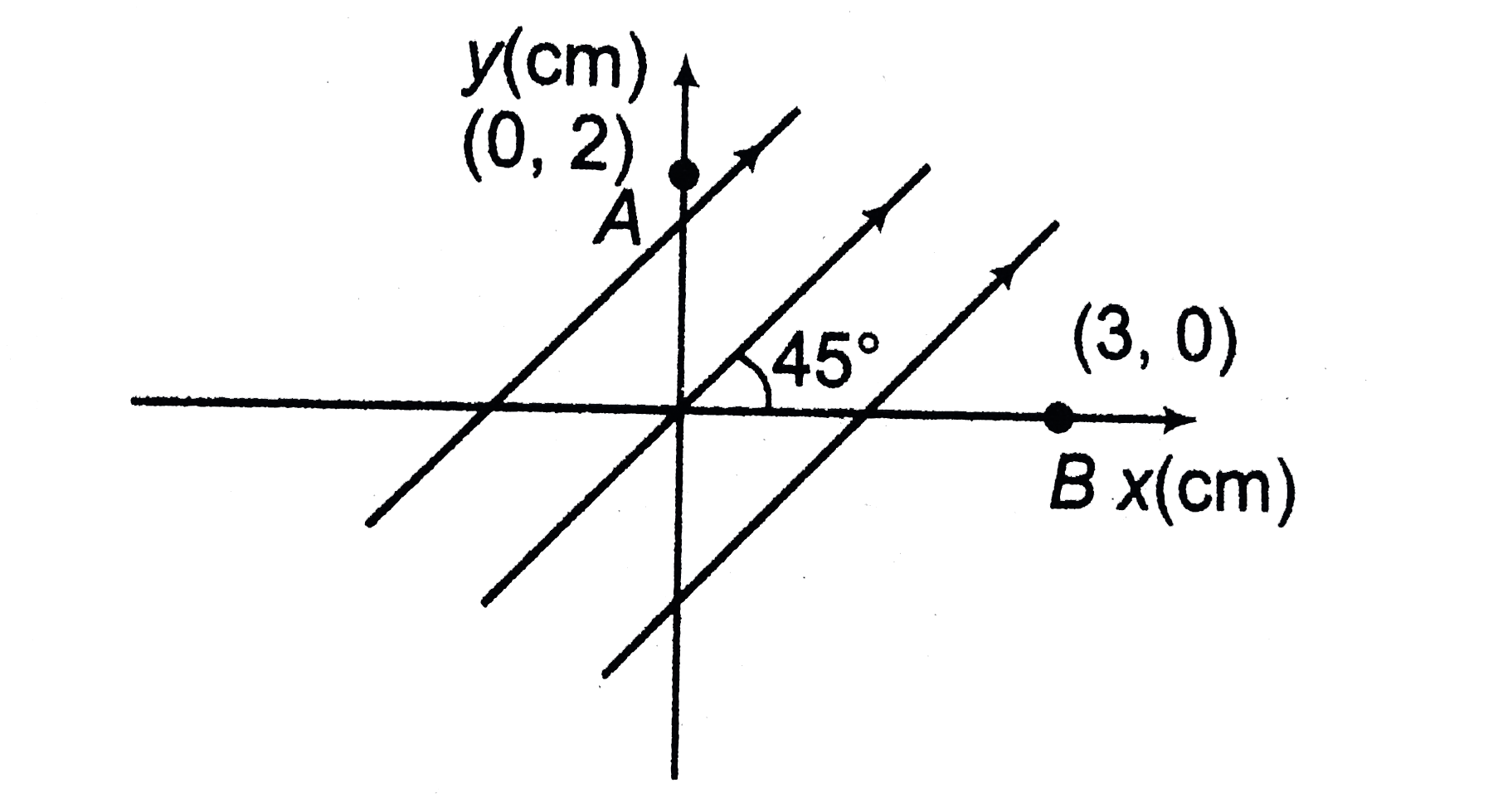 A0B4VC6.4VD2.8V
A0B4VC6.4VD2.8VA uniform electric field exists in x−y plane. The potential of points A(−2m,2m),B(−2m,2m) and C(2m,4m) are 4V,16V and 12V respectively. The electric field is
A(4ˆi+5ˆj)VmB(3ˆi+4ˆj)VmC−(3ˆi+4ˆj)VmD(3ˆi+4ˆj)Vm
When an electron moves from A to B along and electric field line as shown in Fig. the electric field does 3.94×10−19J of work on it. What are the electric potential differences
(VB−VA),(VC−VA),(VC−VB) ?
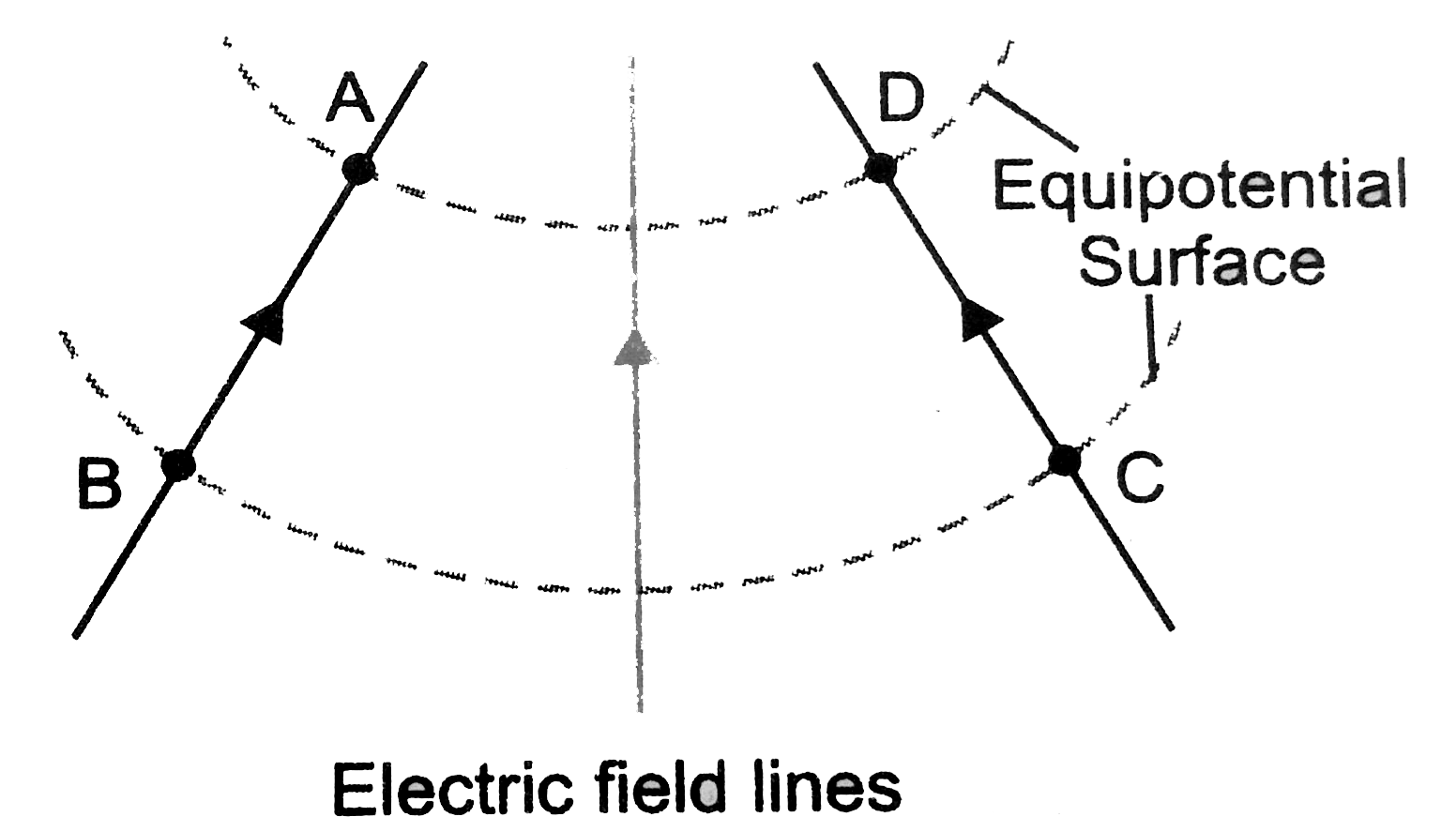
View SolutionAn electric field of 20 N/C exists along the negative x-axis in space. Calculate the potential difference VB−VA, where the points A and B are given by :
(a) A= (0, 0), B=(0, 4m)
(b) A=(2m, 1m), B=(4m, 3m)
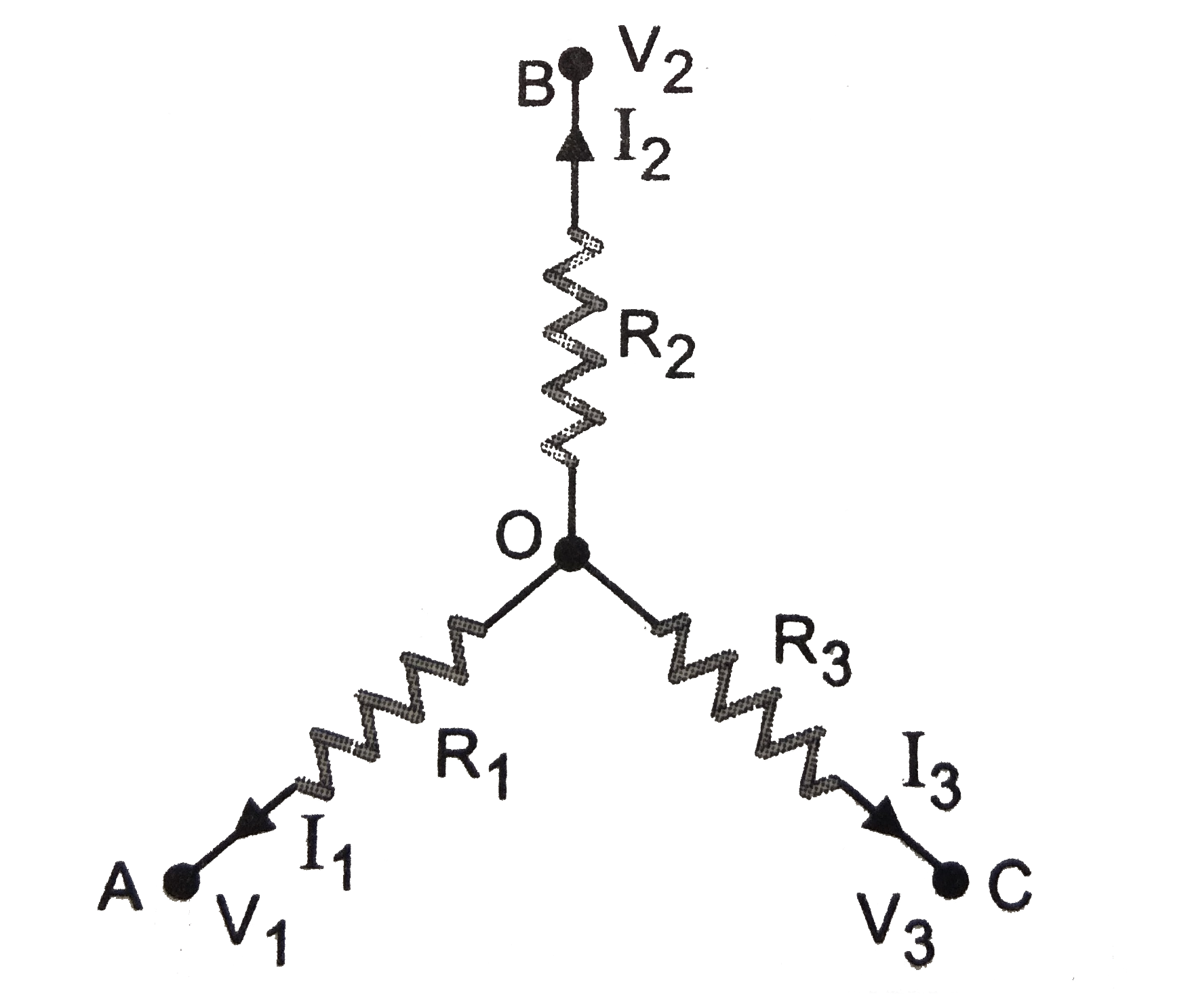
View SolutionA circuit has a section ABC if the potential at point A,BandC are V1,V2andV3 respectively , calculate the potential at point O
View SolutionA uniform electric field exists in x-y plane. The potential of points A(2m,2m),B(−2m,2m) and C(2m,3m) are 4V,16V and 12V respectively. The electric field is
View SolutionThe direction of the field B at P is
the V shaped wire is in x-y plane
View Solution