Two point charges of +q each are placed at two diagonally opposite corners of a square. Another two point charges of -q charge each are placed at the two remaining corners. Take the edge length of square equal to l. Calculate (i) work needed to move these charges slowly from an infinite separation to this given configuration. (ii) Assume that four charges at corners of the square are held fixed. Another charge Q is to be moved from infinity to the centre of this square slowly. What additional work is required to do so?
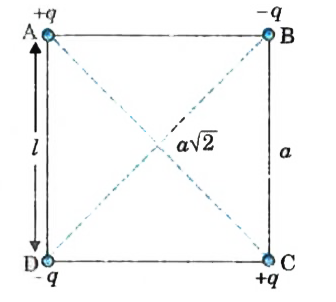
The given arrangement of charges is shown below:
(i) Work required to move these charges slowly from infinite separation to this given configuration will be equal to the difference in electric potential energy of the systems at this configuration and at infinite separation.
∴W=U−U∞
We know that U∞=0 and U= Total electric potential energy of the four charges
∴W=U
=14πε0[qAqBAB+qAqCAC+qAqDAD+qBqCBC+qCqDCD+qBqDBD]
Here, qA=+q,qB=−q,qc=+q,qD=−q
AB=BC=CD=AD=l
AC=BD=√AD2+DC2=√2l
⇒W=14πε0
[−q2l+q2√2l+(−q2)l+(−q2)l+(−q2)l+q2√2l]
=−q24πε0l(4−√2)
(ii) To calculate the work required to move another charge Q from infinity to the centre of the square, we will first calculate the electrostatic potential energy of the system of charges when the charge Q is placed at the centre:
U=14πε0[qQAO−qQBO+qQCO−qQDO]
Here, AO=BO=CO=DO
∴U=0
∴ Work done in bringing Q from infinity to centre, W=U=0
Topper's Solved these Questions
- ELECTROSTATIC POTENTIAL AND CAPACITANCE BOOK - MODERN PUBLICATIONCHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITANCE EXERCISE - PRACTICE PROBLEMS 1
10 Videos
- ELECTROSTATIC POTENTIAL AND CAPACITANCE BOOK - MODERN PUBLICATIONCHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITANCE EXERCISE - PRACTICE PROBLEMS 2
21 Videos
- ELECTROMAGNETIC WAVESBOOK - MODERN PUBLICATIONCHAPTER - ELECTROMAGNETIC WAVESEXERCISE - CHAPTER PRACTICE TEST
14 Videos
- MAGNETISM AND MATTERBOOK - MODERN PUBLICATIONCHAPTER - MAGNETISM AND MATTEREXERCISE - CHAPTER PRACTICE TEST FOR BOARD EXAMINATION
16 Videos
Similar Questions
Two point charges +Q each are placed at adjacent corners of a square. Other two point charges -Q each are placed at the remaining two corners of the same square . The side of the square is l. Find electric field intensity at the centre of the square.
View SolutionTwo equal point charges Q=+√2μC are placed at each of the two opposite corners of a square and equal point charges q at each of the other two corners. What must be the value of q so that the resultant force on Q is zero?
View Solution
Knowledge Check
Four charges +Q,−Q,+Q,−Q are placed at the corners of a square taken in order. At the centre of the square
AE=0,V=0BE=0,V≠0CE≠0,V=0DE=0,V≠0
Four identical point charges q are placed at four corners of a square of side a. Find the potential energy of the charge system.
 .
.
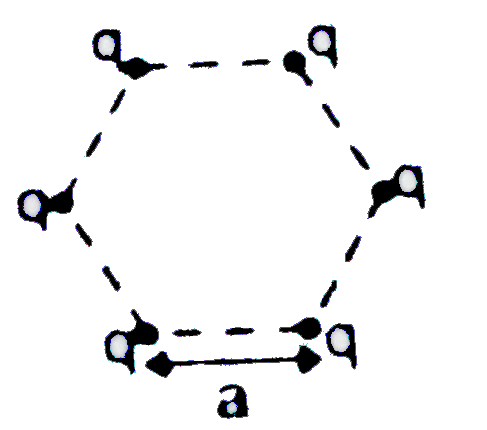
View SolutionSix equal point charges q each are placed at six corners of a hexago of side a. Find out potential energy of charge system
View SolutionTwo identical positive charges are placed at the diagonally opposite corners of a square and two more identical but negative charges are placed at the remaining two corners. What is the work done in putting all charges together at the centre if q is the magnitude of each charge and 2a is the length of each side of the square?
View SolutionFour point charges are placed at the corners of a square ABCD of side 10 cm, as shown in figure. The force on a charge of 1μC placed at the centre of square is

View SolutionFour positive point charges Q are placed at the corners of a square of side a. Calculate the charge that should be placed at the centre so that system remains in equilibrium.
View SolutionFour point charges are placed at the corners of a square of side l. Calculate potential at the centre of square.

View SolutionFour point charges of +1μc each are placed on the four corners of a square of side 1 m. Calculate the electric potential at the centre of the square.
View Solution
