Gaps are left while laying the railway lines in order to allow for expansion. The gap between steel rails of length 65 m is 3.56 cm at 15∘C . Calculate the temperature at which the rails will just touch each other. Take, Osteel=1.1×10−5∘C−1
To solve the problem, we need to calculate the temperature at which the steel rails will just touch each other, given the initial gap and the thermal expansion properties of steel.
1. Identify the Given Data:
- Length of the steel rail (L) = 65 m = 6500 cm (since 1 m = 100 cm)
- Initial gap (ΔL) = 3.56 cm
- Initial temperature (T₀) = 15°C
- Coefficient of linear expansion for steel (α) = 1.1 × 10^(-5) °C^(-1)
2. Convert the Gap into Meters:
- ΔL = 3.56 cm = 3.56 × 10^(-2) m
3. Use the Formula for Linear Expansion:
The change in length due to thermal expansion can be calculated using the formula:
ΔL=L⋅α⋅ΔT
where ΔT is the change in temperature.
4. Rearranging the Formula to Find ΔT:
ΔT=ΔLL⋅α
5. Substituting the Values:
- Substitute ΔL = 3.56 × 10^(-2) m, L = 65 m, and α = 1.1 × 10^(-5) °C^(-1):
ΔT=3.56×10−265⋅1.1×10−5
6. Calculating ΔT:
- Calculate the denominator:
65⋅1.1×10−5=7.15×10−4
- Now calculate ΔT:
ΔT=3.56×10−27.15×10−4≈49.79°C
7. Finding the Final Temperature (T):
- The final temperature at which the rails will just touch each other is:
T=T₀+ΔT=15°C+49.79°C≈64.79°C
Final Answer:
The temperature at which the rails will just touch each other is approximately 64.79°C.
- Length of the steel rail (L) = 65 m = 6500 cm (since 1 m = 100 cm)
- Initial gap (ΔL) = 3.56 cm
- Initial temperature (T₀) = 15°C
- Coefficient of linear expansion for steel (α) = 1.1 × 10^(-5) °C^(-1)
- ΔL = 3.56 cm = 3.56 × 10^(-2) m
The change in length due to thermal expansion can be calculated using the formula:
ΔL=L⋅α⋅ΔT
where ΔT is the change in temperature.
ΔT=ΔLL⋅α
- Substitute ΔL = 3.56 × 10^(-2) m, L = 65 m, and α = 1.1 × 10^(-5) °C^(-1):
ΔT=3.56×10−265⋅1.1×10−5
- Calculate the denominator:
65⋅1.1×10−5=7.15×10−4
- Now calculate ΔT:
ΔT=3.56×10−27.15×10−4≈49.79°C
- The final temperature at which the rails will just touch each other is:
T=T₀+ΔT=15°C+49.79°C≈64.79°C
The temperature at which the rails will just touch each other is approximately 64.79°C.
Topper's Solved these Questions
- THERMAL PROPERTIES OF MATTERBOOK - MODERN PUBLICATIONCHAPTER - THERMAL PROPERTIES OF MATTEREXERCISE - PRACTICE PROBLEM
28 Videos
- THERMAL PROPERTIES OF MATTERBOOK - MODERN PUBLICATIONCHAPTER - THERMAL PROPERTIES OF MATTEREXERCISE - CONCEPTUAL QUESTIONS
16 Videos
- SYSTEMS OF PARTICLES AND ROTATIONAL MOTIONBOOK - MODERN PUBLICATIONCHAPTER - SYSTEMS OF PARTICLES AND ROTATIONAL MOTIONEXERCISE - Chapter Practice Test (for Board Examination)
16 Videos
- UNITS AND MEASUREMENTBOOK - MODERN PUBLICATIONCHAPTER - UNITS AND MEASUREMENTEXERCISE - CHAPTER PRACTICE TEST
15 Videos
Similar Questions
Railway lines are laid with gaps to allow for expansion. If the gap betweeen steel rails 60 m long be 3.60 cm at 10∘C, then at what temperature will the lines just touch? Coefficient of linear expansion of steel =11/10−6.∘C−1.
View SolutionThe scale on a steel on a steel meter stick is calibrated at 15∘C. What is the error in the reading of 60cm at 27∘C ? αsteel=1.2×10−5(.∘C)−1.
View Solution
Knowledge Check
A rod has been laid by using alternate rails of steel and aluminium each 12m long in a winter season when temperature is .−2∘C. The maximum temprature which can must be left be-tween advance rules of they are just to touch on a summer day
(αsteel=1.2×10−5^(0)C−1αAl=2.4×10−5^(0)C−1A0.6cmB1.2cmC0.95cmD0.86cmAt 30∘C, the hole in a steel plate has diameter of 0.099970cm. A cylinder of diameter exactly 1cm at 30∘C is to be slide into the hole. To what temperature the plate must be heated? (Given, αsteel=1.1×10−5∘C−1)
A58∘CB55∘CC57.3∘CD60∘CA heavy brass bar has projections at its ends as shown in the figure. Two find steel wires, fastened between the projections, are just taut (zero tension) when the whole systeam is at 00C. What is the tensile stress in the steel wires when the temperature of the systeam is raised to 3000C?
Given that
αbrass=20×10−6∘C−1
αsteel=12×10−6∘C−1Ysteel=2×1011Nm−2
_E01_077_Q01.png) A48×107Nm−2B84×107Nm−2C32×104Nm−2D24×104Nm−2
A48×107Nm−2B84×107Nm−2C32×104Nm−2D24×104Nm−2
A clock with a steel pendulum gives accurate time at 30∘C . Calculate the fractional loss or gain in time if temperature changes to 50∘C . Coefficient of linear expansion of steel =12×10−6∘C−1
View SolutionA steel metre scale is to be ruled so that the millimetre intervals are accurate to within about 5×10−5mm at a certain temperature. What is the maximum temperature variation allowable during the rulting ? Given α for steel =1.1×10−5.∘C−1.
View SolutionA uniform steel rod of length 50 cm is insulated on its sides. There is a layer of water of thick ness 0.2 mm at each end of the rod. If the ends of the rod are exposed to ice at 0∘C, and steam at 100∘C, calculate the temperature gradient in the rod. Thermal conductivities of steel and water are 0.11calcm−1s−1∘C−1and1.5×10−3calcm−1s−1∘C−1
View SolutionThe brass bar and the alumiumn bar in the drawing are each attached to an immovable wall. At 28∘C the air gap between the rods is 1.3×10−3 m . At what temperature will the be closed ?
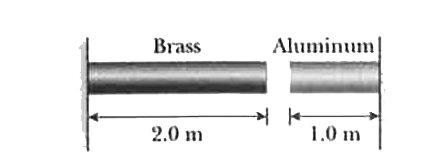
View SolutionIn a certain arrangement, two cylinders of brass and steel are laid side by side and there is a constant difference of length between them at any temperature. Calculate the lengths of these cylinders at 0∘C if there is a difference of 5 cm between their lengths at all temperatures.
αbrass=0.0000019∘C−1
αsteel=1.2×10−6C−1
View Solution
