A solid disc and a ring, both of radius 10cm are placed on a horizontal table simultaneously, with initial angular speed equal to 10πrad/s. Which of the two will start to roll earlier ? The coefficient of kinetic friction is μk=0.2.
As the force of friction produces an acceleration a in the centre of mass.
Therefore equations of motion is μkmg=ma
a=uxg,v=v+at=0+μkgt
The torque due to friction = HimgR which produces an angular retardation α
α=−μkmgRI
As ω=ω0+αt
=ω0−μkmgRtI ...(i)
As u=μkgt
and v=Rω
∴μkgt=Rω
μkgt=Rω0−μkmgR2tI
μkgt=Rω0−μkmgR2tI
t=ω0R2μkg ...(iii)
For a disc, I=12mR2
μkgt)=Rω0−μkmgR2tI
μkgt=Rω0−2μkgt
2μkgt=Rω0
t=Rω03μkg ......(iv)
From (iii) and (iv) we can say that the disc will begin to roll earlier than the ring.
Topper's Solved these Questions
- SYSTEMS OF PARTICLES AND ROTATIONAL MOTIONBOOK - MODERN PUBLICATIONCHAPTER - SYSTEMS OF PARTICLES AND ROTATIONAL MOTIONEXERCISE - NCERT FILE (NCERT EXEMPLAR PROBLEMS (OBJECTIVES QUESTIONS) (MULTIPLE CHOICE QUESTIONS (TYPE-I))
13 Videos
- SYSTEMS OF PARTICLES AND ROTATIONAL MOTIONBOOK - MODERN PUBLICATIONCHAPTER - SYSTEMS OF PARTICLES AND ROTATIONAL MOTIONEXERCISE - NCERT FILE (NCERT EXEMPLAR PROBLEMS (SUBJECTIVES QUESTIONS) (Very Short Answer Type Questions))
9 Videos
- SYSTEMS OF PARTICLES AND ROTATIONAL MOTIONBOOK - MODERN PUBLICATIONCHAPTER - SYSTEMS OF PARTICLES AND ROTATIONAL MOTIONEXERCISE - NCERT FILE ( NCERT TEXTBOOK EXERCISES )
21 Videos
- PHYSICAL WORLD BOOK - MODERN PUBLICATIONCHAPTER - PHYSICAL WORLD EXERCISE - Revision exercises (Long answer questions)
6 Videos
- THERMAL PROPERTIES OF MATTERBOOK - MODERN PUBLICATIONCHAPTER - THERMAL PROPERTIES OF MATTEREXERCISE - CHAPTER PRACTICE TEST
15 Videos
Similar Questions
A cylinder of mass 5kg and radius 10cm is moving on a horizontal surface with speed. 5m/s and angular speed about axis through C.M.10rad/s. Find the angular momentum of the cylinder about point of contact.
View SolutionA small coin of mass 40 g is placed on the horizontal surface of a rotating disc. The disc starts from rest and is given a constant angular acceleration α=2 rad s−2. The coefficient of static friction between the coin and the disc is μs=3/4 and the coefficient of kinetic friction is μk=0.5. The coin is placed at a distance r=1m from the centre of the disc. The magnitude of hte resultant force on the coin exerted by the disc just before it starts slipping on the disc is :

View Solution
Knowledge Check
A ring of radius R is rotating with an angular speed ω0 about a horizontal axis. It is placed on a rough horizontal table. The coefficient of kinetic friction is μk. The time after it starts rolling is.
Aω0μkR2gBω0g2μkRC2ω0RμkgDω0R2μkgA ring of radius R is rotating with an angular speed ω0 about a horizontal axis. It is placed on a rough horizontal table. The coefficient of kinetic friction is μk. The time after it starts rolling is.
Aω0μ1R2gBωaR2μgRC2ω0RωkgDω0R2μggA small coin of mass 80g is placed on the horizontal surface of a rotating disc. The disc starts from rest and is given a constant angular acceleration α=2rad/s2. The coefficient of static friction between the coin and the disc is μs=3/4 and cofficient of kinetic friction is μk=0.5. The coin is placed at a distance r=1m from the centre of the disc. The magnitude of the resultant force on the coin exerted by the disc just before it starts slipping on the disc is
 A0.2NB0.3NC0.4ND1N
A0.2NB0.3NC0.4ND1N
solid sphere of radius r is gently placed on a rough horizontal ground with an initial angular speed ω0 and no linear velocity. If the coefficient of friction is μ, find the time t when the slipping stops. in addition state the linear velocity v and angular velocity ω at the end of slipping
View SolutionA ring of radius 0.5 m and mass 10 kg is rotating about its diameter with angular velocity of 20 rad/s. Its KE is :-
View SolutionA solid sphere of mass 1 kg and radius 10 cm rolls without slipping on a horizontal surface, with a velocity of 20 cm/s. The total kinetic energy of the sphere is
View SolutionA solid sphere of mass 1 kg and radius 10 cm rolls without slipping on a horizontal surface, with a velocity of 20 cm/s. The total kinetic energy of the sphere is
View SolutionA uniform circular ring of radius R is first rotated about its horizontal axis with an angular velocity ω0 and then carefully placed on a rough horizontal surface as shown. The coefficient of friction between the surface and the rings μ. Time after which its angular speed is reduced to 0.5ω0 is
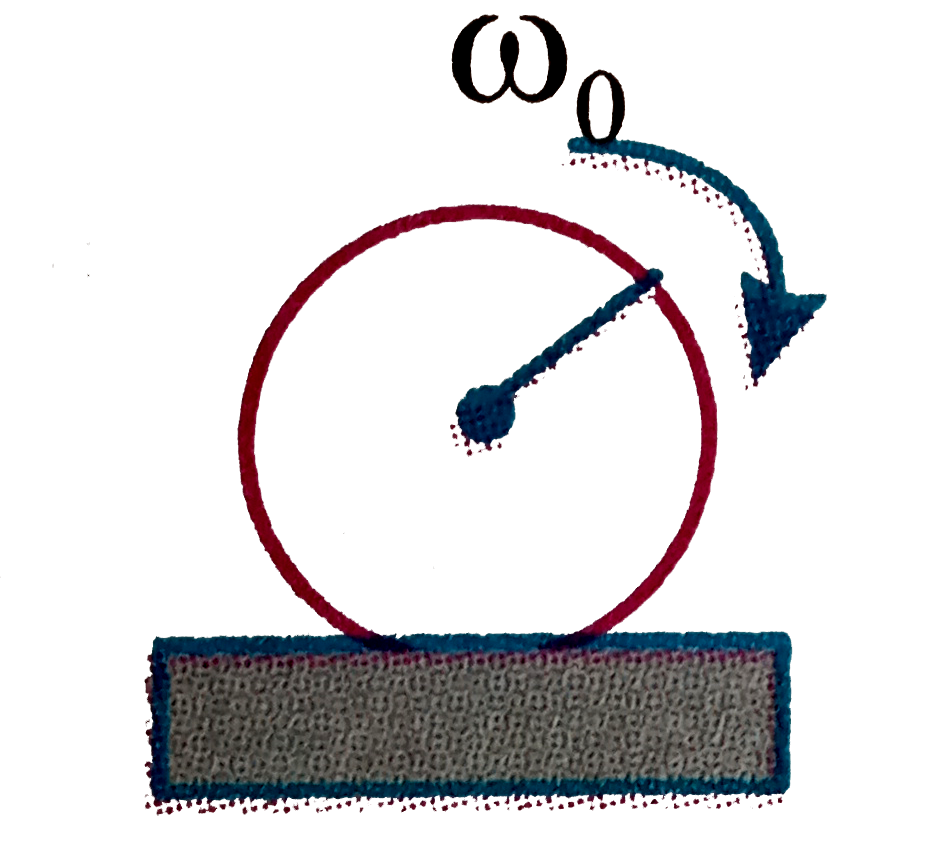
View Solution
