Consider the following equation F=a√x+bt2. If the dimensions of a/b are MpLqTr then calculate value of : -qr
0,1,2,3,4,5,6,7,8,9.
To solve the equation F=a√x+bt2 and determine the values of p,q,r in the dimensions of a and b, we will follow these steps:
Step 1: Identify the dimensions of F
The force F has the dimensions of mass times acceleration, which can be expressed as:
(F)=MLT−2
Step 2: Identify the dimensions of x and t
- The variable x represents a length, so its dimensions are:
(x)=L
- The variable t represents time, so its dimensions are:
(t)=T
Step 3: Determine the dimensions of a
Since F=a√x, we can express the dimensions of a as follows:
√x=√L=L1/2
Thus, the equation becomes:
(F)=(a)⋅(√x)⟹MLT−2=(a)⋅L1/2
Rearranging gives:
(a)=MLT−2L1/2=ML1/2T−2
Step 4: Determine the dimensions of b
Now, consider the term bt2:
t2=T2
Thus, the equation becomes:
(F)=(b)⋅(t2)⟹MLT−2=(b)⋅T2
Rearranging gives:
(b)=MLT−2T2=MLT−4
Step 5: Express the dimensions of a and b
Now we can express the dimensions of a and b:
- For a:
(a)=M1L1/2T−2
- For b:
(b)=M1L1T−4
Step 6: Calculate the dimensions of ab
Now, we can calculate the dimensions of ab:
ab=M1L1/2T−2M1L1T−4=M1−1L1/2−1T−2−(−4)=M0L−1/2T2
This simplifies to:
ab=L−1/2T2
Step 7: Set the dimensions equal to MpLqTr
From the expression L−1/2T2, we can identify:
- p=0
- q=−12
- r=2
Step 8: Calculate −qr
Now, we need to calculate −qr:
−qr=−(−12)(2)=1
Final Answer
Thus, the value of −qr is:
1
The force F has the dimensions of mass times acceleration, which can be expressed as:
(F)=MLT−2
- The variable x represents a length, so its dimensions are:
(x)=L
- The variable t represents time, so its dimensions are:
(t)=T
Since F=a√x, we can express the dimensions of a as follows:
√x=√L=L1/2
Thus, the equation becomes:
(F)=(a)⋅(√x)⟹MLT−2=(a)⋅L1/2
Rearranging gives:
(a)=MLT−2L1/2=ML1/2T−2
Now, consider the term bt2:
t2=T2
Thus, the equation becomes:
(F)=(b)⋅(t2)⟹MLT−2=(b)⋅T2
Rearranging gives:
(b)=MLT−2T2=MLT−4
Now we can express the dimensions of a and b:
- For a:
(a)=M1L1/2T−2
- For b:
(b)=M1L1T−4
Now, we can calculate the dimensions of ab:
ab=M1L1/2T−2M1L1T−4=M1−1L1/2−1T−2−(−4)=M0L−1/2T2
This simplifies to:
ab=L−1/2T2
From the expression L−1/2T2, we can identify:
- p=0
- q=−12
- r=2
Now, we need to calculate −qr:
−qr=−(−12)(2)=1
Thus, the value of −qr is:
1
Topper's Solved these Questions
- UNITS AND MEASUREMENTBOOK - MODERN PUBLICATIONCHAPTER - UNITS AND MEASUREMENTEXERCISE - CHAPTER PRACTICE TEST
15 Videos
- UNITS AND MEASUREMENTBOOK - MODERN PUBLICATIONCHAPTER - UNITS AND MEASUREMENTEXERCISE - COMPETITION FILE (MATRIX MATCH TYPE QUESTIONS)
1 Videos
- THERMAL PROPERTIES OF MATTERBOOK - MODERN PUBLICATIONCHAPTER - THERMAL PROPERTIES OF MATTEREXERCISE - CHAPTER PRACTICE TEST
15 Videos
- WAVESBOOK - MODERN PUBLICATIONCHAPTER - WAVESEXERCISE - CHAPTER PRACTICE TEST
14 Videos
Similar Questions
Knowledge Check
If F denotes force and t time, then in the equation F=at−1+bt2 dimensions of a and b respectively are
A[LT−4] and [LT−1]B[LT−1] and [LT−4]C[MLT−4] and [MLT−1]D[MLT−1] and [MLT−4]Consider the equation of the form x2+px+q=0, then number of such equations that have real roots and have coefficients p and q in the set {1,2,3,4,5,6} (p may be equal to q) is
A18B17C20D19
Consider the following table
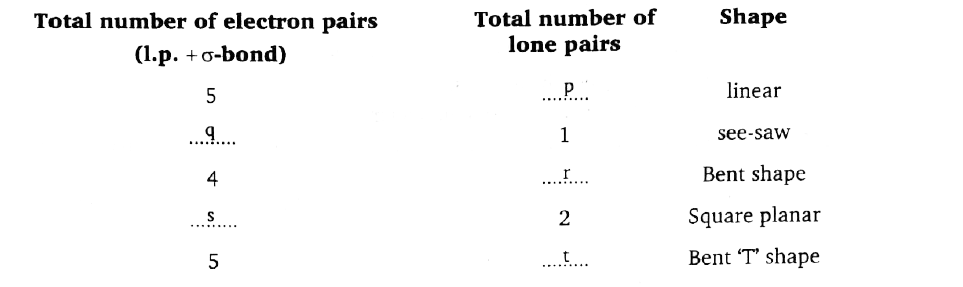
Then calculate value of "p+q+r-s-t".
View SolutionWhat is the dimension of length in moment of inertia? 0,1,2,3,4,5,6,7,8,9.
View SolutionIf P(4,7),Q(7,2)R(a,b) and S(3,8) are the vertices of a parallelogram then find the value of a and b.
View SolutionLet α,β be the roots of the equation x2−px+r=0 and α2,2β be the roots of the equation x2−qx+r=0 , the value of r is (2007, 3M) 29(p−q)(2q−p) (b) 29(q−p(2p−q) 29(q−2p)(2q−p) (d) 29(2p−q)(2q−p)
View SolutionIf y=f(x) satisfies the differential equation (1+x2)f′(x)=x(1-f(x)), f(0)=4/3,thenf∈dthevalueoff(sqrt8)+8/9
View SolutionIf p,q and r are the roots of the equation x3+5x2−16x+48=0 then value of the expression p(qr+q+r)+qr is :
View Solution
