For an ideal gas, the value of (dUdV)T is:
Positive
Zero
Negative
Interchangeable
The correct Answer is:B
Step by step video, text & image solution for For an ideal gas, the value of ((dU)/(dV))_(T) is: by Chemistry experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- THERMODYNAMICS BOOK - ALLENCHAPTER - THERMODYNAMICS EXERCISE - EXERCISE -3
108 Videos
- THERMODYNAMICS BOOK - ALLENCHAPTER - THERMODYNAMICS EXERCISE - EXERCISE -4
18 Videos
- THERMODYNAMICS BOOK - ALLENCHAPTER - THERMODYNAMICS EXERCISE - EXERCISE -1B
22 Videos
- TEST PAPERSBOOK - ALLENCHAPTER - TEST PAPERSEXERCISE - CHEMISTRY
19 Videos
- Thermodynamics And Thermo ChemistryBOOK - ALLENCHAPTER - Thermodynamics And Thermo ChemistryEXERCISE - All Questions
39 Videos
Similar Questions
Knowledge Check
Assertion: CP−CV=R for an ideal gas.
Reason: (∂E∂V)T=0 for an ideal gas.AIf both (A) and (R) are correct and (R) is the correct explanation of (A).BIf both (A) and (R) are correct, but (R) is not the correct explanation of (A).CIf (A) is correct, but (R) is incorrect.DIf (A) is incorrect, but (R) is correct.Assertion: CP−CV=R for an ideal gas.
Reason: (∂E∂V)T=0 for an ideal gas.AStatement 1 is true, statement-2 is true, statement 2 is a correct explanation for statement 6BStatement 1 is true, statement 2 is true, statement 2 is not a correct explanation for statement 6CStatement 1 is true, statement 2 is falseDStatement 1 is false, statement 2 is trueStatement-1: Under adiabatic free expansion, (dUdV)T is +ve when attractive forces are dominant between gas molecules [U,V,T respectively]
Statement-2: Internal energy is a state function.AStatement-1 is true, Statement-2, is true, Statement -2 is a correct explanation for statement-1BStatement-1 is true, Statement-2, is true, Statement -2 is not a correct explanation for statement-1CStatement-1 is true, Statement-2 is falseDStatement-1 is false, Statement-2 is true
For an ideal gas the slope of V-T graph during an adiabatic process is (dV)/(dT) = -matap∮wherevolumeandtemperatureareV_(0)andT_(0).F∈dthevalueofC_(p) of the gas. It is given that m is a positive number.
View SolutionCp−Cv=R and ideal gas
(δUδV)T=0 for an ideal gas .
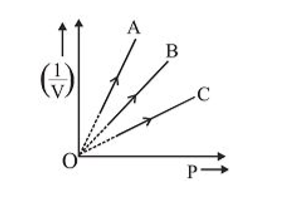
View SolutionFigure shows the isotherms of fixed mass of an ideal gas at three temperatures TA.TB and TC then:-
View SolutionFor one mole of an ideal gas the slope of V versus T curve at constant pressure of 2 atm is X lit mol−1K−1. The value of the ideal universal gas constant 'R' in terms of X is ___________.
View SolutionFor one mole of an ideal gas the slope of V vs. T curve at constant pressure of 2 atm is X lit mol−1K−1. The value of the ideal universal gas constant R in terms of X is
View Solution