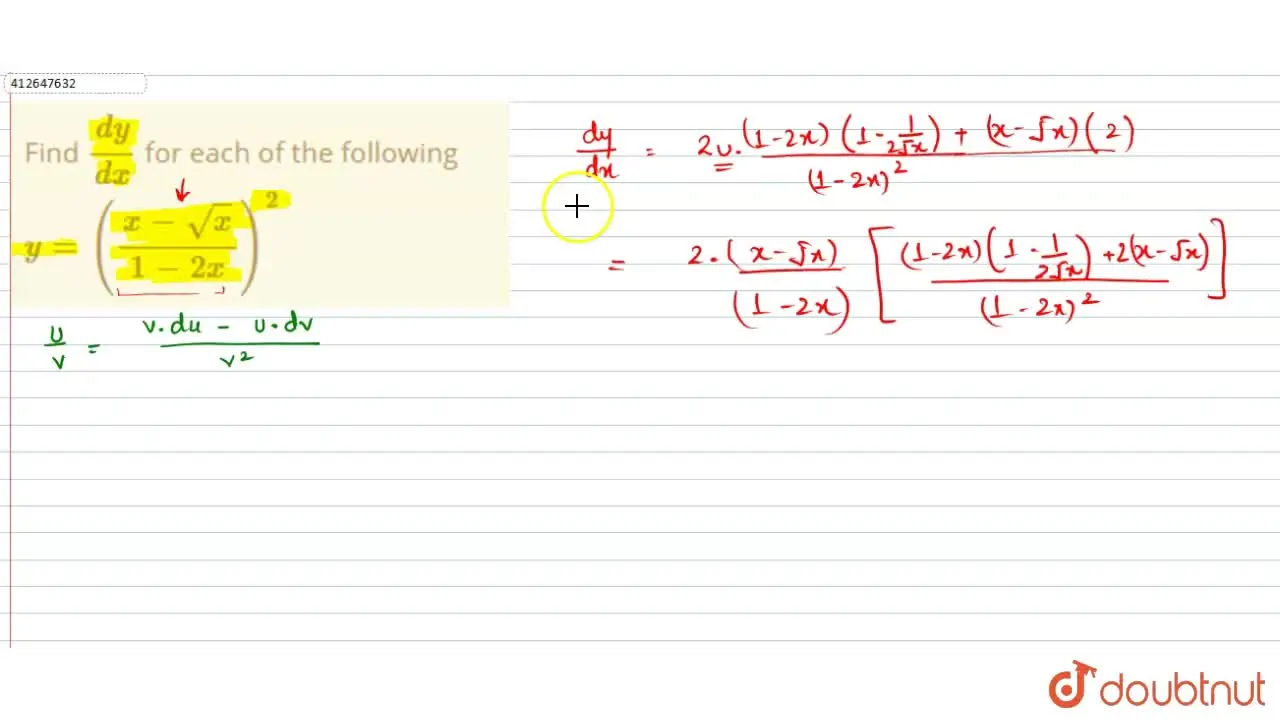
Find dydx for each of the following
y=(x−√x1−2x)2
The correct Answer is:2(x−√x)(1−2x)3[1−√x−12√x];x>0,x≠12
Step by step video, text & image solution for Find dy/dx for each of the following y=((x-sqrtx)/(1-2x))^(2) by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Related Playlists
- BOOK - MODERN PUBLICATIONCHAPTER - CONTINUITY AND DIFFERENTIABILITYEXERCISE - EXERCISE 5(e) (SHORT ANSWER TYPE QUESTIONS)23videos
CONTINUITY AND DIFFERENTIABILITY
- BOOK - MODERN PUBLICATIONCHAPTER - CONTINUITY AND DIFFERENTIABILITYEXERCISE - EXERCISE 5(e) (LONG ANSWER TYPE QUESTIONS (I))22videos
CONTINUITY AND DIFFERENTIABILITY
- BOOK - MODERN PUBLICATIONCHAPTER - CONTINUITY AND DIFFERENTIABILITYEXERCISE - EXERCISE 5(c) (SHORT ANSWER TYPE QUESTIONS)34videos
CONTINUITY AND DIFFERENTIABILITY
- BOOK - MODERN PUBLICATIONCHAPTER - APPLICATIONS OF THE INTEGRALS EXERCISE - CHAPTER TEST12videos
APPLICATIONS OF THE INTEGRALS
- BOOK - MODERN PUBLICATIONCHAPTER - DETERMINANTSEXERCISE - Chapter test 412videos
DETERMINANTS
Similar Questions
Find dydx for each of the following
y=(√x+1√x)(1+x+x2)View SolutionFind dydx for each of the following
y=(x2+3x+5)(x2−2)2View SolutionFind dydx for each of the following
y=3√x2(x2+3).View SolutionFind dydx in each of the following: x23+y23=a23
View SolutionFind dydx for the following functions:y=x+√x+(1√x)
View SolutionFind dydx for the following functions:y=(x−1)(x−2)√x.
View SolutionFind dydx in each of the following: (x2+y2)2=xy
View SolutionFind dydx in the following:y=sin−1(2x√1−x2),−1√2<x<1√2
View SolutionFind dydx in the following
2x+3y=sinxView SolutionFind dydx in the following:2x+3y=siny
View Solution