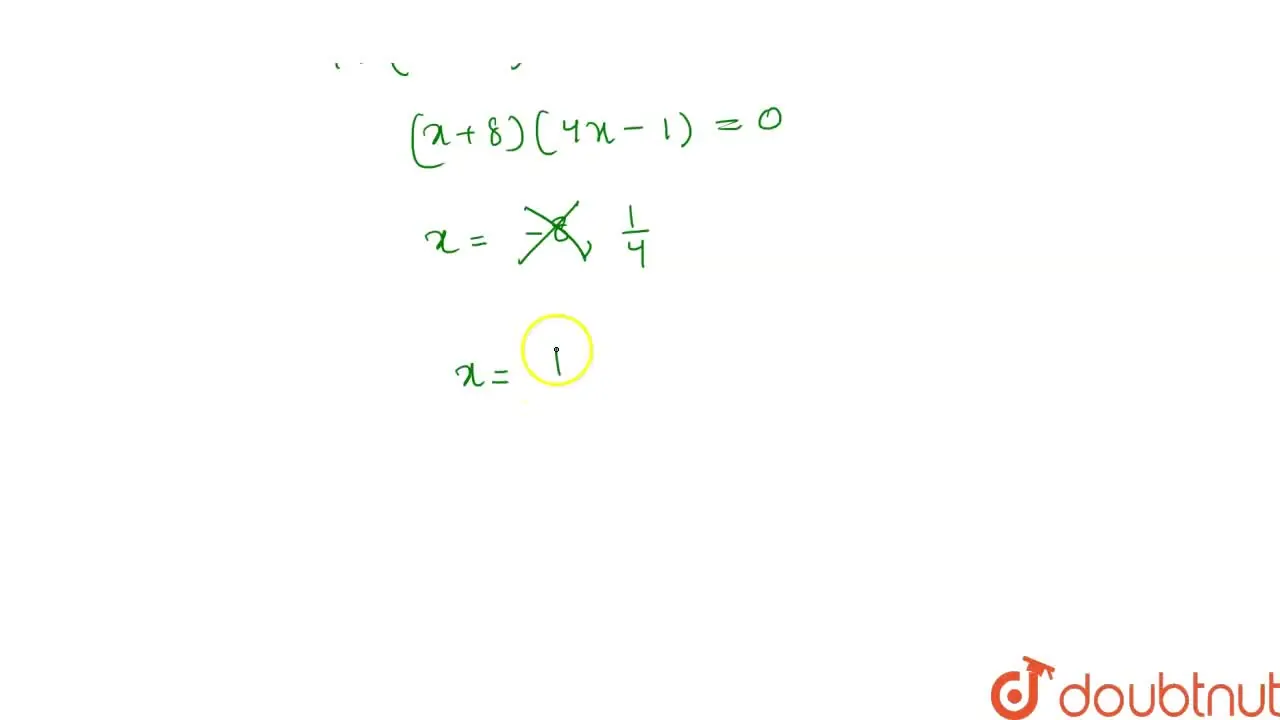Solve the following equations :
tan−1(x+1)+tan−1(x−1)=tan−1(831),x>0
The correct Answer is:14
Step by step video, text & image solution for Solve the following equations : tan^(-1) (x+1) +tan^(-1) (x-1) = tan^(-1) (8/31 ) , x gt 0 by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Related Playlists
- BOOK - MODERN PUBLICATIONCHAPTER - INVERSE - TRIGONOMETRIC FUNCTIONSEXERCISE - EXERCISE 2 (b) (Long Answer Type Questions (II))2videos
INVERSE - TRIGONOMETRIC FUNCTIONS
- BOOK - MODERN PUBLICATIONCHAPTER - INVERSE - TRIGONOMETRIC FUNCTIONSEXERCISE - Objective Type Questions (A. Multiple Choice Questions)26videos
INVERSE - TRIGONOMETRIC FUNCTIONS
- BOOK - MODERN PUBLICATIONCHAPTER - INVERSE - TRIGONOMETRIC FUNCTIONSEXERCISE - EXERCISE 2 (a) (Long Answer Type Questions )3videos
INVERSE - TRIGONOMETRIC FUNCTIONS
- BOOK - MODERN PUBLICATIONCHAPTER - INTEGRALSEXERCISE - COMPETITION FILE24videos
INTEGRALS
- BOOK - MODERN PUBLICATIONCHAPTER - LINEAR PROGRAMMING EXERCISE - Chapter Test12videos
LINEAR PROGRAMMING
Similar Questions
Solve the following equations :
tan−1(x+2)+tan−1(x−2)=tan−1(879)View SolutionSolve the following equations :
tan−1(x+2)+tan−1(x−2)=π4,x>0View SolutionIf tan−1(x+1)+tan−1(x−1)=tan−1(831),then x is equal to
View SolutionSolve the following equations :
2tan−1(sinx)=tan−1(2secx,)0<x<π2View SolutionSolve for x: tan−1(x+1)+tan−1(x−1)=tan−1(831)
View SolutionSolve the following equations :
tan−1(x−2x−4)+tan−1(x+2x+4)=π4View SolutionSolve tan−1x+sin−1x=tan−12x
View SolutionSolve the following system of inequations: 4(tan−1x)2−8tan−1x+3<0&4cot−1x+3<0&4cot−1x−(cot−1x)2−3≥0
View SolutionSolve the following inequalities
tan2(arcsinx)>1View SolutionSolve the following equations :
tan−1.2x1−x2+cos−1.1−x22x=π3,x>0View Solution