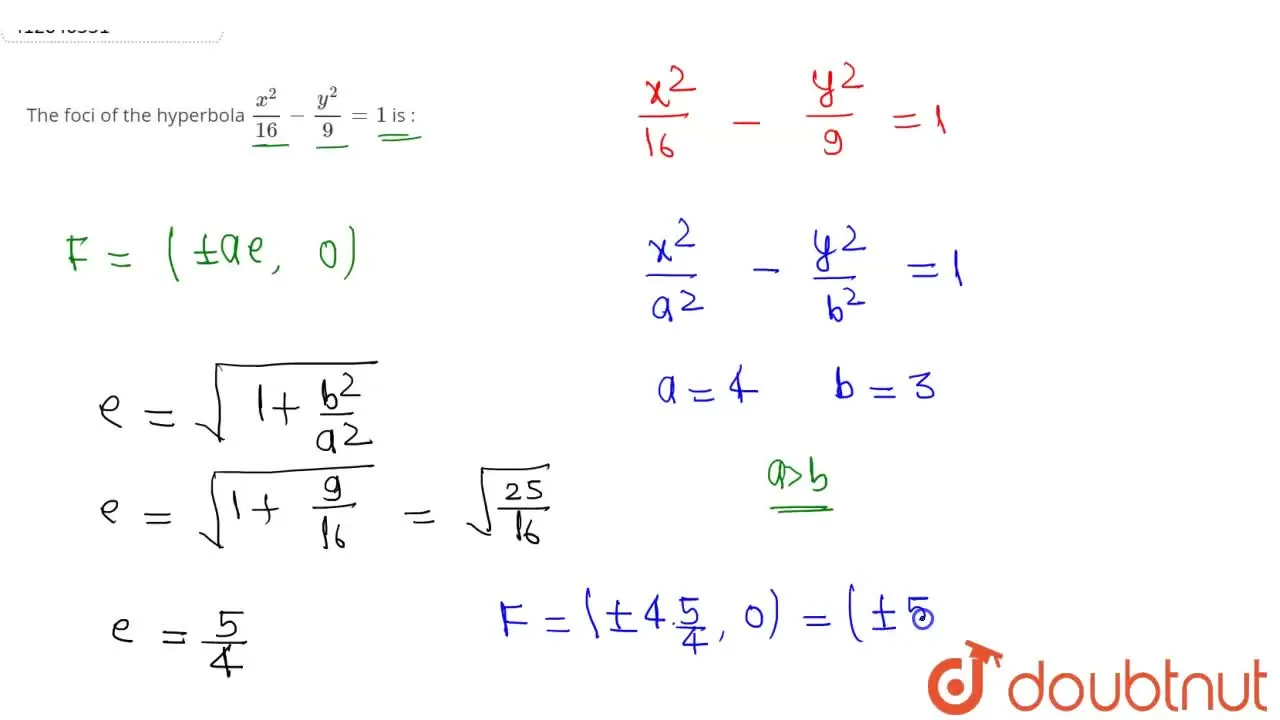
The foci of the hyperbola x216−y29=1 is :
(0,5)
(5,0)
(±5,0)
(0,±5)
The correct Answer is:C
Step by step video, text & image solution for The foci of the hyperbola (x^(2))/(16) -(y^(2))/(9) = 1 is : by Maths experts to help you in doubts & scoring excellent marks in Class 11 exams.
Related Playlists
- BOOK - MODERN PUBLICATIONCHAPTER - CONIC SECTIONS EXERCISE - OBJECTIVE TYPE QUESTIONS (FILL IN THE BLANKS)10videos
CONIC SECTIONS
- BOOK - MODERN PUBLICATIONCHAPTER - CONIC SECTIONS EXERCISE - OBJECTIVE TYPE QUESTIONS (TRUE/FALSE QUESTIONS)5videos
CONIC SECTIONS
- BOOK - MODERN PUBLICATIONCHAPTER - CONIC SECTIONS EXERCISE - EXERCISE 11 (H) (LONG ANSWER TYPE QUESTIONS I )6videos
CONIC SECTIONS
- BOOK - MODERN PUBLICATIONCHAPTER - COMPLEX NUMBERSEXERCISE - CHAPTER TEST12videos
COMPLEX NUMBERS
- BOOK - MODERN PUBLICATIONCHAPTER - INTRODUCTION TO THREE DIMENSIONAL GEOMETRYEXERCISE - CHAPTER TEST12videos
INTRODUCTION TO THREE DIMENSIONAL GEOMETRY
Similar Questions
The circle described on the line joining the foci of the hyperbola x216−y29 = 1 as a diameter passes through an end of the latus rectum of the parabola y2=4ax, the length of the latus rectum of the parabola is
View SolutionThe foci of the hyperbola x29+y216=1 are-
View SolutionThe foci of the hyperbola 4x2−9y2−1=0 are
View SolutionThe foci of the hyperbola 4x2−9y2−1=0 are
View SolutionFind the coordinates of the foci, the vertices, the eccentricity and the length of the latus rectum of the Hyperbola x216−y29=1
View SolutionFind the coordinates of the foci and the vertices, the eccentricity and the length of the latus rectum of the hyperbolas.x216−y29=1
View SolutionThe foci of the hyperbola 4x2−9y2−1=0 are:
View SolutionThe foci of the hyperbola 4x2−9y2−36=0 are
View SolutionThe eccentricity of the hyperbola x216+y29=1 will be-
View SolutionIf the latus rectum of the hyperbola x216−y2b2=1 is 92 , then its eccentricity, is
View Solution