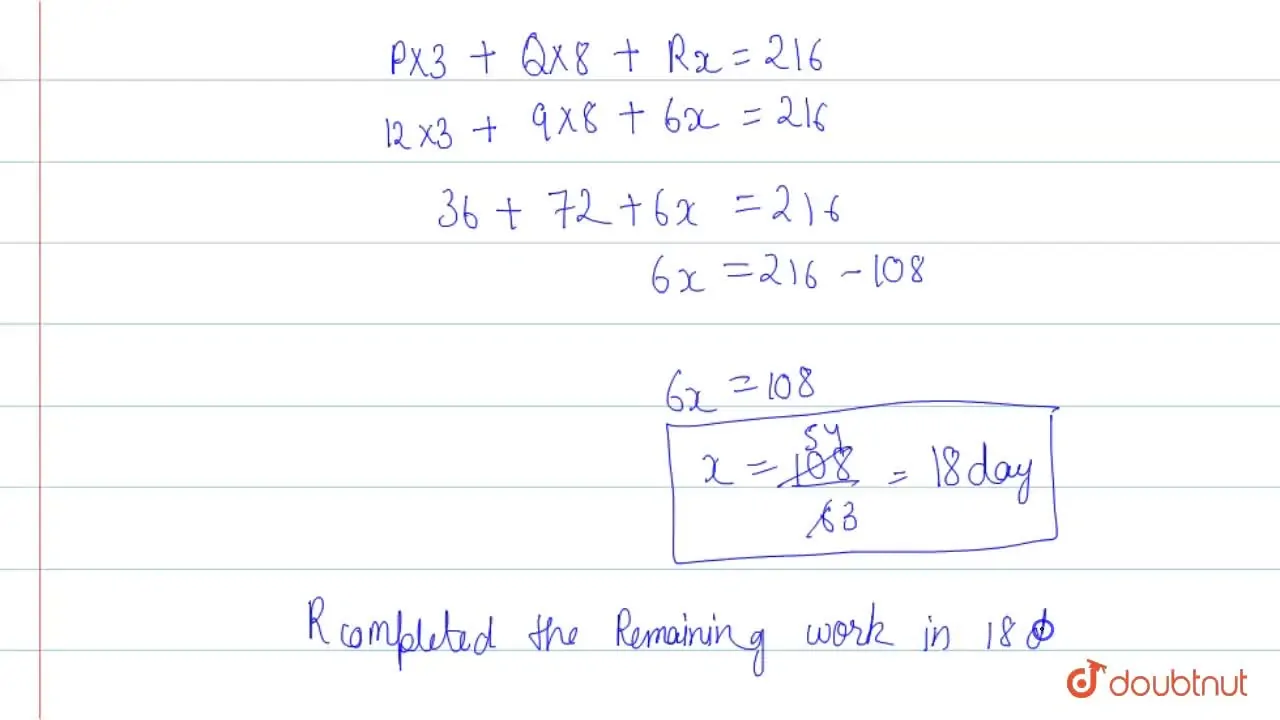
P,Q and R can complete a job in 18 days,24 days and 36 days respectively. P started it and worked for 3 days. P then left Q then worked on it for 8 days. Q then left. R completed the remaining part of the job in 12 days. Find the time for which R worked (in days).
12
18
4
27
The correct Answer is:B
Part of the job that P compound =3
(118)=16
Part of it that Q completed=8(124)=13
Part of it that R completed
=1−(16+13)=12
Time for which R worked (in days)
=12(36)=18.
Related Playlists
- BOOK - PEARSON IIT JEE FOUNDATIONCHAPTER - TIME AND WORK, PIPES AND CISTERNSEXERCISE - CONCEPT APPLICATION (LEVEL-2)20videos
TIME AND WORK, PIPES AND CISTERNS
- BOOK - PEARSON IIT JEE FOUNDATIONCHAPTER - TIME AND WORK PIPES AND CISTERNSEXERCISE - CONCEPT APPLICATION (LEVEL-3)7videos
TIME AND WORK PIPES AND CISTERNS
Similar Questions
P,Q and R can complete a job in 20 days, 30 and 40 days respectiely. They started working on it. Q and R left afte working for certain number of days and P alone completed the remaing work 7 days. Find the time taken to complete the job (in days)
View SolutionA can complete a work in 18 days, B in 20 days and C in 30 days. B and C together start the work but after 2 days, they left the work. How many days will a take to complete the remaining work ?
View SolutionA, B and C can individually complete a task in 24 days, 16 days and 32 days respectively. If A and C start the work and worked for 6 days and left, then the number of days required by B to complete the remaining task is:
A, B और C व्यक्तिगत रूप से किसी कार्य को क्रमशः 24, 16 और 32 दिनों में पूरा कर सकते हैं | यदि A और C ने कार्य शुरू किया तथा 6 दिनों तक कार्य करके छोड़ दिया, तो शेष कार्य पूरा करने में B को कितने दिन लगेंगे ?View SolutionA, B and C can individually complete a task in 24 days, 16 days and 32 days respectively. If A and C started the work and worked for 6 days and left, then the number of days required by B to complete the remaining task is :
View SolutionP,Q and R can complete a work in 12,24 and 6 days respectively. The work will be completed in __ days if all of them are working together.
View SolutionA, B and C can individually complete a piece of work in 24 days, 15 days and 12 days respectively. B and C started the work and worked for 3 days and left. The number of days required by A alone to complete the remaining work is:
A, B और C अकेले-अकेले किसी कार्य को क्रमशः 24, 15 और 12 दिनों में पूरा कर सकते हैं |B और C ने कार्य करना शुरू किया तथा 3 दिनों तक कार्य करके छोड़ दिया| शेष कार्य अकेले पूरा करने में A को कितने दिन लगेंगे ?View SolutionA, B and C can individually complete a piece of work in 24 days, 15 days and 12 days. respectively. B and C started the work and worked for 3 days and left. The number of days required by A alone to complete the remaining work is :
View SolutionP,Q and R can complete a job in 6 days, 9 days and 12 days, respectively. They worked together and completed it. They earned a total of Rs. 2600. Find P's share ( in Rs.)
View SolutionP, Q and R alone can complete a work in 12. 15 and 20 days respectively. In how many days will they together complete the same work?
View SolutionP, Q and R alone can complete a work in 12. 15 and 20 days respectively. In how many days will they together complete the same work?
View Solution