Two cells of internal resistance $r_{1} and r_{2}$ and of same emf are connected in series across a resistor of resistance R. If the terminal potential difference across the call of internal resistance $r_{1}$ is zero , then the value of R is Solution in Kannada

A

$R = 2 (r_{1} + r_{2})$

B

$R = r_{2} - r_{1}$

C

$R = r_{1} - r_{2}$

D

$R = 2 (r_{1} - r_{2})$

Video Solution

Text Solution

Verified by Experts

The correct Answer is:C

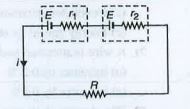
Let the emf of each cell be $ε$ .
The cells are connected in series across R as shown in figure .
Since the cells are in series , so their equivalent emf is

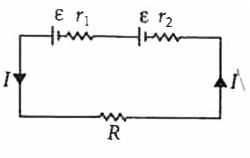
$ε_{e p} = ε + ε = 2 ε$
and their equivalent internal resistance is
$r_{e q} = r_{1} + r_{2}$
The current in the circuit is
$I = \frac{ε_{e q}}{r_{e q} + R} = \frac{2 ε}{r_{1} + r_{2} + R} ... (i)$
The terminal potential difference across the cell of internal resistance $r_{1}$ is
$V_{1} = ε = I r_{1}$
But as per question $V_{1} = 0$
$∴ 0 = ε - I r_{1} or I = \frac{ε}{r_{1}} ... (i i)$
Equating (i) and (ii) , we get
$\frac{2 ε}{r_{1} + r_{2} + R} = \frac{ε}{r_{1}} or 2 r_{1} = r_{1} + r_{2} + R$
or, $R = 2 r_{!} - r_{1} - r_{2} = r_{1} - r_{2}$

Updated on:21/07/2023

|

Class 12 PHYSICS QUESTION BANK

Topper's Solved these Questions

Similar Questions

KCET PREVIOUS YEAR PAPERS-KARNATAKA CET 2018-PHYSICS

Home
Ask Doubt
Profile