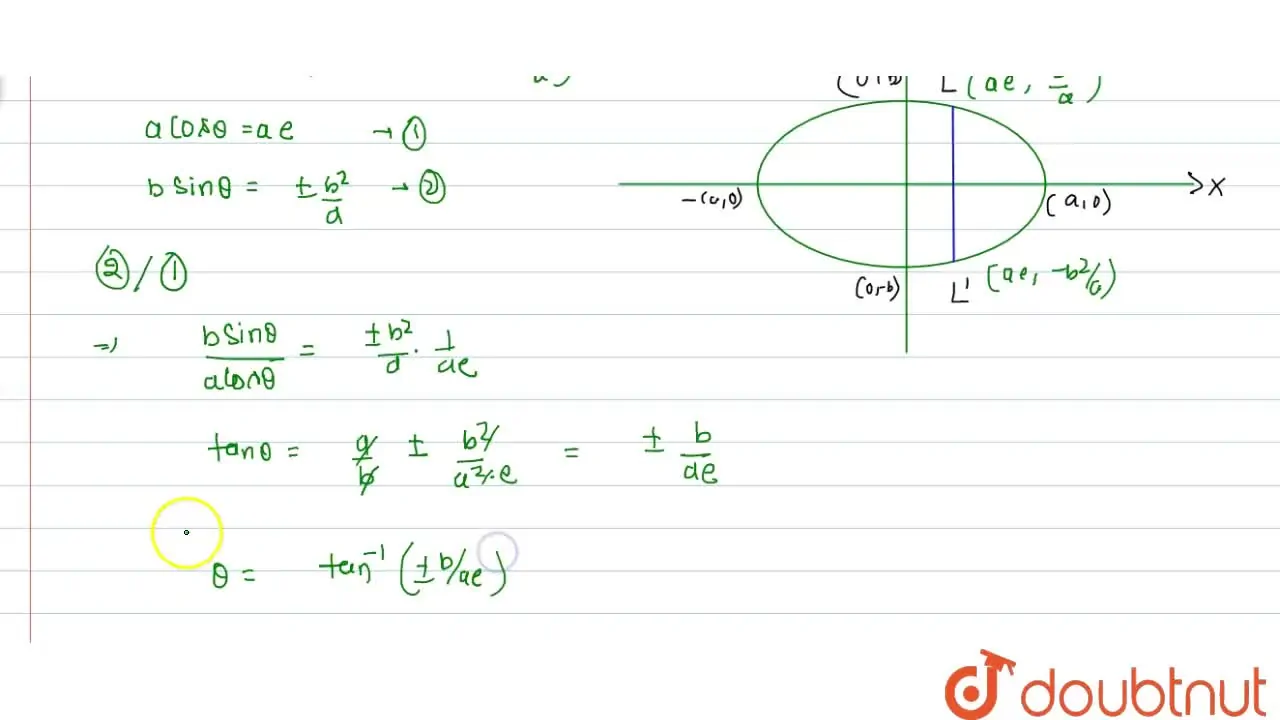
Find the eccentric angles of the extremities of the latus recta of the ellipse x2a2+y2b2=1
Step by step video & image solution for Find the eccentric angles of the extremities of the latus recta of the ellipse (x^2)/(a^2)+(y^2)/(b^2)=1 by Maths experts to help you in doubts & scoring excellent marks in Class 11 exams.
Similar Questions
The eccentric angles of the extremities of latus-rectum of the ellipse x2a2+y2b2=1 are given by
View SolutionIf θ1,θ2 are the eccentric angles of the extremities of a focal chord of the ellipse x2a2+y2b2=1(a<b)ande is the eccentricity then show that e+1e−1=cot(θ12)cot(θ22)
View SolutionFind the eccentricity and the length of the latus rectum of the ellipsex24+y225=1.
View SolutionFind the area of the region bounded by the latus recta of the ellipse x2a2+y2b2=1 and the targets to the ellipse drawn at their ends
View SolutionFind the eccentricity and the length of the latus rectum of the ellipse x249+y236=1.
View SolutionFind the area of parallelogram formed by tangents at the extremities of latera recta of the ellipse x2a2+y2b2=1
View SolutionThe locus of extremities of the latus rectum of the family of ellipse b2x2+a2y2=a2b2 is
View SolutionThe eccentric angles of extremities of a chord of an ellipse x2a2+y2b2=1 are θ1 and θ2 .If this chord passes through the foucs, then
View SolutionFind the eccentricity and length of the latus rectum of the ellipse x2+2y2=3
View SolutionFind the eccentricity of the ellipse x24=y225=1.
View Solution