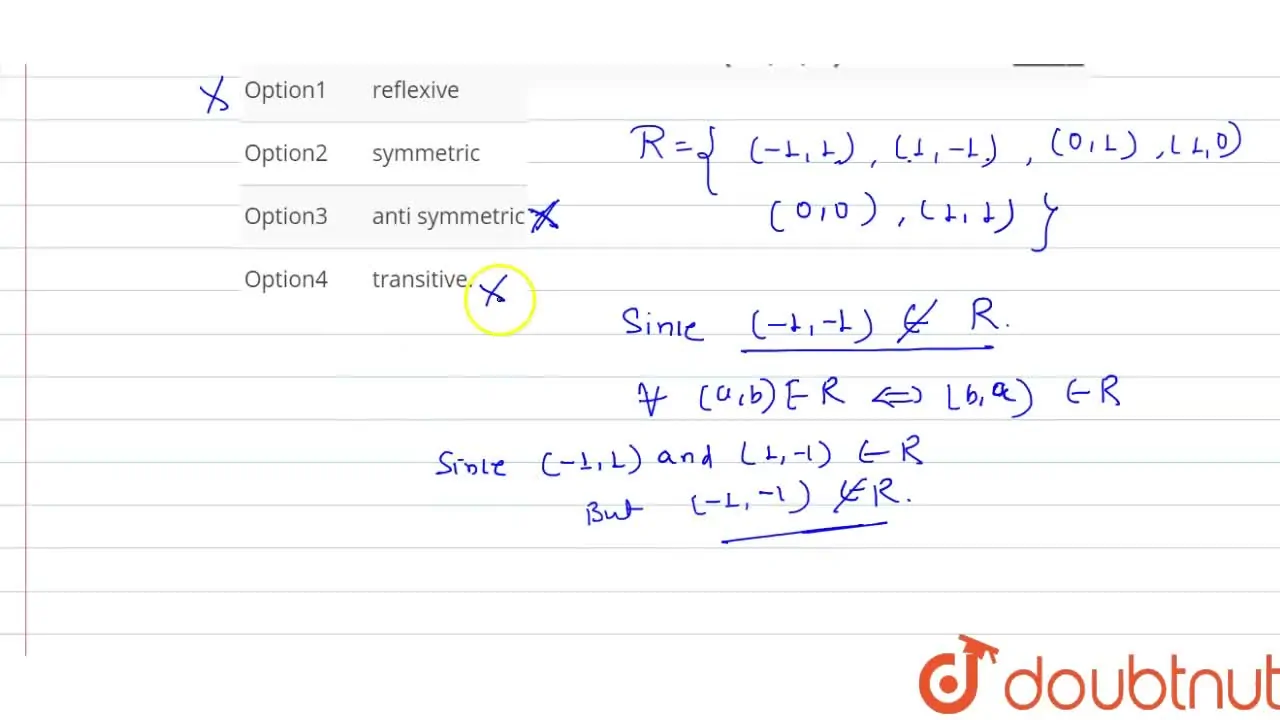
If R={(a,b)/|a+b|=a+b} is a relation defined on a set (-1,0,1) then R is____
reflexive
symmetric
anti symmetric
transitive.
The correct Answer is:B
(i) Verify the reflexive symmetric and transitive properties of relations.
(ii) R={(0,0),(1,1),(1,-1),(01,1),(0,1),(1,0)}.
(iii) Check which properties does R satisfy
Related Playlists
- BOOK - PEARSON IIT JEE FOUNDATIONCHAPTER - SETS AND RELATIONSEXERCISE - LEVEL 38videos
SETS AND RELATIONS
- BOOK - PEARSON IIT JEE FOUNDATIONCHAPTER - SETS AND RELATIONSEXERCISE - LEVEL 129videos
SETS AND RELATIONS
- BOOK - PEARSON IIT JEE FOUNDATIONCHAPTER - SALES TAX AND COST OF LIVING INDEX EXERCISE - Level 314videos
SALES TAX AND COST OF LIVING INDEX
- BOOK - PEARSON IIT JEE FOUNDATIONCHAPTER - SHARES AND DIVIDENDSEXERCISE - CONCEPT APPLICATION (LEVEL 3)10videos
SHARES AND DIVIDENDS
Similar Questions
If R={(a,b)/|a+b|=|a|+|b|} is a relation on a set {−1,0,1} then R is_
View SolutionIf R={(a,b)//a+b=4} is a relation on N, then R is____
View SolutionIf R is a relation defined as aRb ,iff |a−b|>0 then the relation is
View SolutionIf R is a relation defined as aRb, if|a−b|>0, then the relation is
View SolutionLet A={a,b,c} and B={1,2}. Consider a relation R defined from st A to set B. Then R can equal to set
View SolutionLet A = {a,b,c} and B = {4, 5}. Consider a relation R defined from set A to set B, then R can be equal to set
View SolutionLet S be the set of integers. For a,b∈S relation R is defined by aRb If |a−b|<1 then R is
View SolutionLet A={a,b,c}andB={1,2,3,4}. Consider a relation R defined from set A to set B. Then R is a subset of set
View Solutionshow that R is an equivaence relation R defined on the set S={1,2,3,4,5} given by R={ab|a−b∣ is even } is an equivalence relation .
View SolutionLet S be set of all numbers and let R be a relation on S defined by aRb⇔a2+b2=1 then, R is
View Solution