The sides of two similar triangles are in the ratio 2 : 3 , then their areas are in the ratio ………… Solution in Tamil
The correct Answer is:B
Step by step video, text & image solution for The sides of two similar triangles are in the ratio 2 : 3 , then their areas are in the ratio ………… by Maths experts to help you in doubts & scoring excellent marks in Class 10 exams.
Updated on:21/07/2023Topper's Solved these Questions
- GEOMETRYBOOK - FULL MARKSCHAPTER - GEOMETRYEXERCISE - ADDITIONAL QUESTIONS SOLVED (ANSWER THE FOLLOWING QUESTIONS)
24 Videos
- GEOMETRYBOOK - FULL MARKSCHAPTER - GEOMETRYEXERCISE - THINKING CORNER
2 Videos
- GEOMETRYBOOK - FULL MARKSCHAPTER - GEOMETRYEXERCISE - UNIT EXERCISE 4
10 Videos
- DEPARTMENTAL MODEL PAPER BOOK - FULL MARKSCHAPTER - DEPARTMENTAL MODEL PAPER EXERCISE - PART-IV
3 Videos
- MENSURATION BOOK - FULL MARKSCHAPTER - MENSURATION EXERCISE - ADDITIONAL QUESTIONS SOLVED (iii)
8 Videos
Similar Questions
The image of man height 1.8m , is of length 1.5 cm on the film of a camera . If the film is 3 cm from the camera, how far is the man from the camera ?
View SolutionCan we draw two tangents parallel to each other on a circle ?
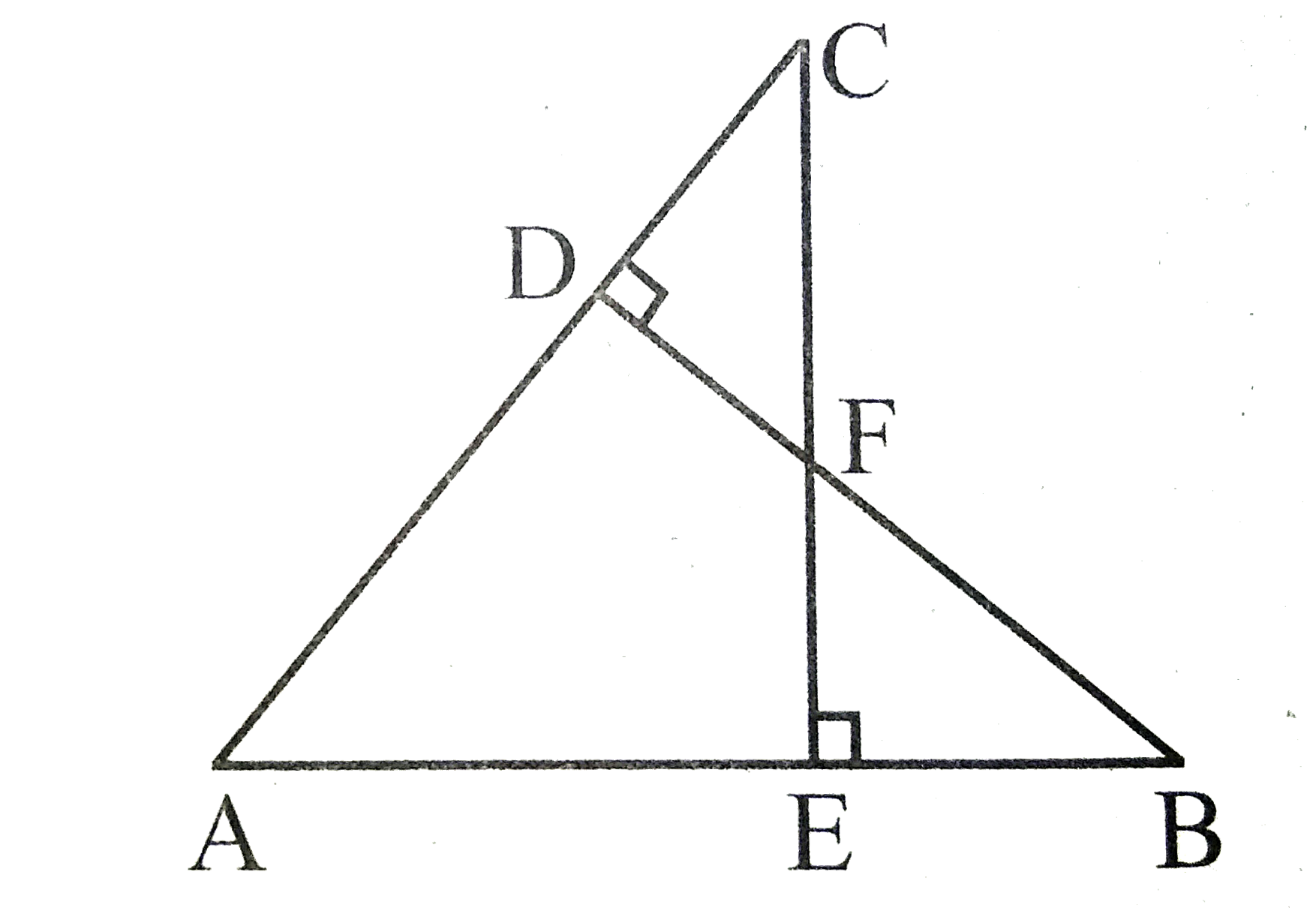
View SolutionIn the figure, if BD⊥ACandCE⊥AB , prove that
(i)ΔAEC~ΔADB (ii)CAAB=CEDB
View SolutionPQ is a chord of length 8 cm of a circle of radius 5 cm. The tangents at P and Q intersect at a point T . Find the length TP .
View SolutionA vessel is in the form of a hemispherical bowl mounted by a hollow cylinder. The diameter is 14 cm and the height of the vessel is 13 cm. Find the capacity of the vessel.
View SolutionThe sides of two similar triangles are in the ratio 2:3, then the areas of these triangles are in the ratio ________.
View SolutionSides of two similar triangles are in the ratio 3:5, Areas of these triangles are in the ratio..
View SolutionThe ratio of the corresponding sides of two similar triangles is 5:3. Then the ratio of their areas………..
View SolutionIF the sides of two similar triangles are in the ratio 7:2 then the ratio of their areas is………..
View SolutionSides of two similar triangles are in the ratio 4 : 9 Areas of these triangles are in the ratio
View SolutionArea of two similar triangles are in the ratio of 5:3 then the ratio of their corresponding sides is :
View SolutionIf the ratio of the sides of two similar triangles is 3 : 5, then the ratio of their areas is :
View SolutionIf the ratio of sides of two similar triangles is 2:5, then the ratio of their areas will be :
View SolutionAreas of two similar triangles are in the ratio 64:121, then the sides of these triangles are in the ratio:
View SolutionThe sides of two similar triangles are in the ratio 3:7. The ratio of areas of these triangles will be :
View Solution
