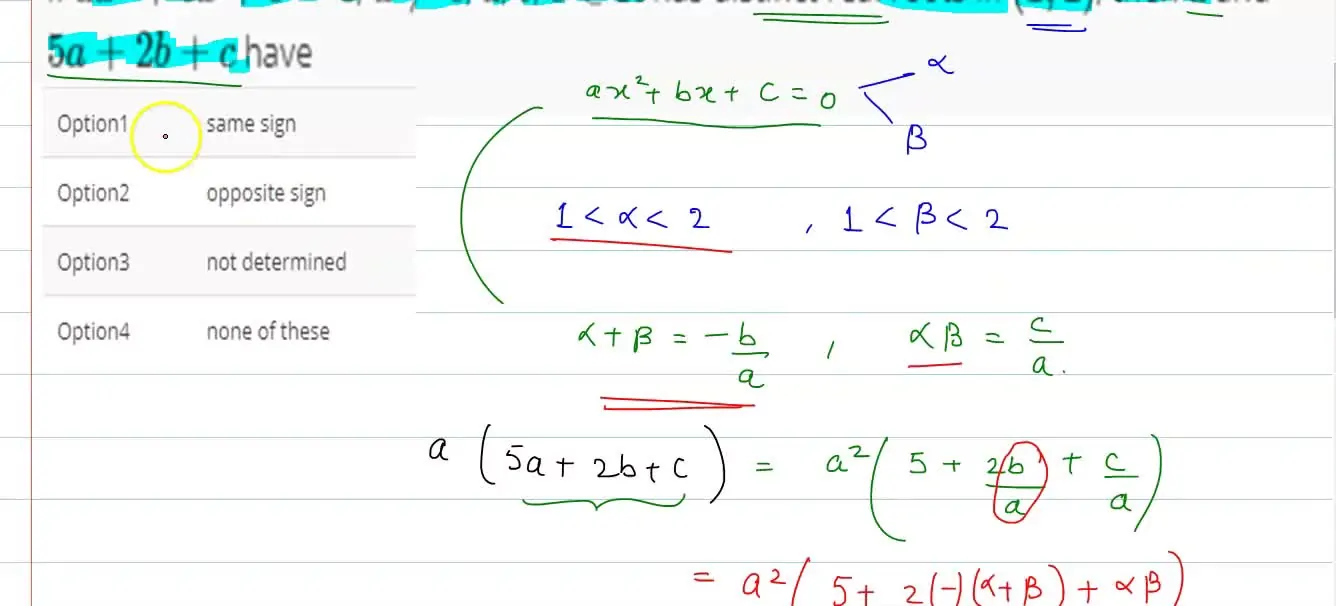
If , , , , has distinct real roots in , then and have
same sign
opposite sign
not determined
none of these
The correct Answer is:A
Let and be two roots of
and
Now,
Hence, and are of same sign.
Related Playlists
- BOOK - CENGAGECHAPTER - THEORY OF EQUATIONSEXERCISE - Multiple Correct Answer6videos
THEORY OF EQUATIONS
- BOOK - CENGAGECHAPTER - THEORY OF EQUATIONSEXERCISE - Comprehension12videos
THEORY OF EQUATIONS
- BOOK - CENGAGECHAPTER - STRAIGHT LINESEXERCISE - JEE Advanced Previous Year4videos
STRAIGHT LINES
- BOOK - CENGAGECHAPTER - THREE DIMENSIONAL GEOMETRYEXERCISE - Question Bank20videos
THREE DIMENSIONAL GEOMETRY
Similar Questions
If ax2+bx+c=0, a≠0, a,b,c∈R has distinct real roots in ( 1,2) then a and 5a + 2b + c have (A) same sign (B) opposite sign (C) not determined (D) none of these
View SolutionIf ax2+bx+c=0 and cx2+bx+a=0(a,b,c∈R) have a common non - real root, then
View SolutionConsider the quadrationax2−bx+c=0,a,b,c∈N which has two distinct real roots belonging to the interval (1,2).
The least value of b isView SolutionConsider the quadrationax2−bx+c=0,a,b,c∈N which has two distinct real roots belonging to the interval (1,2).
The least value of c isView SolutionConsider the quadrationax2−bx+c=0,a,b,c∈N which has two distinct real roots belonging to the interval (1,2).
The least value of a isView SolutionIf ax2+bx+c=0,a,b,c∈R has no real zeros, and if c<0 , then which of the following is true? a<0 a+b+c>0 a>0
View SolutionIf ax2+bx+c=0 has no real roots and a, b, c ∈ R such that a+c>0, then
View SolutionIf ax2+bx+c,a,b,c∈R,a≠0 has real zero and a−b+c<0 , then value of ac is
View SolutionIf a,b,c ∈Randax2+bx+c=0 has no real roots, then
View SolutionIf a,b∈R and ax2+bx+6=0,a≠0 does not have two distinct real roots, then :
View Solution