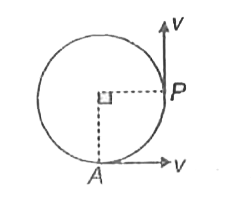
A particle of mass 2 kg moves on a circular path with constant speed 10 m/ s . Find change in speed and magnitude of change in velocity. Whan particle completes half revolution.
To solve the problem step by step, let's break it down:
Step 1: Understand the Problem
We have a particle of mass 2 kg moving in a circular path with a constant speed of 10 m/s. We need to find the change in speed and the magnitude of the change in velocity when the particle completes half a revolution.
Step 2: Analyze Change in Speed
Since the particle is moving with a constant speed, the speed at any point during its motion remains the same. Therefore, the speed at the starting point (Point A) and the speed at the point after half a revolution (Point B) will both be 10 m/s.
- Speed at Point A (V1) = 10 m/s
- Speed at Point B (V2) = 10 m/s
Change in Speed:
ΔSpeed=V2−V1=10m/s−10m/s=0m/s
Step 3: Analyze Change in Velocity
Velocity is a vector quantity, which means it has both magnitude and direction. While the speed remains constant, the direction of the velocity changes as the particle moves along the circular path.
- At Point A, let's assume the velocity vector is directed along the positive x-axis:
→V1=10^im/s
- After completing half a revolution, at Point B, the velocity vector will be directed along the negative x-axis:
→V2=−10^im/s
Change in Velocity:
Δ→V=→V2−→V1=(−10^i)−(10^i)=−20^im/s
Step 4: Calculate Magnitude of Change in Velocity
The magnitude of the change in velocity is given by the absolute value of the change in velocity vector:
|Δ→V|=|−20^i|=20m/s
Final Answers
- Change in Speed: 0m/s
- Magnitude of Change in Velocity: 20m/s
We have a particle of mass 2 kg moving in a circular path with a constant speed of 10 m/s. We need to find the change in speed and the magnitude of the change in velocity when the particle completes half a revolution.
Since the particle is moving with a constant speed, the speed at any point during its motion remains the same. Therefore, the speed at the starting point (Point A) and the speed at the point after half a revolution (Point B) will both be 10 m/s.
- Speed at Point B (V2) = 10 m/s
ΔSpeed=V2−V1=10m/s−10m/s=0m/s
Velocity is a vector quantity, which means it has both magnitude and direction. While the speed remains constant, the direction of the velocity changes as the particle moves along the circular path.
→V1=10^im/s
→V2=−10^im/s
Δ→V=→V2−→V1=(−10^i)−(10^i)=−20^im/s
The magnitude of the change in velocity is given by the absolute value of the change in velocity vector:
|Δ→V|=|−20^i|=20m/s
- Change in Speed: 0m/s
- Magnitude of Change in Velocity: 20m/s
Topper's Solved these Questions
- KINEMATICS (MOTION ALONG A STRAIGHT LINE AND MOTION IN A PLANE)Book:ALLENChapter:KINEMATICS (MOTION ALONG A STRAIGHT LINE AND MOTION IN A PLANE)Exercise:BEGINNER S BOX-3
Explore 2 Videos
- KINEMATICS (MOTION ALONG A STRAIGHT LINE AND MOTION IN A PLANE)Book:ALLENChapter:KINEMATICS (MOTION ALONG A STRAIGHT LINE AND MOTION IN A PLANE)Exercise:BEGINNER S BOX-4
Explore 6 Videos
- KINEMATICS (MOTION ALONG A STRAIGHT LINE AND MOTION IN A PLANE)Book:ALLENChapter:KINEMATICS (MOTION ALONG A STRAIGHT LINE AND MOTION IN A PLANE)Exercise:BEGINNER S BOX-1
Explore 6 Videos
- KINEMATICSBook:ALLENChapter:KINEMATICSExercise:Integer Type Question
Explore 3 Videos
- MISCELLANEOUSBook:ALLENChapter:MISCELLANEOUSExercise:SUBJECTIVE QUESTION
Explore 9 Videos
Similar Questions
Knowledge Check
If a particle is moving along a circular path with constant speed, then Its motion is
APeriodic and simple harmonicBNon periodicCPeriodic but not simple harmonicDNon periodic but simple harmonicA particle is moving on a circular path with constant speed, then its acceleration will be
AZeroBExternal radial accelerationCInternal radial accelerationDConstant accelerationA particle is moving on a circular path with constant speed v. The magnitude of the change in its velocity after it has described an angle of 90∘ is :
AvB√2vC0D√3 v
A particle moves on circular path of radius 5 m with constant speed 5 m/s. Find the magnitude of its average acceleration when it completes half revolution.
View SolutionA particle is moving along a circular path with a constant speed 10ms−1.What is the magnitude of the change in velocity of the particle ,when it moves through an angle of 60∘ around the center of the circle ?
View SolutionA particle is moving on a circular path of radius 10m with uniform speed of 4ms−1.The magnitude of change in velocity of particle when it completes a semi circular path is
View SolutionFor a particle moving along a circular path with a constant speed, the accelerationis constant in
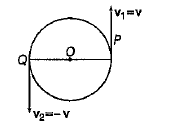
View SolutionA particle is moving with a constant speed in a circular orbit. Find the change in its velocity when it completes half the revolution.
View Solution